Stress concentrations

1
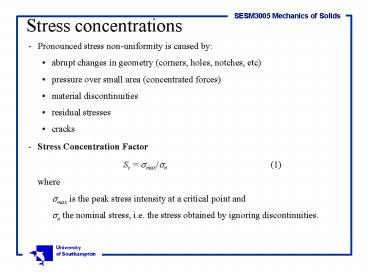
Stress concentrations

• Pronounced stress non-uniformity is caused by
• abrupt changes in geometry (corners, holes,
  notches, etc)
• pressure over small area (concentrated forces)
• material discontinuities
• residual stresses
• cracks
• Stress Concentration Factor
• Sc smax/sn (1)
• where
• smax is the peak stress intensity at a critical
  point and
• sn the nominal stress, i.e. the stress obtained
  by ignoring discontinuities.

2
Stress concentrations

• Methods for determining Sc
• Analytical, exact or approximate (numerical
  experimental), assuming elastic behaviour, giving
  the calculated Scc
• Experimental, accounting for material
  characteristics and the nature of loading
  (particularly fatigue), giving the effective Sce
• Example fatigue experiment with perfect and
  notched specimens

3
Stress concentrations

• Two-Dimensional Elasticity
• Theoretical predictions of stress concentration
  factors can be made by applying the
  two-dimensional theory of elasticity
• (i) Equations of equilibrium

Introducing Airy's stress function F, the
equations above are identically satisfied if the
stresses are given by
4
Stress concentrations - Two-Dimensional Elasticity

• (ii) Strain-stress relations
• In the case of thin solids (plates), it is
  assumed that sxz syz szz 0. This is the
  state of plane stress

In the case of thick or wide solids, it is
assumed that gxz gyz ezz 0. This is the
state of plane strain
5
Stress concentrations - Two-Dimensional Elasticity

• (iii) Compatibility Condition

In terms of Airys stress function, the above
condition is written
In polar/cylindrical co-ordinates
6
Stress concentrations

• Concentrated load on free edge of half-plane
• The stress function in this case is given by

The stresses are obtained in polar co-ordinates.
srr constant along circle with diameter d, srr ?
? as d ? 0
7
Stress concentrations

• Hole in infinite plate under uniaxial tension s
• Stresses relative to a polar frame of reference

From the above solution, Scc 3.
8
Stress concentrations

• Hole in a plate of finite width under uniaxial
  tension s

k is the ratio of strip width to hole diameter
sn is the average stress over the weakened
cross-section

9
Stress concentrations Elliptic hole

• Solution obtained using complex potentials and
  elliptic (orthogonal curvilinear) coordinates a
  and b defined by

Given a pair (a, b), the position of a point on
the x-y plane is specified as the intersection of
an ellipse
and a hyperbola
10
Stress concentrations Elliptic hole

• Constant c is chosen so that the ellipse for a
  particular value of co-ordinate a a0 coincides
  with a hole with major semi-axis a and minor
  semi-axis b.
• The hole is described by the equation

Constants a0 and c are given in terms of a and b
11
Stress concentrations Elliptic hole

• Along the hole boundary (a a0), saa 0,
• f angle between the major axis and the
  direction of loading
• Normal stress in the b (tangential) direction

12
Stress concentrations Elliptic hole

• Infinite plate in the direction of the minor axis
  (f p/2)

The maximum value of the right-hand side is
obtained for b 0 and b p
where r is the radius of curvature at the ends
of the major axis. As b/a ? 0, sbb ? ? but then
the hole becomes a crack and the problem is
considered in the context of Linear Elastic
Fracture Mechanics (LEFM)
13
Stress concentrations Elliptic hole
Shear in the directions of major and minor axes
Equivalent state of stress uniform tension s t
at f p/4 and uniform compression s t at f
p /4. Then
14
Stress concentrations Elliptic hole

• Any state of plane stress
• The principal stresses s1, s2 and their
  directions are determined.
• If f1 is the angle between the major axis and the
  first principal direction, sbb found for s1, f1
  and s2, f2 f1 p/2 are superposed.
• It is possible to find the maximum value of sbb
  and the angle b at which it occurs numerically.

15
Stress concentrations - cavities

• Cavity shown on the right prolate spheroid
  (rugby ball), formed by rotating ellipse about
  its major axis.
• Exact solutions obtained for an infinite solid
  (a, b ltlt overall dimensions)
• Scc given as a function of a/b
• For a sphere (a/b 1) Scc 2.05
• Similar data available for cavities in the shape
  of oblate spheroids, formed by rotating ellipse
  about its minor axis (door knob)

16
Flat bar with two grooves in tension

• Approximate formulae
• Shallow groove (t ltlt b)

• Deep groove t O(b)

• Groove of intermediate depth

17
Neubers diagram
18
Neubers diagram
19
Combined loading

• Stress concentrations may be the result of
  various loads acting together. If the stress they
  produce is of the same type, e.g. normal stress
  in the same direction, then the principle of
  superposition can be applied to evaluate the
  total maximum stress developing locally.
• Example circular shaft with an axisymmetric
  groove under both tension and bending (see Boresi
  Schmidt, p. 580). Both these loads cause
  nominal axial stresses sn(P) and sn(M),
  respectively. At the root of the groove, these
  stresses become Scc(P)sn(P) and Scc(M)sn(M),
  respectively. Applying superposition, the maximum
  stress at that point is found from
• smax Scc(P)sn(P) Scc(M)sn(M)

20
Combined loading

• If the shaft is also subjected to a torque T, the
  maximum shear due to local stress concentration
  is found from
• tmax Scc(T)tn(T)
• Yield failure is then assessed by introducing
  smax, tmax to either von Mises or Tresca yield
  criterion.