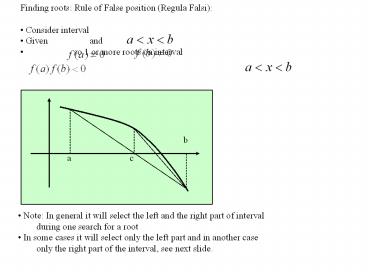
Finding roots: Rule of False position (Regula Falsi):

1

• Finding roots Rule of False position (Regula
  Falsi)
• Consider interval
• Given and
• so 1 or more roots on
  interval

b
a
c

• Note In general it will select the left and the
  right part of interval
• during one search for a root
• In some cases it will select only the left part
  and in another case
• only the right part of the interval, see next
  slide.

2
In interval point of inflection
b
1
a
2
Consider this when preparing the function
RegulaFalsi!
3
Algorithm
Rule of False position converges always,
sometimes slow.
4

• Finding roots Newton Raphson
• Select a point on the graph of f(x)
• Draw the tangent in that point
• Determine intersection of the tangent with
  x-axis
• Repeat procedure in the intersection until root
  is accurate

5
p1
p2
p0
Recursive formula
Problem?
6
Newton Raphson does not converge always, for
example
Start x0 gives
7
Fixed point iteration
Suppose you want a root of
So
Or
and
(equivalent form)
Fixed point iteration works as follows
x0 4 x1 3.31662 x2 3.10375 x3 3.01144 .
. . xn 3.0
Start value is
Compute successively
etc. Hopefully it will converge.
Stop when
8
In general, one may derive other equivalent
forms, e.g.
9

• Iterations
• Sometimes do not converge
• An iteration may be written as
• F does not need to be a mathematical function!
• Convergence requires for all
• This should hold for
• So if iteration does not converge try another
• start point.

10

• Advantages of iterations seen from Computer
  Science perspective
• Iterations are simple to program
• If iteration converges then arithmetic errors
  will be resolved
• in the next iteration
• Suppose you need to know the roots of
• Which method is better?
• quadratic-formula
• iterative method like Rule of False position

11
Stop conditions For example Newton iteration to
compute
e 0.01

• Criteria
• absolute
• relative
• combined

If a 1 then tolerance 1. If a 10 then
tolerance 0.1 For large values strict
Strict for small values, better suited for large
values
For small values of a ad is small absolute
criterion For large values of a e
negligible relative criterion
12

• Divide and conquer
• Suppose one needs to prepare a function to find
  the first 100
• prime numbers
• Prepare two functions
• one function that tests whether a number is
  prime
• another function that finds the first 100 prime
  numbers
• In this second function you call the first
  function.
• Separation of responsibilities and
• the function is clear and can be maintained more
  easily