ELECTROMAGNETIC DISTANCE MEASUREMENT EDM

1
ELECTROMAGNETIC DISTANCE MEASUREMENT (EDM)

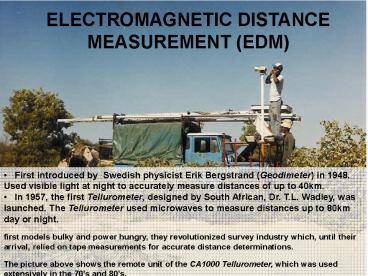
• First introduced by Swedish physicist Erik
  Bergstrand (Geodimeter) in 1948. Used visible
  light at night to accurately measure distances of
  up to 40km.
• In 1957, the first Tellurometer, designed by
  South African, Dr. T.L. Wadley, was launched. The
  Tellurometer used microwaves to measure distances
  up to 80km day or night.
• first models bulky and power hungry, they
  revolutionized survey industry which, until their
  arrival, relied on tape measurements for accurate
  distance determinations.
• The picture above shows the remote unit of the
  CA1000 Tellurometer, which was used extensively
  in the 70s and 80s.

2
INITIAL IMPACTS OF EDM
EDM Traverses (and Trilateration)
3
Propagation of Electromagnetic Energy
Velocity of EM energy V ? is the
frequency in hertz (cycles/second) ? is the
wavelength In vacuum the velocity of
electromagnetic waves equals the speed of
light. V c/n n gt1, n is the refractive
index of the medium
through which the wave
propagates c is the speed of light 299
792 458 m/sec f ? c/n or ? cf/n Note
that n in any homogeneous medium varies with the
wavelength ?. White light consists of a
combination of wavelengths and hence n for
visible light is referred to as a group index of
refraction. For EDM purposes the medium through
which electromagnetic energy is propagated is the
earths atmosphere along the line being measured.
It is therefore necessary to determine n of the
atmosphere at the time and location at which the
measurement is conducted.
4
Propagation of Electromagnetic Energy
The refractive index of air varies with air
density and is derived from measurements of air
temperature and atmospheric pressure at the time
and site of a distance measurement. For an
average wavelength ? na 1 ( ng-1
) x p - 5.5e x 10-8
1 0.003661T 760 1
0.003661T Where ng is the group index of
refraction in a standard atmosphere

(T0C, p760mm of mercury, 0.03
carbon dioxide) ng 1 ( 2876.04 48.864/?2
0.680/ ?4 ) x 10-7 p is the
atmospheric pressure in mm of mercury (torr)
T is the dry bulb temperature in C and
e is the vapor pressure Where e
ede and e4.58 x 10a, a(7.5T)/(237.3T),
de-(0.000660p
(10.000115T) (T-T) and
T is the wet-bulb temperature
So measuring p, T and T will allow for the
computation of n for a specific ?
5
THE FRACTION OF A WAVELENGTH AND THE PHASE ANGLE
?
90
r
?
½?
½?
0
Amplitude
180
- r
¼?
¼?
¼?
¼?
270
? ? 360
A fraction of a wavelength can be determined from
a corresponding phase angle ?
Note For ? 0 the fraction is 0 For ?
90 the fraction is ¼ For ? 180 the fraction
is ½ For ? 270 the fraction is ¾ For ? 360
the fraction is 1
EDM INSTRUMENTS CAN MEASURE PHASE ANGLES
6
Principles of Electronic Distance Measurement
If an object moves at a constant speed of V over
a straight distance L in a time interval ?t,
then L V?t (c/n)?t Knowing the speed
of light c and being able to determine the
refractive index, we could measure the time
interval it takes for an electromagnetic wave to
move from A to B to determine the distance L
between A and B. But since the speed of light (c)
is very high, the time interval ?t would need to
be measured extremely accurately. Instead, the
principle of EDM is based on the following
relationship L (m p) ?
m is an integer number of whole wavelengths, p is
a fraction of a wavelength
So L can be determined from ?, m and p
7
Solving for the integer number (m) of whole
wavelengths(Resolving the ambiguity in the
number of whole wave lengths)
p3
?2
p2
10
11
9
12
8
1
4
7
2
3
5
6
B
A
p1
?
?
?
?
?
?
?
?
?
?
?
?
L
Additional waves of known lengths ?3 k?2 and
?2 k ?1 (k is a constant), are introduced to
measure the same distance L L (m3 p3)
?3 L (m2 p2) ?2 L (m1 p1)
?1 Determining p1 p2 and p3 by measuring phase
angles ?1 ?2 and ?3 and solving the above
equations simultaneously yields L ( Note For L lt
?3 , m3 0).
8
USING DIFFERENT WAVELENGTHS
For example, if ?1 10.000 meters, k 10.000
and p1 0.2562, p2 0.2620 and p3 0.0125
(measured) Then ?2 10.000m x 10.000
100.000 and ?3 100.000 x 10.000
1000.000 L (m3 p3) ?3 (00.1250)x 1000.000
125.000m approximately m2 125/ ?2
125/100 1 and hence L (10.2620)x100.000
126.200m approximately m1 126.2/ ?1 126.2/10
12 and hence L (12 0.2562)x10 122.562m
mi whole wavelengths pi fractional parts of
a wavelength k constant
9
Basic Components of an EDM Instrument
Reflector
Reflector
L
Beam Splitter
Length of measured path is 2xL
Variable Filter
Interference Filter
Transmitter
L
F4
F1
Receiver Optics and phase-difference circuits
F2
F3
Measurement signal Reference signal
Frequency Generator
Phase Meter
Display
To obtain the phase angle the reflected signal
phase is compared to the reference signal phase.
Note also that the measured distance equals 2 x L.
10
General Remarks on EDM

• The original Tellurometer models, using
  microwaves, consisted of two units, the master
  and the remote, both of which required an
  operator
• The carrier wave was used to establish a voice
  channel between the operators in order to
  coordinate the manual switching of the
  frequencies.
• For long lines careful measurements of pressure
  and the wet- and dry-bulb temperatures were made
  at each end of the line.
• Measurements were very susceptible to multipath
  reflections (ground swing).

11

• Developments in electronics reduced the size of
  the components so EDMs could be mounted on
  theodolites to allow for simultaneous measurement
  of distances and directions
• Eventually EDMs were completely integrated into
  total stations
• Total stations allow for the direct input of
  temperature and pressure and automatic
  application of meteorological corrections
• Most of the current EDM instruments use LASER
  beams and passive optical reflectors, thus
  reducing the possibility of multipathing
• The latest models provide for reflector-less
  measurements, thus improving efficiency for
  certain applications

12
Sources of Error in EDM

• Instrumental
• Instrument not calibrated
• Electrical center
• Prism Constant (see next
• slide)

• Personal
• Careless centering of instrument and/or
  reflector
• Faulty temperature and pressure measurements
• Incorrect input of T and p

• Natural
• Varying met along line
• Turbulence in air

Remember L (m p) ?
13
Sources of Error in EDM
Determination of System Measuring Constant
C
A
B

• Blunders
• Incorrect met settings
• Incorrect scale settings
• Prism constants ignored
• Incorrect recording settings
• (e.g. horizontal vs. slope)

• Measure AB, BC and AC
• AC K (AB K) (BC K)
• K AC- (AB BC)
• If electrical center is calibrated, K rep-
• resents the prism constant.

Accuracy of EDM usually expressed as 5mm 10
ppm (constant scale error)
Good Practice Never mix prism types and brands
on same project!!! Calibrate regularly !!!