12.1 Solving Right Triangles

1
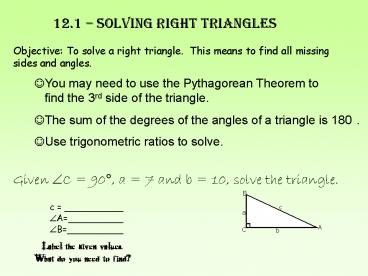
12.1 Solving Right Triangles
Objective To solve a right triangle. This means
to find all missing sides and angles.
?You may need to use the Pythagorean Theorem to
find the 3rd side of the triangle.
?The sum of the degrees of the angles of a
triangle is 180.
?Use trigonometric ratios to solve.
Given ?C 90?, a 7 and b 10, solve the
triangle.
c ?A ?B
Label the given values. What do you need to find?
2
Given ?A 20?, ?C90?, and c 15, solve the
triangle.
a b ?B
Label the given values. What do you need to find?
Homework worksheet 12.1
3
12.2 The Law of Sines
The trigonometric ratios work on right triangles.
However, if the triangle is not a right
triangle, we can use the Law of Sines or Law of
Cosines to solve the triangle.
For any triangle
For any triangle ABC with sides a, b and c
opposite ÐA, ÐB and ÐC respectively, if the
information given is in the form of ASA or AAS
then the Law of Sines can be used. Basically
you need an angle with the side opposite of the
angle given for this law to work.
4
Just like in 12.1, to solve a triangle means to
find the lengths of the missing sides and degree
of the missing angles.
Make sure your calculator is in degrees!!
Homework 12.2 worksheet
5
12.3 - The Ambiguous CaseObjective We will
extend the law of sines to solve triangles in
which we are given 2 sides and the angle opposite
one of the given sides. (SSA)
Since we are not guaranteed one and only one
triangle with SSA, we have the ambiguous case.
What are other options for the above triangle?
What if a 1?
6
To summarize When we have SSA then we have an
ambiguous case. This means that we can have 0,
1, or 2 triangles.
If ?A is acute and a lt b 0, 1, or 2
solutions possible If ?A is acute and a gt b
1 solution If ?A is obtuse/right a lt b
0 solutions If ?A is obtuse/right a gt b 1
solution
Assign Worksheet 12.3
7
12.4 Law of Cosines
Objectives Derive the Law of Cosines
To know when and how to use the Law of Cosines.
You use the Law of Sines when you have an angle
and the side opposite. So If you do not have
an angle and the side opposite, you need the Law
of Cosines.
But, from the right triangle ACD, we have
so
h
x
D
Now, since cosAx / b we have xbCosA or
8
Ex1) Given ?C 60, a 10 and b 14
?A ?B c
Once you use the Law of Cosines once, you will be
able to use the Law of Sines to finish the
problem.
9
Ex2) a 43, b 39, c
59
?A ?B ?C
Ex4) Two planes leave an airport at the same
time. Their speeds are 130 miles per hour and
150 miles per hour, and the angle between their
courses is 36 degrees. How far apart are they
after 1.5 hours?
Assign Worksheet 12.4 (Quiz after solving for
area using Trigonometry ?)
10
12-5 Area of a Triangle using Trigonometry
Objective To calculate the area of a triangle
using trigonometry.
You have learned how to find the missing parts of
a triangle using the trigonometric ratios, Law of
Sines and Law of Cosines. Since a triangle is
completely determined in these cases, its area is
also determined.
The area K of any triangle is given by one of the
following formulas. K ½ bc sin A K ½ ac
sin B K ½ ab sin C
Choose the formula depending upon what is given
in the problem. Use the angle and NOT the side
opposite of the angle.
11
Can you find the area using trig??? Assign
WS 12-5
12
Review of Solving Triangles

• Given a right triangle Use the trig ratios and
  Pythagorean Theorem.

• Given an angle and the side opposite Use Law
  of SinesBe aware that you may have the ambiguous
  case. (SSA)
• Ambiguous Case Be able to summarize all possible
  cases.

? If you do not have the angle and side opposite,
you will need to use the Law of Cosines.
13
Answers for the Review

• ? Z 80, y 5.4, z 6.1 7. ? X 50, y
  7.7, z 6
• 8. ? B 73.2, ? C 56.8, c 21.8 9. ? B
  24.2, ? C 56.8, c 21.8
• ? B 106.8, ? C 23.2, c 10.3
• Ø 11. ? A 16.9, ? C 127.5, c 16.4
• 12. ? A 90, ? B 60, c 5.2 13. Ø
• 14. ? A 46.6, ? B 57.9, ? C 75.5 15. ? A
  36.4, ? B 65.6, c 27.7
• 16. ? A 18.2, ? B 51.3, ? C 101.5 17. ?
  B 22.1, ? C 46.9, a 46.9
• Dont forget you can use the trig ratios if you
  have a right triangle. ?