Newtons Law of Viscosity

1
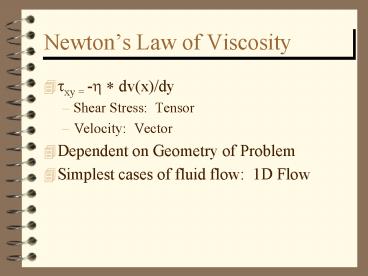
Newtons Law of Viscosity

• ?xy -????dv(x)/dy
• Shear Stress Tensor
• Velocity Vector
• Dependent on Geometry of Problem
• Simplest cases of fluid flow 1D Flow

2
Shear Stress Tensor
Txx Txy Txz Tyx Tyy
Tyz Tzx Tzy Tzz
?xy
Tii Are Normal Stresses in the Shear Stress Tensor
3
Typical Viscosities

• Metals 5x10-4 Pa-s to 1x10-2 Pa-s above melt
  temperature
• Ceramics Molten Viscosities, In the range of
  1x10-3 Pa-s to 10Pa-s again in relation to the
  melt temperature
• Polymers Molecular Weight Dependent, but 1 to
  1x105Pa-s above Tm or Tg
• Log ???? 3.4 log Mw N

4
Viscosity Relations

• ??Newtonian ?xy -??dvx/dy
• Bingham ?xy ?0 ??dvx/dy
• Power Law Fluids
• ?xy A??dvx/dy)n
• ngt1, shear thickening
• nlt1, shear thinning
• n 1, Newtonian

5
Flow Between Parallel Plates
y
2?
x
vx
x0
xL
Assumptions Incompressible Fluid Fully
Developed Flow Profile...Steady State Develop a
momentum balance within a small block of fluid
6
Momentum Balances

• Pressure momentum flux per square area
• Shear Stress Also a momentum flux per unit area
• Goal Do an equivalent sum of momentum flux as
  one would do a sum or forces around a free body
  diagram

7
Flow Between Parallel Plates

• Momentum Flux Across Surface y
• LW?yx evaluated at y y
• Momentum Flux Across Surface y ?y
• LW ?yx evaluated at y y ?y
• Momentum Flux Across Surface at x 0
• W ?yvx(?vx), evaluated at x 0
• Momentum Flux Across Surface at x L
• W ?yvx(?vx), evaluated at x L

8
Flow Between Parallel Plates

• Pressure Force on liquid at x 0
• W?yPx0 W?yP0
• Pressure Force on liquid at x L
• W?yPxL W?yPL

9
Momentum Flux Sum, Integration

• convective momentum (that carried through the
  cube) is the same given that the fluid velocity
  profile is the same...fully developed
• LW?yx at y y ?y - ?yx at y y W ?yPL -
  P0 0
• ?yx at y y ?y - ?yx at y y / ?y P0 -
  PL/L
• d ?yx/dy P0 - PL/L
• ?yx P0 - PLy/L C1
• B. C. 1 Shear Stress 0 at y 0, midpoint in
  fluid
• ?yx P0 - PLy/L

10
Applying Newtons Law

• ?xy -??dvx/dy P0 - PLy/L
• vx -P0 - PL/L ??y2/2 C2
• B. C. 2 No slip at wall... vx 0 at y ?
• C2 P0 - PL/L ?????2/2
• Plug back into equation and exactly solve for vx

11
Summary of Results

• Flow through // plates can be exactly solved.
• Assumes viscosity is uniform and known, other
  assumptions include no slip at the wall, an
  incompressible fluid and a fully developed flow
  profile.
• Other concerns could include friction which could
  alter m, and plate roughness.

12
How about a different geometry

• Flow down an incline plane
• Assumptions
• Fully Developed Flow
• Newtonian Fluid
• Shear is a function of normal distance from plane
• Gravity acting to aid flow
• Pressurenot a contributing since flow isnt
  pressurized

13
Flow Down the Incline Plane

• Momentum Balance
• Neglect convection
• Sforces 0
• tyx _at_yy - tyx_at_yyDy DZDx rgcos(q) DxDyDz
  0
• d tyx/dy rgcos(q)
• Boundary Conditions no slip at wall
• no shear stress at free surface,