2' Waves, the Wave Equation, and Phase Velocity

1
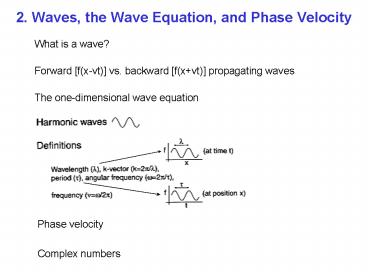
2. Waves, the Wave Equation, and Phase Velocity

• What is a wave?
• Forward f(x-vt) vs. backward f(xvt)
  propagating waves
• The one-dimensional wave equation

Phase velocity Complex numbers
2
What is a wave?

• A wave is anything that moves.
• To displace any function f(x)
• to the right, just change its
• argument from x to x-a,
• where a is a positive number.
• If we let a v t, where v is positive
• and t is time, then the displacement
• will increase with time.
• So f(x-vt) represents a rightward, or forward,
• propagating wave.
• Similarly, f(xvt) represents a leftward, or
  backward,
• propagating wave.
• v will be the velocity of the wave.

3
The one-dimensional wave equation
has the simple solution

• where f(u) can be any twice-differentiable
  function.

4
Proof that f(xvt) solves the wave equation

• 1. Write f(xvt) as f(u), where uxvt. So
  and
•  
• 2. Now, use the chain rule
•  
• 3. So Þ
  and Þ
•   
• 4. Substituting into the wave equation

QED
5
The 1D wave equation for light waves

• Well use cosine- and sine-wave solutions
•  
• or
• where

6
A simpler equation for a harmonic wave

• E(x,t) A cos(kx wt q)
• Use the trigonometric identity
• cos(uv) cos(u)cos(v) sin(u)sin(v)
• to obtain
• E(x,t) A cos(kx wt) cos(q) A sin(kx
  wt) sin(q)
• which is the same result as before, as long as
• A cos(q) B and
  A sin(q) C
• Life will get even simpler in a few minutes!

7
Definitions Amplitude and Absolute phase

• E(x,t) A cos(kx wt q)
• A Amplitude
• q Absolute phase (or initial phase)

8
Definitions

• Spatial quantities
  

Temporal quantities
9
The Phase Velocity

• How fast is the wave traveling? Velocity is a
  reference distance
• divided by a reference time.

The phase velocity is the wavelength /
period v l / t In terms of the
k-vector, k 2p/ l, and the angular frequency,
w 2p/ t, this is v w / k
10
The Phase of a Wave

• The phase is everything inside the cosine.
• E(t) A cos(j), where j
  kx wt q
• We give the phase a special name because it comes
  up so often.
• In terms of the phase,
• w j/t
• k j/x
• and
• j/t
• v
• j/x

11
Electromagnetism is linear The principle of
Superposition holds.

• If E1(x,t) and E2(x,t) are solutions to Maxwells
  equations,
• then E1(x,t) E2(x,t) is also a solution.
•  
• Proof and
•  
•  
• Typically, one sine wave plus another equals a
  sine wave.
•  
• This means that light beams can pass through each
  other.
•  
• It also means that waves can constructively or
  destructively interfere.

12
Complex numbers
Consider a point, P (x,y), on a 2D Cartesian
grid.
Let the x-coordinate be the real part and the
y-coordinate the imaginary part of a complex
number.

• So, instead of using an ordered pair, (x,y), we
  write
• P x i y
• A cos(j) i A sin(j)
• where i (-1)1/2

13
Euler's Formula

• exp(ij) cos(j)
  i sin(j)
• so the point, P A cos(j) i A sin(j), can be
• written
• P A exp(ij)
• where
• A Amplitude
• j Phase

14
Proof of Euler's Formula

• exp(ij) cos(j) i
  sin(j)
• Using Taylor Series

15
Complex number theorems
16
More complex number theorems

• 1. Any complex number, z, can be written
• z Re z i Im z
• So
• Re z 1/2 ( z z )
• and
• Im z 1/2i ( z z )
• where z is the complex conjugate of z ( i
  i )
• 2. The "magnitude," z, of a complex number is
• z2 z z
• 3. To convert z into polar form, A exp(ij)
• A2 Re z 2 Im z 2
• tan(j) Im z / Re z

17
We can also differentiate exp(ikx) as if the
argument were real.
18
Waves using complex numbers

• The electric field of a light wave can be
  written
• E(x,t) A cos(kx wt q)
• Since exp(ij) cos(j) i sin(j), E(x,t) can
  also be written
• E(x,t) Re A expi(kx wt q)
• or
• E(x,t) 1/2 A expi(kx wt q) c.c.
• where " c.c." means "plus the complex
• conjugate of everything before the plus sign."

19
The 3D wave equation for the electric field

• or
• which has the solution
•  
• where
• and

20
Waves using complex amplitudes

• We can let the amplitude be complex
• where we've separated out the constant stuff from
  the rapidly changing stuff.
• The resulting "complex amplitude" is
• So
• How do you know if E0 is real or complex?
• Sometimes people use the "", but not always.
• So always assume it's complex.