Appendix II: Introduction to Matrices

1
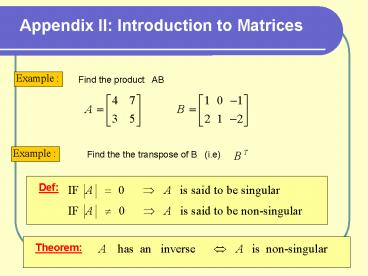
Appendix II Introduction to Matrices
Find the product AB
Find the the transpose of B (i.e)
Def
Theorem
2
Appendix II Introduction to Matrices
Find the augmented matrix
Def
A matrix A in row-echelon form if

1. The first nonzero entry in a nonzero row is 1
2. In consecutive nonzero rows the first entry 1 in
   the lower row appears to the right of the first
   1 in the higher row
3. Rows consisting of all 0s are at the bottom of
   the matrix

3
Appendix II Introduction to Matrices
Theorem
(by row operation)
Row Operation

1. Multiply a row by a nonzero constant
2. Interchange any two rows
3. Add a nonzero constant multiple of one row to any
   other

4
Appendix II Introduction to Matrices
Def
A matrix A in reduced-row-echelon form if

1. A is row-echelon form
2. A column containing a first entry 1 has 0s
   everywhere else

Theorem
(by row operation)
5
Solving Linear System
Gaussian Elimination Method
Solve
Gauss-Jordan Elimination Method
Row-echelon form
Reduced Row-echelon form
6
Using Row operation to find the inverse
Theorem
Special Case
7
Minors and Cofactor to find the inverse
Minors
Cofactor
8
Minors and Cofactor to find the inverse
Cofactor
Theorem II.2
9
Cofactor to find the determinant
Cofactor
Determinant
Determinant
Expand along row or column
10
The Eigenvalue Problem
Characteristic Equation
It is a polynomial of order n. ( A is nxn)
Eigenvalues of A are the roots of the
characteristic equation
Eigenvalues
11
The Eigenvalue Problem
Characteristic Equation
It is a polynomial of order n. ( A is nxn)
Eigenvalues of A are the roots of the
characteristic equation
Eigenvalues
Eigenvector