7'5 Complex Rational Expressions

1
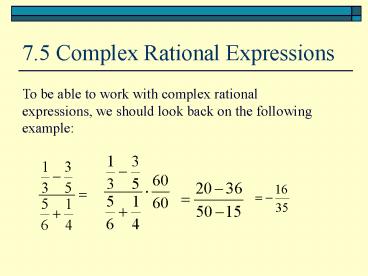
7.5 Complex Rational Expressions
To be able to work with complex rational
expressions, we should look back on the following
example
2
Complex Fractions

• Complex rational expressions, also called complex
  fractions, have numerators or denominators
  containing one or more rational expressions.
• Examples of complex fractions.

3
Simplifying a Complex Rational Expression by
Dividing

• If necessary, add or subtract to get a single
  rational expression in the numerator.
• If necessary, add or subtract to get a single
  rational expression in the denominator.
• Perform the division indicated by the main
  fraction bar Invert the denominator of the
  complex rational expression and multiply.
• If possible, simplify.

4
Simplifying a complex rational expression by
dividing.
The numerator is a single expression, so we do
nothing ?! Add to get a single expression in the
denominator. LCD is xy.
5
Simplifying a complex rational expression.
Invert and multiply. Simply.
6
Simplifying a Complex Rational Expression by
Multiplying by the LCD

• Find the LCD of all rational expressions within
  the complex rational expression.
• Multiply both the numerator and denominator of
  the complex fraction by the LCD.
• Use the distributive property and multiply each
  term in the numerator and denominator by the LCD.
  Simplify. No fractional expressions should
  remain in the numerator and denominator.
• If possible, factor and simplify.

7
Simplifying a complex rational expression by
multiplying.
Find the LCD of all rational expressions within
complex fraction. LCD 3xy
Multiply the numerator and denominator by the
LCD. Distribute the LCD.
Simplify.
8
7.5 Complex Rational Expressions
The notes for this section were based on the book
Blitzer, Introductory and Intermediate Algebra