DISTANCE MEASUREMENT

1
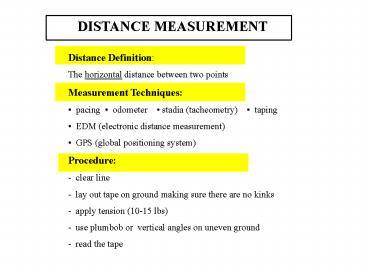
DISTANCE MEASUREMENT

• Distance Definition
• The horizontal distance between two points
• Measurement Techniques
• pacing odometer stadia (tacheometry)
  taping
• EDM (electronic distance measurement)
• GPS (global positioning system)
• Procedure
• - clear line
• - lay out tape on ground making sure there are
  no kinks
• - apply tension (10-15 lbs)
• - use plumbob or vertical angles on uneven
  ground
• - read the tape

2
TAPE READING
Normal Tape Shows cms and all divisions between
whole meters or feet. Adding Tape
0
1
1
2
67
68
Distance 68 0.60 68.60
Subtracting Tape
1
0
2
3
67
68
Distance 68 - 0.40 67.60
3
TAPING ON STEEP SLOPES
B
Breaking Tape
DISTANCE
A
Keep 100 ft/m (larger end) ahead in route surveys
when not using reel tape
4
COMMON TAPING ERRORS 1. Incorrect length of
tape 2. Temperature variation 3. Non-standard
tension 4. Sag (catenary) 5. Poor alignment 6.
Slope TAPE STANDARDIZATION Done by comparison
with standard tape or known baseline. Standardizat
ion usually defined in terms of - tension
(e.g. 12 lbs) - temperature (e.g. 68F)
5
1. CORRECTION FOR INCORRECT LENGTH cl ((l -
l)/l) L L L cl Where l calibrated
or actual length l nominal length (e.g.
100ft/m) L measured distance L corrected
distance Example A 100ft steel tape when
calibrated measures 100.02ft. What is the
corrected distance of a line that measures
565.75? cl ((100.02 - 100.00) / 100.00)
565.75 0.11315 ft So L 565.75 0.11
565.86
6
2. TEMPERATURE CORRECTION ct k (T1 - T)
L L L ct Where ct temperature
correction k coefficient of
expansion/contraction of steel 0.000 0065
per unit length per F 0.000 0116 per unit
length per C T1 tape temp. at time of
measurement T standard temperature L
measured distance L corrected
distance Example The recorded length of a line
measured at a temp of 30.5 F with a steel tape
that is 100 ft long at 68 F was 872.54 ft. What
is the corrected distance? ct 0.000 0065
(30.5 - 68)872.54 -0.213 So L 872.54
- 0.213 872.33
7
3. TENSION (PULL) CORRECTION cP (P1 - P)
L/ AE L L cp Where P standard tension
for tape P1 tension applied to tape L
measured distance L corrected distance A
cross-sectional area of tape E modulus of
elasticity of steel in lbs/in2 (29,000,000) Examp
le A steel tape that is 100 ft long under a pull
of 12.0 lbs when supported, and has cross
sectional area of 0.005 in2 is used to measure a
distance of 686.79 with a tension of 20 lbs.
What is the corrected distance? cp (20 - 12)
686.79/(0.005 29,000,000) 0.038 So
L 686.79 0.038 686.83
8
4. SAG OR CATENARY CORRECTION cs (- w2 Ls3)
/ (24 P12) - (W2 Ls) / (24 P12) L L
cs Where w unit weight of tape
(lbs/ft) Ls unsupported length measured P1
pull/tension (actual) L corrected
distance Example A steel tape 100 ft long weighs
1.50 lbs (0.015 lb/ft) and is supported at the
ends only. A line is measured in 3 segments of
100.00, 100.00 and 50.52 at a tension of 12 lbs
and recorded as 250.52ft. What is the corrected
distance? Segment 1 cs - (1.50)2 100.00 /
24 (12)2 -0.065 Segment 2 cs
-0.065ft Segment 3 cs - (0.015)2 (50.52)3 /
24 (12)2 -0.008 So L 250.52 - 2
(0.065) - 0.008 250.38
9
5. MISALIGNMENT OF TAPE (should be avoided)
d
If d 1.0 ft, then error of 0.02 ft is introduced
6. CORRECTION FOR SLOPE
B
L
a
H
A
H L Cos a a vertical angle
10
LAYOUT When laying out (setting out) a line
corrections are applied in reverse. If a line
(L) of exactly 10.00 ft, for example, is to be
set out and the taping corrections amount to
0.039 ft L L Corrn. So L L
- Corrn. L 10.000 0.039 9.961 ft
(measured on ground)