Second Order Partial Derivatives

1
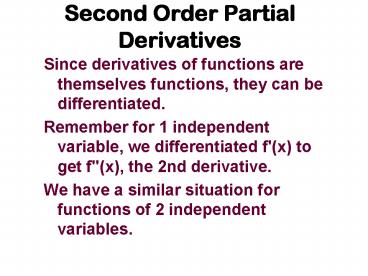
Second Order Partial Derivatives

• Since derivatives of functions are themselves
  functions, they can be differentiated.
• Remember for 1 independent variable, we
  differentiated f'(x) to get f"(x), the 2nd
  derivative.
• We have a similar situation for functions of 2
  independent variables.

2
Second Order Partial Derivatives

• We have a similar situation for functions of 2
  independent variables.
• You have seen that the partial derivatives of
  functions are also functions. So we
  differentiate them. However, since the 1st
  partial derivative can be a function of both
  independent variables, we have more possible 2nd
  derivatives.

3
Second Order Partial Derivatives

• If z f(x,y), then we find
• How many ways can we differentiate fx??

4
Second Order Partial Derivatives

• If z f(x,y), then we find
• How many ways can we differentiate fx??
• Since fx is a function of x and y, we may find
  partial derivatives with respect to both x and y!

5
Second Order Partial Derivatives

• If z f(x,y), then we find
• Since fx is a function of x and y, we may find
  partial derivatives with respect to both x and y!

6
Second Order Partial Derivatives

• If z f(x,y), then we find
• Since fy is a function of x and y, we may find
  partial derivatives with respect to both x and y!

7
Second Order Partial Derivatives

• Remember 2nd derivatives from Calculus 1?
• What did they tell us about a function of 1
  independent variable??

8
Second Order Partial Derivatives

• The 2nd derivative tells us about the curvature
  of the f(x). If f"(x)gt0 near a point, what do we
  know??
• If f"(x)gt0 in an interval around x, the function
  is concave up on that interval.
• If f"(x)lt0 in an interval around x, the function
  is concave down on that interval.

9
Second Order Partial Derivatives

• If f"(x)gt0 in an interval around x, the function
  is concave up on that interval.
• If f"(x)lt0 in an interval around x, the function
  is concave down on that interval.
• Can you carry these ideas over for fxx and fyy?

10
Second Order Partial Derivatives

• fxx determines the curvature of f(x,y) in a
  constant y plane.
• The figure shows tangent lines with slope fx on
  the surface of f(x,y) on the plane yb.

11
Second Order Partial Derivatives

• fyy determines the curvature of f(x,y) in a
  constant x plane.
• The figure shows tangent lines with slope fy on
  the surface of f(x,y) on the plane xa.

z
y
(a,b,0)
x
12
Second Order Partial Derivatives

• What about the mixed derivatives, fxy and fyx?
• The figure shows tangent lines with slope fx
  varying with y.
• fxy tells us how the slope, fx, varies with y.

z
(a,b,0)
y
x
13
Second Order Partial Derivatives

• What about the mixed derivatives, fxy and fyx?
• The figure shows tangent lines with slope fy
  varying with x.
• fyx tells us how the slope, fy, varies with x.

z
y
(a,b,0)
x
14
Second Order Partial Derivatives

• Examples Find all 2nd partial derivatives
• a)
• b)
• c)

15
Local Extrema

• Remember again from Calculus 1 and 2 for
  functions of 1 independent variable how you found
  local extrema.
• What is the difference between global and local
  extrema?
• What were the tests you can use to determine
  local extrema?

16
Local Extrema

• Recall
• f has a local maximum at the point Po, if
  f(Po)?f(P) for all point P near Po.
• f has a local minimum at the point Po, if
  f(Po)?f(P) for all point P near Po.
• What do we mean by critical points of a function
  of 1 variable, f(x)?
• How do we find critical points for f(x)?

17
Local Extrema

• Recall
• f has a local maximum at the point Po, if
  f(Po)?f(P) for all point P near Po.
• f has a local minimum at the point Po, if
  f(Po)?f(P) for all point P near Po.
• How will these ideas translate for f(x,y)??

18
Local Extrema

• Recall
• f has a local maximum at the point Po, if
  f(Po)?f(P) for all point P near Po.
• f has a local minimum at the point Po, if
  f(Po)?f(P) for all point P near Po.
• The gradient of f serves the same function for
  f(x,y) as f'(x) did for x.
• Recall that grad f points in the direction of
  greatest increase of f. What does this mean if
  Po is a local max?

19
Local Extrema

• Examine Fig 14.1 and 14.2 pg 176.
• You can see the local maxima and minima in Fig.
  14.1. How do they appear in the contour diagram
  in Fig 14.2??
• Can you see as you get closer and closer to a
  local extrema, the contour lines get closer and
  closer together until what happens?

20
Local Extrema

• The condition for finding the critical points is
  for
• For grad f to be zero, what must be true?

21
Local Extrema

• The condition for finding the critical points is
  for
• For grad f to be zero, what must be true?
• This means that each partial derivative must be
  zero.
• Example 1,2 pg 177-178. Note how the local
  min/max are found.

22
Local Extrema

• The condition for finding the critical points is
  for
• Find the critical points of the function