Estimating a Population Mean Using a Confidence Interval - PowerPoint PPT Presentation
1 / 25
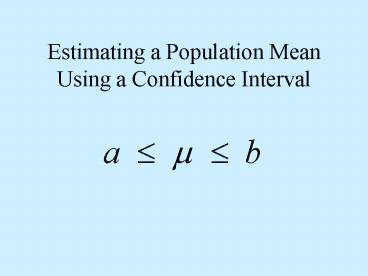
Title:
Estimating a Population Mean Using a Confidence Interval
Description:
Z. Figure. z. 0 -z .90 .4500 .4500. M155 L28: Estimating with a Confidence Interval -- Slide 8 ... confidence, draw a normal histogram for with an interval ... – PowerPoint PPT presentation
Number of Views:98
Avg rating:3.0/5.0
Title: Estimating a Population Mean Using a Confidence Interval
1
Estimating a Population MeanUsing a Confidence
Interval
2
Figure 4
CONFIDENCE
_
CONFIDENCE 2
CONFIDENCE 2
X
?
MARGIN OF ERROR
Z
z
-z
0
3
Figure 4
CONFIDENCE
_
CONFIDENCE 2
CONFIDENCE 2
X
?
Starting with sample mean, must subtract and add
margin of error
Z
z
-z
0
4
The margin of error is the sample half-width of
the confidence interval, measured on the scale
is given by the formula You can write
numbers on the z-scale, but don't write any
numbers on the -scale just the letter ?.
Sometimes students find numbers in the problem to
put in these places, but the numbers can't be
right, because ? is unknown.
M155 L28 Estimating ? with a Confidence Interval
-- Slide 4
5
EXAMPLE K A large group of bolts has just been
received, but before allowing them to be used in
manufacturing, the Quality Assurance Department
wants to estimate their average weight. These
bolts will be part of a large aircraft, and
multiplying the average weight by the number of
bolts used in a wing gives the total weight of
bolts in that wing. Suppose a random sample
of 36 bolts from the shipment has an average
weight of .155 ounces and a standard deviation of
.018 ounces. Estimate the average weight of all
the bolts in the shipment with 90 confidence.
Then revise the estimate using 95 confidence.
Finally, estimate the average weight of the
shipment with 99 confidence.
M155 L28 Estimating ? with a Confidence Interval
-- Slide 5
6
SOLUTION K Notice first that the confidence
level to be used was given in the problem,
following the word confidence. (We actually have
three problems for different confidence
levels.) Here is a summary of what we
know. POPULATION SAMPLE !! n 36
(large) ? unknown .155 (data ) ? ? s
(since n large) s .018 Since the sample
is large (n?30), we know that the sample mean
statistic is nearly normal, the sample
standard deviation is close to the population
standard deviation, and therefore
M155 L28 Estimating ? with a Confidence Interval
-- Slide 6
7
Figure
.90
.4500
.4500
_
X
?
Z
z
-z
0
8
For 90 confidence, draw a normal histogram for
with an interval centered on the unknown mean ?
and containing probability .90, so that half that
probability (.90/2 .45) falls on each side of
?.
M155 L28 Estimating ? with a Confidence Interval
-- Slide 8
9
Figure 5
.90
.4500
.4500
_
X
?
Z
z1.645
0
-1.645
10
M155 L28 Estimating ? with a Confidence Interval
-- Slide 10
11
For 95 confidence, draw a normal histogram for
with an interval centered on the unknown mean ?
and containing probability .95, so half that
probability (.95/2 .475) falls on each side of
?. Since the desired probability .4750 appears
exactly in the body of the table (for z1.96) we
use z1.96
M155 L28 Estimating ? with a Confidence Interval
-- Slide 11
12
Figure 6
.95
.4750
.4750
_
X
?
Z
z1.96
0
-1.96
13
M155 L28 Estimating ? with a Confidence Interval
-- Slide 13
14
For 99 confidence, draw a normal histogram for
with an interval centered on the unknown mean ?
and containing probability .99, so half that
probability (.99/2 .495) falls on each side of
?. Now we seek a Z-score corresponding to a
probability .4950 in the body (inside) of the
normal table.
M155 L28 Estimating ? with a Confidence Interval
-- Slide 14
15
Figure 7
.99
.4950
.4950
_
X
?
Z
z2.575
0
-2.575
16
M155 L28 Estimating ? with a Confidence Interval
-- Slide 16
17
EXAMPLE L Suppose subjects in the DATA\grades
data set were chosen randomly from all freshmen
and sophomores at the university. Estimate the
mean Verbal SAT score for all freshmen and
sophomores with 95 confidence. SOLUTION
L For MINITAB instructions, refer to Chapter
One 5.2 . The data set has 200 subjects, so we
can use the Z statistic but MINITAB expects us
to provide an estimate of the population standard
deviation ?. Since the sample is large, we will
estimate ? with the sample standard deviation
s. In MINITAB open the L\Mtbwin\DATA\grades
worksheet, choose Stat gt Basic Statistics gt
Display Descriptive Statistics, and double-click
on the variable Verbal, (or all three variables
Verbal, Math, GPA). Click on OK. Write down the
(sample) standard deviation s73.21 that
appears in the Verbal row.
M155 L28 Estimating ? with a Confidence Interval
-- Slide 17
18
Now choose Stat gt Basic Statistics gt 1-Sample Z,
double-click on the variable Verbal, enter 73.21
for Sigma, and enter anything for the Test mean.
Choose Options, enter a confidence level of 95.0
(percent), and select the alternative hypothesis
not equal. When you click on OK, MINITAB will
return both endpoints of a 95 symmetric
confidence interval. (We are not necessarily
interested in whatever two-sided test is
performed.) Test of mu 600 vs mu not
600 The assumed sigma 73.21 Variable
N Mean StDev SE Mean Verbal
200 595.65 73.21 5.18 Variable
95.0 CI Z P Verbal
( 585.50, 605.80) -0.84 0.401 CONCLUSION
L This means we are 95 confident that 585.50 ?
? ? 605.80 that the mean Verbal SAT is at east
585.5 points and at most 605.8 points.
M155 L28 Estimating ? with a Confidence Interval
-- Slide 18
19
EXAMPLE M Suppose subjects in the DATA\grades
data set were chosen randomly from all freshmen
and sophomores at the university. Estimate the
mean Verbal SAT score for all freshmen and
sophomores with 90 confidence. SOLUTION
M Now choose Stat gt Basic Statistics gt 1-Sample
Z, double-click on the variable Verbal, enter
73.21 for Sigma, and enter anything for the Test
mean. Choose Options, enter a confidence level
of 90.0 (percent), and select the alternative
hypothesis not equal. When you click on OK,
MINITAB will return both endpoints of a 90
symmetric confidence interval. Test of mu
600 vs mu not 600 The assumed sigma
73.21 Variable N Mean StDev
SE Mean Verbal 200 595.65 73.21
5.18 Variable 90.0 CI
Z P Verbal ( 587.14, 604.16)
-0.84 0.401 CONCLUSION M This means we are
90 confident that 587.14 ? ? ? 604.16 that
the mean Verbal SAT is at east 587.14 points and
at most 604.16 points.
M155 L28 Estimating ? with a Confidence Interval
-- Slide 19
20
(No Transcript)
21
(No Transcript)
22
(No Transcript)
23
(No Transcript)
24
(No Transcript)
25
(No Transcript)