Repeated Measure

1
Repeated Measure
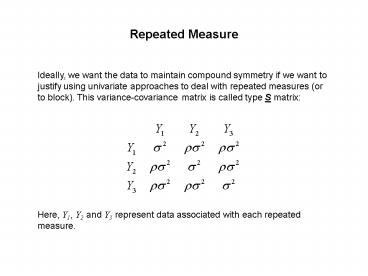
Ideally, we want the data to maintain compound
symmetry if we want to justify using univariate
approaches to deal with repeated measures (or to
block). This variance-covariance matrix is called
type S matrix
Here, Y1, Y2 and Y3 represent data associated
with each repeated measure.
2
Repeated Measure
Though, important it is but a subset of a more
general condition to be able to use the regular
set up of blocking and repeated measure in a
univeratiate structure. The more general
condition is sphericity (Huynh-Feldt condition)
that states that the variance of the difference
of any two repeated measurements is constant
This results in a variance covariance matrix
called the H matrix. Where, Yi, and Yj
represent data associated with some repeated
measurements.
3
Repeated Measure
If sphericity holds then we can use the
univariate approach and deal with the data as if
the repeated measurements are blocks as we stated
before (blocks need to conform to this condition
as well).
How do we know? We can test for it using a test
by Mauchly (1940) that is implemented in SAS.
This test is distributed approximately Chi2 with
degrees of freedom (p(p-1)/2)-1), p is the
number of repeated measures.
4
Repeated Measure
Example 1 Rabbits are tested for there response
to a certain drug with two control factor levels.
The drug is Amidaron (a2) and the other two
factor levels are vehicle solution (a1) and
saline solution (a0), both harmless. Temperature
of 15 rabbits that are randomly assigned to these
different levels, with a condition that five
rabbits are assigned to each levelis taken every
30 minutes at time periods (0, 30, 60, and 90),
representing repeated measures levels b0, b1, b2,
and b3.
5
Repeated Measure
Disclaimer The above mentioned test is low
power i.e. its ability to reject the null when
the alternative is true is quite small. Hence,
according to Kuehl (1994) failing to reject the
null doesnt give much indication to the
appropriateness of using a univariate approach
unless the sample size is large. Otherwise, the
researchers needs to use her/his experience to
evaluate the univaraite analysis appropriateness,
or just go multivariate.
6
Repeated Measure

• What if Sphericity doesnt hold, three choices
• Univariate still
• Adjust the F test statistic to compensate for
  correlated measurements.
• Construct contrasts on the repeated measurements.
• Multivariate
• Multivariate Analysis.

7
Repeated Measure
Just a note The Multivariate Analysis of
Variance is NOT quite the same as the analysis of
Repeated measures (even though we can use
Multivariate setup to analyze repeated
measure). If the measurements taken on the same
individual represent qualitatively different
variables, such as weight, height, and shoe size,
for the same experimental unit then these are
considered multivariate measures.
8
Repeated Measure
Just a note (Cont.) If, on the other hand, the
measurements can be thought of as being
measurements under different levels of a factor,
such as response to medicine 1, 2 and 3 or
measurements that are time dependentthen these
are considered repeated measurements. In
repeated measurements we are interested in
comparing the different repeated measurements to
one another as well as comparing the main effect
of the other factor, in multivariate we are not.
9
Repeated Measure

• Adjust the F test statistic to compensate for
  correlated measurements.

Two available adjustments both are based on a
proposed adjustment, e freedom by Box (1954). Both of which estimate
the adjusting factor Greenhouse and Geisser
(1959) use a maximum likelihood approach that
tends to underestimate this factor according to
Huyhn and Feldt (1976) who provide another
estimate for the same factor. SAS calculates
both for you!
10
Repeated Measure

• Construct contrasts on the repeated measurements.

Use contrasts on the repeated measurements to
reduce these measurements to one number, and then
use that number in a regular analysis of variance
to study the effect of the factor.
11
Repeated Measure

• Construct contrasts on the repeated measurements
  (Cont.).

There are multiple types of contrasts that can be
used that can capture the trend in the repeated
measurements. These contrasts can actually be
used to capture any trend in the means
understudy. These contrasts capture the linear,
quadratic, cubic .
12
Repeated Measure

• Multivariate Analysis.

The above approaches are a bit within the realm
of this class, another approach is to use a
multivariate data analysis to analyze the data.
This assumes that the measurements are correlated
(which they are). SAS provide the REPEATED
option that gives one the ability to analyze the
data and compare the repeated measurements as
well as the main effects, which exactly what we
want.
13
Repeated Measure

• Multivariate Analysis (Cont.).

Multivariate data analysis introduces tests that
are similar to the F test statistic that we all
know and love. For test statistics are compared
usually Wilks Lambda Pillias
Trace Hotelling_Lawley Trace and Roys Greatest
Root
14
Repeated Measure

• Multivariate Analysis (Cont.).

These are beyond the scope of this class Though
Wilks Lambda comes highly recommended by Johnson
(1998) because it is based on a likelihood
testing approach with good qualities.
15
Repeated Measure

• Multivariate Analysis (Cont.).

What does it tell you when these tests are
significant? That at least one of the means at
one of the levels of each of these repeated
measures is different. Remember that you are
dealing with a vector of means right now. You
will need to do further analysis to see which
means really differ. In a way it is like the F
test of the equality hypotheses that we have been
doing, before going further into analyzing the
data. This involves univariate ANOVAs on the
separate variables, among other kinds of analyses.