Univariate Statistics of Dispersion

1
Univariate Statistics of Dispersion
p 47

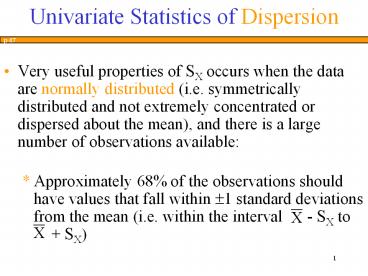
• Very useful properties of SX occurs when the data
  are normally distributed (i.e. symmetrically
  distributed and not extremely concentrated or
  dispersed about the mean), and there is a large
  number of observations available
• Approximately 68 of the observations should have
  values that fall within ?1 standard deviations
  from the mean (i.e. within the interval - SX
  to ( SX)

2
Univariate Statistics of Dispersion
p 47

• Approximately 95 of the observations should have
  values that fall within ?2 standard deviations
  from the mean (i.e. within the interval - 2SX
  to ( 2SX)

3
Univariate Statistics of Dispersion
p 47

• The variance (S2X) is the square of the standard
  deviation
• 
  (3.7)

4
Univariate Statistics of Dispersion
p 47

• It provides the same information about the
  variable of interest contained in the standard
  deviation, but it is often used as the main
  measure of dispersion in statistics
• The numerator in the variance is
  considered a measure of the total variation in

5
Linear Transformations

• In applied statistics, sometimes is convenient to
  define and create a new variable as a
  transformation of an existing one, i.e.
• Yi f(Xi) for all i

6
Linear Transformations

• If we know and SX, and the transformation is
  linear, there is a simple way to calculate and
  SY directly from and SX for instance if
• Yi a bXi for all i, then
• a b

7
Linear Transformations

• In addition
• S2Y b2S2X and SY bSX

8
Bivariate Statistics
p 53

• The ultimate objective of regression analysis is
  to determine if and how certain (independent)
  variables influence another (dependent) variable
• Bivariate statistics can be used to examine the
  degree in which two variables are related,
  without implying that one causes the other

9
Bivariate Statistics
p 54

• In Figure 3.3 (a) Y and X are positively but
  weakly correlated while in 3.3 (b) they are
  negatively and strongly correlated

10
Bivariate Statistics Covariance
p 53

• The covariance is one measure of how closely the
  values taken by two variables Y and X vary
  together
• 
  (3.17)
• A disadvantage of the covariance statistic is
  that its magnitude can not be easily interpreted,
  since it depends on the units in which we measure
  Y and X

11
Bivariate Statistics Correlation Coefficient
p 54

• The related and more used correlation coefficient
  remedies this disadvantage by standardizing the
  deviations from the mean
• 
  (3.18)