The Additive Model of Stock Price Changes

1
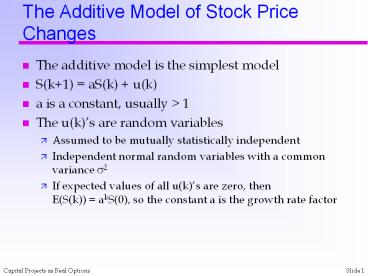
The Additive Model of Stock Price Changes

• The additive model is the simplest model
• S(k1) aS(k) u(k)
• a is a constant, usually gt 1
• The u(k)s are random variables
• Assumed to be mutually statistically independent
• Independent normal random variables with a common
  variance ?2
• If expected values of all u(k)s are zero, then
  E(S(k)) akS(0), so the constant a
  is the growth rate factor

2
Criticisms of the Additive Model

• Since the random term is a normally distributed
  random variable, the prices could go negative
• The standard deviation should be proportional to
  the price

3
The Multiplicative Model

• The multiplicative model has the form
• S(k1) u(k)S(k)
• u(k) is the relative (or percentage) change in
  S(k)
• This relative change is S(k1)/S(k), and
  independent of units (eg dollars, yen, etc.)
• Taking the natural logarithms, we have the
  additive model
• ln S(k1) ln S(k) ln u(k)
• define w(k) ln u(k)
• w(k)s are normally distributed with expected
  value ? and variance ?2

4
Lognormal prices

• Note that u(k) ew(k)
• The u(k)s are lognormal random variables
• Since ln S(k) ln S(0)
• ln S(k) is lognormal also
• Eln S(k) ln S(0) ?k
• Varln S(k) k?2
• Note Empirical evidence supports this view

5
Estimating ? and ?

• The value of w(k) ln u(k) is the logarithm of
  the return on the stock, with a mean value ? and
  variance ?2
• Typically, these parameters are estimated for a
  year. If the time period is a percentage of year
  p, then

6
The Mean

• Suppose that w has expected value and variance
  ?2
• Then the mean of the expected rate of increase is
  given by
• Note that there is a correction factor related
  to the variance of the distribution.
• As the variance increases, the lognormal
  distribution spreads out, but cannot go below
  zero, so the mean increases as a function of the
  variance

7
Random Walks

• We define the additive process z by
• This process is a random walk, and
• is a normal random variable with mean 0 and
  variance equal to 1

8
Wiener Process

• A Wiener process is obtained by taking the limit
  of a random walk as ?t goes to 0
• In symbolic form, we have
• A process z(t) is a Wiener process if
• For any sltt the quantity z(t) z(s) is a normal
  random variable with mean zero and variance t-s
• For any 0ltt1ltt2ltt3ltt4, the random variables z(t2)
  z(t1) and z(t4) z(t3) are uncorrelated
• z(t0) 0 with probability 1

9
Wiener Process (Continued)

• Intuitively, a continuous time version of a
  random walk
• A generalized Wiener process has the form
• where x(t) is a random variable for each t, z is
  a Wiener process, and a and b are constants
• An Ito process is written
• where z is a Wiener process

10
Stock prices and Wiener processes

• The multiplicative model of stock prices
  ln S(k1) ln S(k) w(k) may be
  written in continuous time as d ln S(t) ?dt
  ?dz where z is a standard Wiener process
• ?dt may be interpreted as the mean value of the
  right hand side, and is proportional to dt
• The standard deviation of the right hand side is
  ? times the standard deviation of dz, which is of
  the order of magnitude of ??dt
• The continuous time model is a generalized Wiener
  process with solution ln S(t) ln S(0) ?t
  ?z(t), so Eln S(t) Eln S(0) ?t

11
Lognormal prices

• The continuous time solution is termed geometric
  Brownian motion, and is a lognormal process
• The mean value must be adjusted, and is
• If we define ? ? .5?2
• The standard deviation is given by

12
The Ito Process for Prices

• We can express the random process for prices in
  terms of S(t) rather than in terms of its ln
• This requires a correction using Itos lemma
• Using ? ? .5?2 we obtain the standard Ito
  form for price dynamics

13
Summary of relations for Brownian motion

• Suppose the geometric Brownian process S(t) is
  governed by
• where z is a standard Wiener process. Let ? ?
  .5?2. Then S(t) is lognormal and

14
These results lead to these binomial lattice
approximations
15
A Review of Financial Options

• Options Special contractual arrangements giving
  the owner the right to buy or sell an asset at a
  fixed price anytime on or before a given date.
• Types of Options
• Stock options - traded on organized exchanges
  since 1973.
• Currency, commodity and interest rate options.
• Corporate securities - bonds, warrants and other
  convertibles.
• Capital structure decisions - lender acquires
  company and shareholders obtain option to buy it
  back by paying off debt.
• Capital-budgeting decisions - oil and mineral
  leases.

16
Characteristics of Options

• Not bought for the usual benefits offered by
  other securities, i.e. interest and dividends.
• Alternative to investing in a security.
• Options' expected return is greater than the
  underlying security (and so is its risk).
• Motivations for participating in option market
• Speculate in an attempt to grab a quick buck.
• Earn extra income on a security you already own.
• Hedging mechanism for existing position.

17
What is an Option?

• Listed options are contracts that give the holder
  the right (but not the obligation) to buy or sell
  a pre-specified security at a pre-specified price
  by a pre-specified date.
• Call options give the owner the right to buy 100
  shares of a specific stock at a specific price.
• Put options give the owner the right to sell 100
  shares of a specific stock at a specific price.
• Calls and Puts are very distinct investments.
• Calls are an expression of the buyer's optimism -
  you buy calls if you expect stock prices to rise.
• Puts are bearish investments - you expect stock
  prices to fall.

18
Option Terminology

• Option Buyer (Holder or Owner) - the individual
  who obtains the right to exercise.
• Option Seller (Writer) - the individual who is
  obligated, if and when he or she is assigned an
  exercise notice, to perform according to the
  terms of the option contract.
• Exercise (Strike) Price - For a call, the price
  per share at which the holder can purchase the
  underlying stock from the option writer for a
  put, the price at which the holder can sell the
  underlying stock to the option writer.
• Expiration Date -stock options expire on the
  Saturday following the third Friday of the
  expiration month expirations are based on a
  3-month calendar cycle.
• Premium - price paid by the buyer to the writer
  of an option set in the marketplace by
  investors' demand and supply.

19
Option Terminology (contd)

• American and European Options - An American
  option may be exercised anytime up to the
  expiration date a European option differs in
  that it can be exercised only on the expiration
  date.
• "In-the-Money" Option - A call option is
  "in-the-money" whenever its exercise price is
  below the current stock price. Conversely, a put
  option is "in-the-money" whenever its exercise
  price is above the current stock price. Such
  options are said to have intrinsic value.
• "Out-of-the-Money" Option - A call option is
  "out-of-the-money" whenever its exercise price is
  above the current stock price. Conversely, a put
  option is "out-of-the-money" whenever its
  exercise price is below the current stock price.
  Such options are said to have no intrinsic value.

20
Call Option - An Example
Suppose Mr. Optimist holds a six month call
option for 100 shares of Exxon common stock. It
is a European call option and can be exercised at
150 per share. Now assume that the expiration
date has arrived. What is the value of the Exxon
call option on the expiration date? Answer If
Exxon is selling for 200 share, Mr. Optimist can
exercise the option - purchasing 100 shares of
Exxon at 150 per share - and then immediately
sell the shares at 200. Mr. Optimist will have
made 5,000 (100 shares x 50). Let St be the
stock price on the exercise date, then Payoff
on Expiration Date If St ? 150, then call
option value 0 If St gt 150, then call
option value St - 150 Conversely, assume
that Exxon is selling for 100 per share on the
expiration date, If Mr. Optimist still holds the
call option, he will throw it out.
21
A Graphical View of a Call Option
Assumes options are in lots of 100
22
Option Writer - Naked Option . . .
23
. . . and a covered call option
24
Put Option - An Example
Suppose Ms. Pessimist feels quite certain that
Exxon will fall from its current 160 share
price. She buys a put. Her put contract gives her
the right to sell 100 shares of Exxon at 150 six
months from now. Now assume that the expiration
date has arrived. What is the value of the Exxon
put option on the expiration date? Answer If
Exxon is selling for 200 share, Ms. Pessimist
will tear up the put option and throw it away -
it is worthless. On the other hand, if Exxon is
selling for 100 per share, Ms. Pessimist can
purchase 100 shares of Exxon at 100 per share -
and then immediately exercise her option to sell
the shares at 150. Ms. Optimist will have made
5,000 (100 shares x 50). Value of the put
option on the exercise date is 5000. Again, let
St be the stock price on the exercise date,
then Payoff on Expiration Date If St lt 150,
then call option value 150 - St If St ?
150, then call option value 0
25
A Graphical View of a Put Option
26
Put Option Writers Point of View
27
. . . and a covered put writers view
28
Call and Put Option Quotes
Mobil Oil Corporation
Column 1 - Stock of Mobil Oil closed at 697/8
per share on the previous day (Monday, January
20). Column 2 3 - Indicates that Monday's
closing price for an option maturing at the end
of February with a striking price of 60 was
101/4. Because it is a 100-share contract, the
cost of the contract is 1025. Columns 6, 7, 8
- Quotes on puts for example, a put maturing in
February with an exercise price of 65 sells at
5/8.
29
Some Observations on Option Pricing

• For a given exercise price, the premium steadily
  increases moving across time (from February to
  April). This occurs because of an options time
  value.
• Time value of an option is that portion of an
  option's premium that represents what investors
  are willing to pay in hopes that the option will
  increase in value as the stock price increases.
• Farther away from expiration, the greater a put
  or call option's time value will be.
• For a given expiration date (reading down a
  column), a call's premium decreases, but a put's
  premium increases.
• EX. In February, the 60 call is "in-the-money"
  (has intrinsic value), the 75 call is
  "out-of-the-money" (has no intrinsic value). With
  the puts, just the opposite occurs.

30
Option Behavior vs. Stock Behavior

• The value of the call increases with the value of
  the stock, while the value of the put decreases
  with value of the stock.
• Unless the option is certain to be exercised, the
  absolute dollar change in the stock value is
  greater than the accompanying absolute dollar
  change in the option value.
• The difference between the absolute dollar
  changes for the stock and for the options becomes
  smaller as the options move "out-of-the money" to
  "in-the-money".
• The absolute percentage changes in the option
  prices are greater than the accompanying absolute
  percentage changes in the stock price.
• The difference in the absolute percentage
  changes between the stock and the options again
  becomes smaller as the options move from being
  out-of-the money" to "in-the-money". Thus,
  out-of-the-money options are inherently more
  volatile.

31
Financial Options and Leverage

• Major advantages of buying puts and calls are
  their speculative appeal in
• leveraging potential investment returns
• limiting potential losses
• reducing the required investment.
• A Call Option Example
• Let's say you are bullish on Mobil Oil stock
  that is currently priced at 40/share. You could
• (1) Invest 4000 in 100 shares or
• (2) Invest only 400 to buy a Mobil June 40
  call
• Let's compare and contrast the absolute dollar
  profits (losses) and percentage returns
  associated with different stock prices at the
  exercise date.
• 1. Stock rises to 50.
• 2. Stock Remains at 40.
• 3. Stock drops to 30.

32
How Options Affect Risks and Rewards
Stock Price 40 Call Option Premium 4
Put Option Premium 4

• Contrast gains between stock and call at 50
  price Need less capital to buy the option and
  participate for in stocks appreciation.
• The more leveraged the investment, the greater
  the risk that a large percentage of it will be
  lost.
• Compared to stock ownership, dollar losses for
  calls and puts are limited.

33
Combinations of options . . .
34
provide the building blocks . . .
35
for more complex contracts.
36
What about a riskless return?

• One strategy in the options market may offset
  another strategy, resulting in a riskless return.
• Example of an Offsetting Strategy Suppose the
  stock price is currently 44. At the expiration
  date, the stock will either be 58 or 34.
  Consider the following (1) Buy the stock (2)
  Buy the put and (3) Sell the call.
• Payoffs at expiration are

37
What About Your Investment Capital?

• Suppose you originally paid 44 for the stock, 7
  for the put and received 1 for selling the call.
• Because you paid out 50 and will receive 55 in
  one year, you have earned 10 ROR - the
  equilibrium rate of interest. Conversely, if the
  put sold for only 6 your initial investment
  would be 49. You would then have a
  non-equilibrium return of 12.2 (55/49 -1).
• Put-Call Parity It can be proved that, in order
  to prevent arbitrage, the prices at the time you
  take on your original position must conform to
  the following
• Value of Stock Value of Put - Value of Call
  PV of Exercise Price
• 44 7 -
  1 50 55/1.10
• This shows that the values of a put and call with
  the same exercise price and same expiration date
  are precisely related to each other.

38
Valuing Options - A Qualitative Look

• Features of the Option Contract and Their Effects
• Exercise Price (X)
• Higher the exercise price, lower the value of the
  call option.
• However, the value of a call option cannot be
  negative, no matter how high we set the exercise
  price.
• As long as the is some probability that the price
  of the underlying asset will exceed the exercise
  price before the expiration date, the option will
  have value.
• Time to Expiration (t)
• Value of an American call option must be at least
  as great as the value of otherwise identical
  option with a shorter term to expiration.
• Ex Consider two options, one with a maturity of
  nine months and one with a maturity of six
  months. The nine-month option has the same rights
  as the 6-month call and also has an additional 3
  months within which these rights can be
  exercised. (Not necessarily true with European
  options).

39
Qualitative Observations (contd)

• Stock Price (S)
• Higher the stock price, the more valuable the
  call option will be.
• Relationship between stock price at exercise date
  and option value can be shown by the convex
  curve.
• Variability of the Underlying Asset (?2)
• Greater the variability of the underlying asset,
  the more valuable the call option will be.
• Ex. Suppose just before call expires, stock
  price will be either 100 w/probability of 0.5
  or 80 with probability of 0.5. What will be the
  value of a call with an exercise price of 110?
• Now assume the stock price is much more variable
  say 60 as the worst case and 120 as best case
  with same probabilities. Note that the expected
  value of the stock is the same (90). However,
  now the call option has value because there is a
  one-half chance that the stock value will go to
  120.

40
Qualitative Observations (contd)

• Variability of the Underlying Asset (contd)
• An Important Distinction Between Stocks and
  Options
• If investors in the marketplace are risk averse,
  a rise in the variability of the stock will
  decrease its market value.
• However, holders of calls only are concerned
  about the positive tails of the probability
  distribution as a consequence, a rise in the
  variability of the underlying stock increases the
  market value of the call.
• The Interest Rate (r)
• Buyers of calls do not pay the exercise price
  until they exercise the option, if they do so at
  all.
• The delayed payment is more valuable when
  interest rates are high and less valuable when
  interest rates are low.
• Value of a call is positively related to interest
  rates.

41
OPT - A Quantitative Approach

• Ex. Applying a Two-State Option Model
• Suppose the market price of a stock is 50 and it
  will be either 60 or 40 at the end of the year.
• Further suppose that there exists a call option
  for 100 shares of this stock with a one year
  expiration date and a 50 exercise price. Assume
  investors can borrow at 10.
• Let's examine two possible trading strategies
• 1. Buy a call on the stock.
• 2. Buy 50 shares of the stock and borrow a
  duplicating amount. (Duplicating amount is the
  amount of borrowing necessary to make the future
  payoffs equivalent.

42
OPT - A Quantitative Approach (contd)

• Future payoff structure of buy a call is
  duplicated by the strategy of buy stock and
  borrow.
• Since the strategies are equivalent as far as
  market traders are concerned, the two strategies
  must have the same cost.

43
OPT - A Quantitative Approach (contd)

• The cost of purchasing 50 shares of stock while
  borrowing 1818 is
• Buy 50 shares of stock 50 x 50
  2500
• Borrow 1818 at 10
  -1818
• 
  682
• Because the call option gives the same return,
  the call must be priced at 682. This is the
  value of the call option in a market where no
  arbitrage profits exist.
• Some Comments
• Note that we found the exact option value without
  even knowing the probability that the stock would
  go up or down!
• The optimist and the pessimist would agree on the
  option value. How could that be?
• The answer is that the current 50 stock price
  already balances the views of the optimists and
  pessimists - the option reflects that balance
  because its value depends on the stock price.

44
OPT and the Black-Scholes Model

• Extension of the two-state model
• BS Model allows us to value a call in the real
  world by
• Determining the duplicating combination at any
  moment
• Valuing the option based on the duplicating
  strategy.
• The Model

where
S Current stock price E Exercise price
of call r Continuous risk-free rate of
return (annualized) ?2 Variance (per year) of
the continuous return on the stock t Time
(in years) to expiration date In addition, N(d)
equals probability that a standardized, normally
distributed, random variable will be less than or
equal to d.
45
A Simple Example of Black-Scholes

• Consider the Big Oil Company (BOC). On 10/4/97,
  BOC April 49 call option had a closing value of
  4. The stock itself is selling at 50. On 10/4,
  the option had 199 days to expiration (maturity
  date 4/21/98). The annual risk-free interest
  rate is 7 percent.
• From this we can determine the following
  variables
• 1. Stock price, S, is 50.
• 2. Exercise price, E, is 49.
• 3. Risk-free rate, r, is 0.07.
• 4. Time to maturity in years, t, 199/365.
• Estimates of Variance may differ but must
  obviously involve analysis of a series of past
  price movements for the stock. Let's assume
  variance of returns on BOC is estimated at
  0.09/year.

46
Black-Scholes Example (contd)
Step 1 Calculate d1 and d2
Step 2 Calculate N(d1) and N(d2)
From a table of the cumulative probabilities of
the standard normal distribution, we know
that N(d1) N(0.3743) 0.6459 N(d2)
N(0.1528) 0.5607 Interpretation N(d) is the
cumulative probability of d. For example, N(d1)
tells us that there is a 64.59 percent
probability that a drawing from the standardized
normal distribution will be below 0.3743.
47
Black-Scholes Example (contd)
Step 3 Calculate the call option value (C)
Note The estimated price of 5.85 is greater
than the actual price of 4 this implies the
call option is underpriced!
48
Why is Black-Scholes So Attractive?

• Four of the five necessary parameters are
  observable.
• Investor's risk aversion does not affect value
  formula can be used by anyone, regardless of
  willingness to bear risk.
• It does not depend on the expected return of the
  stock.
• Investors with different assessments of the
  stock's expected return will nevertheless agree
  on the call price.
• As in the two-state example, the call depends on
  the stock price, and that price already balances
  investors' divergent views.