Components of Thermodynamic Cycles

1
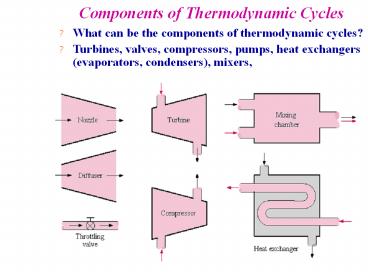
Components of Thermodynamic Cycles

• What can be the components of thermodynamic
  cycles?
• Turbines, valves, compressors, pumps, heat
  exchangers (evaporators, condensers), mixers,

2
BUTTERFLY VALVE
GATE VALVE
SPHERE VALVE
PIPE CRACK
GLOBE VALVE
3
Throttling Devices (Valves)

• Typical assumptions for throttling devices
• No work
• Potential energy changes are zero
• Kinetic energy changes are usually small
• Heat transfer is usually small
• Two port device

4
Look at energy equation
Apply assumptions from previous page
0
0
0
0
We obtain
or
Does the fluid temperature
increase,
decrease, or
remain constant
as it goes through an adiabatic valve?
5
Look at implications If the fluid is
liquid/vapor

• During throttling process
• The pressure drops,
• The temperature drops,
• Enthalpy is constant

6
Look at implications if fluid is an ideal gas
Cp is always a positive number, thus
7
P1 gt P2
P
S
T
T
v
v
s
diminui
Pressão Entalpia Temperatura En. Interna Entropia
constante
gás ideal hh(T), portanto T fica constante
gás ideal uu(T), portanto u fica constante
diminui, Ds Cpln(T2/T1)-Rln(P2/P1)
8
(No Transcript)
9
Cold embrittlement (dureza e rigidez mas baixa
resistência a tensão)
T-1000 (Robert Patrick)
10
Cold embrittlement (dureza e rigidez mas baixa
resistência a tensão)
Consequences of the Temperature Drop on Material
Strenght
Low temperature embrittlement does affect most
materials more or less pronounced. It causes
overloaded components to fracture spontaneously
rather than accommodating the stress by plastic
deformation. The picture shows a fractured
fitting whose material was not suitable for low
temperatures.
11
TEAMPLAY
Refrigerant 12 enters a valve as a saturated
liquid at 0.9607 Mpa and leaves at 0.1826 MPa.
What is the quality and the temperature of the
refrigerant at the exit of the valve?
State (1) Liquid saturated, x0 Psat 0.9607
MPa Tsat ? Hliq ?
State (2) Liqvap x? Psat 0.1826 MPa Tsat
? Hliq ?
0.33
40oC
-15oC
75kJ/kg
22 180 kJ/kg
12
P-h Diagram of an Ideal Vapor-Compression
Refrigeration Cycle

• Isentropic compression (1 to 2)
• Constant pressure condensation (2 to 3)
• Isenthalpic expansion (3 to 4)
• Constant pressure evaporation (4 to 1)

The refrigerant enters the compressor as a
saturated vapor and is cooled to the saturated
liquid state in the condenser. It is then
throttled to the evaporator and vaporizes as it
absorbs heat from the refrigerated space.
13
Heat exchangers are used in a variety of
industries

• Automotive - radiator
• Refrigeration - evaporators/condensers
• Power production - boilers/condensers
• Power electronics - heat sinks
• Chemical/petroleum industry- mixing processes

14
Something a little closer to home..
15
Heat Exchangers
16
Condenser/evaporator for heat pump
17
Heat Exchangers

• Now, we must deal with multiple inlets and
  outlets

If we have steady flow, then
18
Heat Exchangers
0
0

0, (sometimes negligible)
0, (usually negligible)
0, (sometimes negligible)
0, (usually negligible)
19
Heat Exchangers

• And we are left with

The energy change of fluid A is equal to the
negative of the energy change in fluid B.
20
mw ? kg/s hw 100 kJ/kg
mvap 0.1 kg/s hvap 2776 kJ/kg
mc ? kg/s hc 200 kJ/kg
Resp. mw 2.576 kg/s
21
Nozzles and Diffusers

• Nozzle--a device which accelerates a fluid as the
  pressure is decreased.

• Diffuser--a device which decelerates a fluid and
  increases the pressure.

These configuration is for sub-sonic flow.
22
For supersonic flow, the shape of the nozzle is
reversed.
Nozzles
23
General shapes of nozzles and diffusers
nozzle
diffuser
Subsonic flow
nozzle
diffuser
Supersonic flow
24
conservation of energy
q 0 (adiabatic) w 0 (these are not work
producing devices neither is work done on them)
25
Sample Problem
An adiabatic diffuser is employed to reduce the
velocity of a stream of air from 250 m/s to 35
m/s. The inlet pressure is 100 kPa and the inlet
temperature is 300C. Determine the required
outlet area in cm2 if the mass flow rate is 7
kg/s and the final pressure is 167 kPa.
OUTLET P2 167 kPa V2 35 m/s A2?
26
Conservation of Mass Steady State Regime
But we dont know v2!
Remember ideal gas equation of state?
and
We know T1 and P1, so v1 is simple. We know P2,
but what about T2?
NEED ENERGY EQUATION!!!!
27
Energy Eqn
If we assumed constant specific heats, we could
get T2 directly
The ideal gas law
And the area
28
Vapor Power Cycles

• Well look specifically at the Rankine cycle,
  which is a vapor power cycle.
• It is the primary electrical producing cycle in
  the world.
• The cycle can use a variety of fuels.

29
(No Transcript)
30
(No Transcript)
31
(No Transcript)
32
Well simplify the power plant
33
Carnot Vapor Cycle
T
compressor and turbine must handle two phase
flows!
TH lt TC
TH
1
2
3
4
TL
s

• The Carnot cycle is not a suitable model for
  vapor power cycles because it cannot be
  approximated in practice.

34
Ideal power plant cycle is called the Rankine
Cycle

• The model cycle for vapor power cycles is the
  Rankine cycle which is composed of four
  internally reversible processes
• 1-2 reversible adiabatic (isentropic) compression
  in the pump
• 2-3 constant pressure heat addition in the
  boiler.
• 3-4 reversible adiabatic (isentropic) expansion
  through turbine
• 4-1 constant pressure heat rejection in the
  condenser

35
Ideal power plant cycle is called the Rankine
Cycle

• The model cycle for vapor power cycles is the
  Rankine cycle which is composed of four
  internally reversible processes
• 1-2 reversible adiabatic (isentropic) compression
  in the pump
• 2-3 constant pressure heat addition in the
  boiler.
• 3-4 reversible adiabatic (isentropic) expansion
  through turbine
• 4-1 constant pressure heat rejection in the
  condenser

36
What are the main parameters we want to describe
the cycle?
Net Power
Net Specific Work
Efficiency
or
37
Start our analysis with the pump
The pump is adiabatic, with no kinetic or
potential energy changes. The work per unit mass
is
38
Boiler is the next component

Boilers do no work. In boilers, heat is added to
the working fluid, so the heat transfer term is
already positive. So,
39
Proceeding to the Turbine
Turbines are almost always adiabatic. In
addition, well usually ignore kinetic and
potential energy changes
40
Last component is the Condenser
Condensers do no work (they are heat exchangers),
and if there is no ?KE and ?PE,
41
Efficiency
42
Start an analysis
A Rankine cycle has an exhaust pressure from the
turbine of 0.1 bars. Determine the quality of
the steam leaving the turbine and the thermal
efficiency of the cycle which has turbine inlet
pressure of 150 bars and 600?C.
Assumptions
Pump and turbine are isentropic P2 P3 150
bars 15 MPa T3 600?C P4 P1 0.1 bars
0.01 MPa Kinetic and potential energy changes
are negligible
43
Put together property data
Pump (1 to 2) -gt isoentropic (const.
volume) Boiler heat exchanger (2 to 3) -gt
const. pressure Turbine (3 to 4) -gt isoentropic
Condenser heat-exchanger (4 to 1) -gt const.
pressure
44
Property Data
45
Let start with pump work
46
More calculations...
Enthalpy at pump outlet
Plugging in some numbers
47
How Can I Get The Pump Outlet Temp?
If the Enthalpy at pump outlet is 206.93 KJ/kg,
then consider the compressed liquid a the same
temperature of the saturated liquid which has h
206.93 KJ/kg Interpolating from the saturated
steam table one finds 49oC
48
Calculate heat input
49
Turbine work
Isentropic
Turbine work
50
Overall thermal efficiency
51
Some general characteristics of the Rankine cycle

• Low condensing pressure (below atmospheric
  pressure)
• High vapor temperature entering the turbine (600
  to 1000?C)
• Small backwork ratio (bwr)

52
Questions

• Consider the ideal Rankine cycle from 1-2-3-4

• How would you increase its thermal efficiency ??
• What determines the upper T limit?
• What determines the lower T limit?

• Improve the efficiency of the Rankine Cycle
  decrease exhaust pressure of turbine
• decreases condensing temperature
• increases work output
• increases heat input
• decreases quality at turbine outlet

53
Lowering Turbine Exit Pressure

• The average temperature during heat rejection can
  be decreased by lowering the turbine exit
  pressure.
• Consequently, the condenser pressure of most
  vapor power plants is well below the atmospheric
  pressure.

54
Reducing Condenser Pressure

• Notice that reducing the condenser pressure
  (which will lower the temperature of heat
  rejection and again increase the efficiency) will
  also reduce the quality of the steam exiting the
  turbine.
• Turbines do not like to see water coming out the
  exhaust.
• Lower qualities mean water droplets are forming
  before the steam leaves the turbine.
• Water droplets lead to turbine blade erosion.
• Efforts are made to keep the quality gt 90.

55
Raising Boiler Pressure

• The average temperature during heat addition can
  be increased by raising the boiler pressure or by
  superheating the fluid to high temperatures.
• There is a limit to the degree of superheating,
  however, since the fluid temperature is not
  allowed to exceed a metallurgically safe value.

56
Increase Superheat (Boiler Temperature)
Work output increases faster than heat input, so
the cycle efficiency increases.
higher quality
Increasing Superheat increases heat input
increases work output increases quality at
turbine outlet may produce material problems
if temperature gets too high
57
Effect of Increasing Boiler Pressure on the Ideal
Rankine cycle keeping constant the Boiler Outlet
Temperature Tmax
58
TEAMPLAY

• Consider a simple ideal Rankine cycle with fixed
  turbine inlet temperature and condenser pressure.
  What is the effect of increasing the boiler
  pressure on

Pump work input (a) increases, (b) decreases, (c) the same
Turbine work output (a) increases, (b) decreases, (c) the same
Heat supplied (a) increases, (b) decreases, (c) the same
Heat rejected (a) increases, (b) decreases, (c) the same
Cycle efficiency (a) increases, (b) decreases, (c) the same
Moisture content at turbine exit (a) increases, (b) decreases, (c) the same
59
Simple schematic of Rankine reheat cycle
60
The Ideal Reheat Rankine Cycle
61
Reheat on T-s diagram
Note that T5 lt T3. Many systems reheat to the
same temp (T5T3).
62
Cogeneration

• The production of more than one useful form of
  energy (such as process heat and electric power)
  from the same energy source is called
  cogeneration.
• Cogeneration plants produce electric power while
  meeting the process heat requirements of certain
  industrial processes. This way, more of the
  energy transferred to the fluid in the boiler is
  utilized for a useful purpose.
• The fraction of energy that is used for either
  process heat or power generation is called the
  utilization factor of the cogeneration plant.

63
An Ideal Cogeneration Plant

64
Schematic and T-s Diagram for Cogeneration
65
Combined Gas-Steam Power Plant