Flashback

1
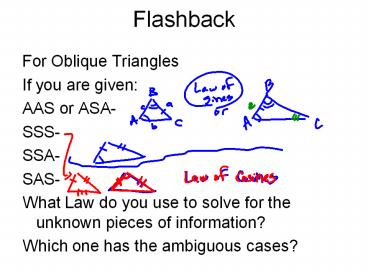
Flashback

• For Oblique Triangles
• If you are given
• AAS or ASA-
• SSS-
• SSA-
• SAS-
• What Law do you use to solve for the unknown
  pieces of information?
• Which one has the ambiguous cases?

2
Chapter 6.5Trigonometric Form of a Complex
Number
3
The Complex Number
Imaginary Axis
Real Axis
4
Finding the Absolute Value of a Complex Number

• Plot
• and find the absolute value

Imaginary Axis
Real Axis
5
Trigonometric Form Of a Complex Number
Imaginary Axis
Real Axis
6
Write in Trigonometric
Form
Imaginary Axis
Real Axis
7
Try 15 page 440
8
Writing a Complex Number in Standard Form

• Write
  in
• Standard Form

9
Multiplying Complex Numbers in Trigonometric Form
Find the product of the complex numbers
10
Dividing Complex Numbers in Trigonometric Form
Find the Quotient of the complex numbers
11
Powers of Complex Numbers

• DeMoives Theorem
• If is a
  complex number and n is a positive integer, then

12
Use DeMoives Theorem to find
13
Remember that an nth degree polynomial has at
least one complex zero (root) and at most n
complex zeros (roots).

• A complex number also has n nth roots. For
  example,
• has 3 cube roots. To find them you need to use
  this formula for a complex number
• The n distinct nth roots are given by
• where k 0, 1, 2, , n - 1

14
Example Find the 4 fourth roots of . If

• the n distinct nth roots are given by
• where k 0, 1, 2, , n - 1