Resistance Characteristic

1
Resistance Characteristic
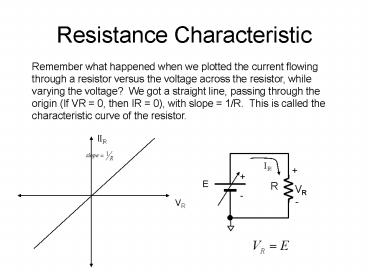
Remember what happened when we plotted the
current flowing through a resistor versus the
voltage across the resistor, while varying the
voltage? We got a straight line, passing through
the origin (If VR 0, then IR 0), with slope
1/R. This is called the characteristic curve of
the resistor.
I
IR
IR


E
R
VR
-
-
VR
2
Resistance Characteristic
The slope-intercept form of the equation for a
line is y mx b, where y is the vertical
coordinate, x is the horizontal coordinate, m is
the slope and b is the y-intercept. In the case
of the characteristic curve of a resistor R, m
1/R, b 0 (because the line passes through the
origin), y IR, and x VR
The resulting equation is
I
IR
The I-V characteristic maps a particular voltage
to a particular current.
or,
VR
where VR and IR are in Volts and Amperes,
respectively, and R is the resistance in ohms.
3
Resistance Characteristic
Intuitively, this makes sense Increasing the
battery Voltage E increases VR (according to
KVL), and is like increasing the pressure at the
inlet to a pipe. Increasing pressure increases
the flow rate, which is analogous to current IR.
Reducing the resistance is analogous to
increasing the pipes diameter, which also
increases the flow rate.
I
IR
Increasing Voltage increases current
IR


E
R
VR
-
-
4
Ohms Law
The equation
is called Ohms Law, and its true for any
resistance The resistance of an ideal resistor,
the dynamic resistance (the slope of the
characteristic curve at a particular point) of an
element with a nonlinear characteristic curve
(e.g., a diode or transistor), or the
resistance of a wire (which wed rather was
zero). if we know R and IR, but not VR, we can
solve the equation
I
IR
for VR
VR
if we know VR and IR, but not R, we can solve for
R
5
Ohms Law
Lets take a simple example A battery (Voltage
E) in series with a resistor R1. KVL tells us
that E V1, and KCL tells us that the same
current, I, flows through both the battery and
the resistor. Ohms law tells us
or


R1
E
V1
-
I
-
6
Ohms Law
Lets add another resistor in series, as shown.
Now,
So,
R2
and

-
V2


R1
V1
E
-
-
7
Ohms Law
Turning our attention to R2,
R2

-
V2
Ohms law, KVL and KCL allowed us to fully
analyze this circuit. We were able to find the
current flowing around the loop, and the Voltages
across each of the resistors.


R1
V1
E
-
-
8
Nonlinear Resistance
The characteristic of an ideal resistor is a
straight line. In other words, a resistor has a
linear I-V characteristic. Some devices, such as
transistors and diodes, have nonlinear I-V
characteristics. This means plotting current
versus voltage does not result in a straight line.
I
diode characteristic
I
resistor characteristic
reverse bias
Forward Bias
The diodes characteristic can be divided into
three regions, each of which is approximately
linear reverse breakdown, reverse bias, and
forward bias.
V
reverse breakdown
9
Nonlinear Resistance
Pick a voltage in the forward-bias region. Now,
if the voltage is increased by a small amount,
the current increases by a large amount. If the
Voltage is reduced slightly (but stays in the
forward-bias region) the current diminishes by a
small amount. Within the forward-bias region,
the characteristic can be approximated by a line
with a large slope.
I
I
Recall that the slope of the characteristic is
1/R, so a large (steep) slope means small
resistance. We can say that a forward-biased
diode has a small (ideally, zero) dynamic
resistance. In other words, the forward-biased
diode conducts current easily.
V
linear approximation to diode in forward-bias
region
10
Nonlinear Resistance
Next, consider a point on the diode
characteristic in the reverse-bias region. A
large increase or decrease in voltage (but not so
large as to leave the reverse-bias region)
results in a very small increas or decrease in
current. The line approximating the
characteristic in this region has very small
slope, so the dynamic resistance of a reverse-
biased diode is very large ideally infinite,
like an open circuit.
I
I
V
linear approximation to diode in reverse-bias
region
11
Nonlinear Resistance
Finally, consider a point on the diode
characteristic in the reverse-breakdown region.
A small increase or decrease in voltage (staying
within the reverse-breakdown region ) results
in a large increase or decrease in current. The
line approximating the characteristic in this
region has large slope, so the dynamic resistance
of a diode in reverse-
breakdown is very small.
I
I
V
linear approximation to diode in
reverse-breakdown region
12
Nonlinear Resistance
Finally, consider a point on the diode
characteristic in the reverse-breakdown region.
A small increase or decrease in voltage (staying
within the reverse-breakdown region ) results
in a large increase or decrease in current. The
line approximating the characteristic in this
region has large slope, so the dynamic resistance
of a diode in reverse-
breakdown is very small.
I
I
V
linear approximation to diode in
reverse-breakdown region
13
Nonlinear Resistance
Heres an example. Wed like to light an LED.
We can increase the LEDs brightness by
increasing the current flowing through it, until
the current becomes so large it destroys the LED.
We need to make sure the current is great enough
to make the LED as bright as we want, but not so
great that the LED destroys itself. W do this
with a resistor in series with the LED.
I
I
VR
-

R
I
12V


VLED
VLED
-
-
2.5 V
14
Nonlinear Resistance
The LEDs I-V characteristic shows that its
forward-bias voltage is about 2.5 V. The LED
must be forward biased to light the LED. Only a
very large (large enough to destroy the LED)
increase in current could produce an increase
beyond 2.6 V or so, so its a reasonable
approximation to say
I
I
VR
-

R
I
12V


VLED
-
-
VLED
2.5 V
15
Nonlinear Resistance
Examining the manufacturers data sheet, we find
that an LED current of 20 mA should make the LED
sufficiently bright. Applying KVL around the
loop,
I
I
VR
-

R
I
12V


VLED
-
-
VLED
2.5 V
16
Nonlinear Resistance
Applying Ohms law,
475W is not a standard resistor value, but 470W
and 510W are.
I
I
VR
-

R
I
12V


VLED
-
-
VLED
2.5 V
17
Power Rule
Weve already seen that the power consumed by a
circuit element (e.g., a resistor) is given
Recall that power is the rate at which energy is
converted from one form to another. For example,
a 1 Hp motor converts electrical energy to
kinetic energy at a rate of 746 J/sec., or 746
Watts (1 Hp 746 W).
In other words, if K represents energy and t
time,
I

Current is the rate of flow of charge, so if Q
charge and I current,

E
R
VR
-
-
and Voltage is energy per unit charge
18
Power Rule
So we can prove P IV, the power rule
Resistive elements consume electrical energy by
converting it to heat. This is called
dissipation, because as heat is produced it is
radiated away to the surrounding. The faster
heat is consumed (that is, the faster electrical
energy is converted to heat, which means greater
power),
the faster it must be radiated away. The only
way (aside from increasing the surface area of
the component) to make it radiate heat faster is
for the temperature to rise, but if the
temperature rises too much, damage can result.
All components have a maximum power dissipation
rating, which must not be exceeded.
I


E
R
VR
-
-
19
Power Rule
Consider our simple LED example. We found
previously that
So
The LEDs power dissipation rating had better be
at least 50 mW.
We also found that VR 9.5 V, so the resistor
dissipates
VR
-

R
I
12V


And the power delivered by the battery is
VLED
-
-
which can also be found like this
20
Power Rule
Weve already seen that the power consumed by a
circuit element (e.g., a resistor) is given
In other words, the power consumed by an element
is equal to the product of the voltage drop
across it and the current flowing through it.
For the resistor shown below,
Ohms law tells us that
I


Substituting,
E
R
VR
-
-
Now we have another way of calculating the power
consumed by a resistor Its the product of the
resistance and the square of the current through
the resistor.
21
Power Rule
Another form of the power rule is found as
follows Start with the basic power rule
If we know VR and R, we can solve Ohms law for I
Substituting,
I


E
R
We now have yet another way of calculating the
power consumed by a resistor Its the quotient
of the square of the voltage across the resistor
divided by the resistance.
VR
-
-
22
Power Ratings
Through-hole-mount resistors, the kind we use in
the lab, are available with power ratings of 1/8
W, ¼ W, ½ W, 1 W, and 2 W. Suppose we have a 470
W resistor (a standard value) rated at ¼ W. What
is the maximum voltage it can drop?
I


E
R
VR
-
-
23
A BJT Circuit
Lets look at the example in the book, with a few
differences. We see that IC 10 mA, and IB
430 mA. KCL gives us
Ohms law tells us

RC
3V
-
10 mA

2 V
LED
-
430 mA
VRB

-


The definition of b gives us
4V
9V

RB 10K
-
-

0.7 V
5V
24
A BJT Circuit
Lets let b increase to 40. The base-emitter
loop is unchanged, so IB is still 430 mA.

RC
6V
-
20 mA

2 V
LED
-
430 mA
The voltage across RC is now
VRB

-


4V
9V

RB 10K
-
-

0.7 V
5V
25
A BJT Circuit
a. The base current is unchanged, so the power
generated by the 5 V supply is still 2.15 mW

RC
6V
-
20 mA

2 V
LED
b. and c. Similarly, the power dissipated by RB
is still 1.85 mW, and the power dissipated by the
base-emitter junction is still 301 mW.
-
430 mA
VRB

-


4V
9V

RB 10K
-
-

0.7 V
5V
d. The power delivered by th 9 V supply is
doubled, to 180 mW
26
A BJT Circuit

• The power dissipated by RC is quadrupled, to 120
  mW.
• power dissipated by the LED is doubled, to 40 mW.

RC
6V
-
20 mA

2 V
LED
-
430 mA
VRB

-


4V
9V

RB 10K
-
-

0.7 V
5V
27
A BJT Circuit
g. KVL gives us the collector-emitter voltage,
VCE

RC
6V
-
20 mA

2 V
LED
-
430 mA
The power dissipated by the collector-emitter
output is now
VRB

-


4V
9V

RB 10K
-
-

0.7 V
5V
28
A MOSFET Circuit
Heres the circuit used by Herrick in his
example. Lets work that example, except that
the load voltage VLoad is doubled to 6 V. If the
load voltage is doubled, the source current must
also be doubled, to 2 A. Examination of the
MOSFET characteristic reveals that
Applying KVL around the gate-source loop yields


VDS
12 V

VDD
-
-

VGS

VIN
Rload 3W
Vload
-
29
A MOSFET Circuit
The load power is now given by
so the load power is more than doubled. The
supply power is doubled, to 18 W. The MOSFET
output power is


VDS
12 V

VDD
-
-

VGS

VIN
Rload 3W
Vload
-
30
An Opamp Circuit
Heres Herricks opamp example. Suppose we
change VIN from 1 V to 2 V The ideal op-amp
assumptions say that Va VIN, because the
Voltage difference between the inverting and
noninverting inputs must be zero.
Having found Va, we see that the VRi Va, so
Ini
VIN
2 V
VOUT
KCL tells us that IRf IRi, so Ohms law says
IRf
2 V
Rf2.7KW
a

-
Rload 1KW
Ri 1KW
VRf

VRi
IRi
-
31
An Opamp Circuit
KVL tells us that
Ini
VIN
2 V
VOUT
ILoad
so
IRf
2 V
Rf2.7KW
a

-
Rload 1KW
Ri 1KW
VRf

VRi
IRi
-
32
Energy
Our lab exercises all require the use of a power
supply. What does a power supply do? It
supplies power! What does that mean? Whats
Power? Power is the rate at which energy is
converted from one form to another. If K
represents the energy converted in a time
interval Dt, the rate of conversion is K/Dt, and
the power which must be supplied for conversion is
An electric motor converts electrical energy to
mechanical (kinetic) energy. A motor which is
100 efficient (that is, it converts all of the
electrical energy supplied to it to useful work,
none is wasted as heat or other forms) and is
rated at 1 horsepower (1 hp 746 Watts) converts
energy at the rate of
33
Energy
So a 1 hp. motor can convert 746 Joules of
electrical energy to 746 Joules of work every
second. Where does the electrical energy come
from? A power supply!
120 V
I

1 horsepower DC motor
M
Here the power supply is a 120 Volt battery. The
motor converts 746 J/sec. of energy, so the
battery must supply 746 J/sec., or 746 W. of
power. remember that P IV, so the current
supplied must be
34
Energy
Notice that in this example the current is
flowing out of the batterys terminal, and into
the more positive terminal of the motor. There
is a voltage rise across the battery terminals,
and a drop across the motor.
120 V
I


1 horsepower DC motor
M
VM
-
Since current flows out of the positive battery
terminal, the battery is supplying energy to the
motor.
35
Energy
Some batteries, like the one in your car, are
rechargeable. That is, they can convert
electrical energy to chemical energy for storage,
then reverse the process to supply electrical
energy.
120 V
I


DC generator
M
VM
-
Heres the same battery, connected to a DC
generator (which may be identical to the DC
motor, except that its shaft is driven by a
gasoline or diesel engine). The generator
converts mechanical energy to electrical energy.
Current flows out of the generators positive
terminal and into the batterys positive
terminal, so now the generator is supplying
energy to the battery.
36
Energy
The battery now receives energy from the
generator at the rate of
Joules per sec., or Watts
120 V
ICharge


DC generator
M
VM
-
So the battery stores energy at that rate. If
the generator supplies charging power to the
battery at 746 Watts, then the battery must
charge 1 minute for every minute the motor is to
run at full power.
37
Energy
Of course, the wires connecting the battery to
the generator have some resistance. Lets say
its 1 Ohm. The generator voltage must be
slightly greater than the battery voltage, to
force current to flow from the
generator to the battery. To charge at a 746
Watt rate, the current must be 6.22 A, so
1W


ICharge
M
120 V
DC generator
VM
-
Similarly, the voltage across the motor when its
receiving power from the battery must be lower
than the battery voltage, so current flows out of
the battery. to run at full power,
38
Energy
A DC motor does produce a voltage across its
terminals while its running, called the back emf,
or counter emf. Thats where VM comes from.
1W


ICharge
M
120 V
DC Motor
VM
-