MODELING THE DOUBLYEXCITED STATE WITH REALTIME

1
MODELING THE DOUBLY-EXCITED STATE WITH REAL-TIME
TIME-DEPENDENT HARTREE-FOCK AND DENSITY
FUNCTIONAL THEORIES
Christine M. Isborn, Xiaosong Li University of
Washington Seattle, WA 98195
Multi-electron excited states have become a hot
topic in many cutting-edge research fields, such
as the photophysics of polyenes and in the
possibility of multi-exciton generation in
quantum dots for the purpose of increasing solar
cell efficiency. However, obtaining
multi-electron excited states has been a major
obstacle as it is often done with
multi-configurational methods, such as CASSCF or
SAC-CI, which involve formidable computational
cost for large systems. Although they are
computationally much cheaper than
multi-configurational wave function based
methods, linear response adiabatic TDHF/TDDFT are
generally considered incapable of obtaining
multi-electron excited states 1-2. We have
developed a real-time TDHF/TDDFT approach that is
beyond the perturbative regime, in which we use
intense fields to drive electrons into the
doubly-excited state. This is done within the
adiabatic approximation of TDDFT, a realm in
which the doubly-excited state has been deemed
missing. We report results on simple
two-electron systems, including the energies and
dipole moments for the two-electron excited
states of H2 and HeH. These results are
compared to those obtained with the full
configuration interaction (CI) method. Upon
excitation, there is a shift in the single peak
of the absorption spectra, which is due to a
superposition of single-electron transitions from
the ground and doubly-excited states.
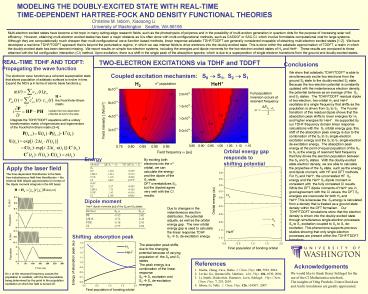
REAL-TIME TDHF AND TDDFT Propagating the wave
function
TWO-ELECTRON EXCITATIONS via TDHF and TDDFT
Conclusions
We show that adiabatic TDHF/TDDFT is able to
simultaneously excite two electrons from the
ground S0 state to the doubly-excited S2 state.
Because the two-electron potential is constantly
updated with the instantaneous electron density,
the potential behaves as an average of the S0
and S2 states. The TDHF/TDDFT residual dipole of
two-electron, two-orbital H2 and HeH oscillates
at a single frequency that shifts as the
population is driven from S0 to S2. The Fourier
transform of this residual dipole shows that the
absorption peak shifts to lower energies for H2
and higher energies for HeH. As supported by
our TDHF frequency domain linear response
calculations with the S2 orbital energy gap,
this shift of the absorption peak energy is due
to the combination of the S0 to S1
single-electron excitation energy and the S2 to
S1 single-electron de-excitation energy. The
absorption peak energy at the point of equal
population of the S0 to S2 is the energy of
resonant field frequency that fully drives the
electron population between the S0 and S2 states.
With the doubly-excited state electron density,
we are able to calculate the properties of the S2
state, such as the energy and dipole moment, with
HF and DFT methods. For H2 and HeH, the
uncorrelated HF S2 energy and the HeH S2
dipole moment is consistent with the fully
correlated CI results. While the DFT dipole
moments of HeH are in good agreement with the CI
values, the DFT S2 energies are inaccurate for
both H2 and HeH.This is because the S2 energy
is calculated from a density that is treated as a
ground state density within the DFT formalism.
Our TDHF/TDDFT simulations show that the electron
density is driven into the doubly-excited state
through simultaneous single-electron processes
S0 to S1 excitation coupled to S2 to S1
de-excitation. This phenomena supports previous
studies showing that only single-electron
processes are present within the TDHF/TDDFT
formalism.
Coupled excitation mechanism S0 ? S1, S2 ? S1
The electronic wave function as a coherent
superposition state that allows population of
adiabatic surfaces to evolve in time. Expand the
MOs f in terms of atomic basis functions ?
HeH
H2
s population
Full population inversion occurs at resonant
frequency
H the Fock/Kohn-Sham matrix
Update the time-dependent potential at every
time step
Field intensity W/cm2
Integrate the TDHF/TDDFT equations with a unitary
transformation matrix of eigenvalues and
eigenvectors of the Fock/Kohn-Sham matrix 3-4
Orbital energy gap responds to shifting
potential
Field frequency w au
Energy
By exciting both electrons into the s orbital,
we can calculate the energy and the dipole of the
S2 state. DFT overstabilizes S2, but the dipoles
agree very well with the CI results.
Apply the laser field
The time-dependent Hamiltonian is the field-free
instantaneous field-free Hamiltonian the
external field (dipole approximation) in terms of
the dipole moment integrals in the AO basis
Dipole moment
HeH dipole moments au of the S0 and S2 states
Due to changes in the instantaneous electron
distribution, the potential adjusts, as well as
the orbital energy gap. The new orbital energy
gap is used to calculate the linear response TDHF
S2 ? S1 de-excitation energy.
Shifting absorption peak
The absorption peak shifts due to the changing
potential because of varying population of the
S0 and S1 states. The peak energy is a
combination of the linear response S0 ? S1
excitation and S2 ? S1 de-excitation energies.
References
Acknowledgements
1. Maitra, Zhang, Cave, Burke. J. Chem. Phys.
120, 5932, 2004. 2. Levine, Ko, Quenneville,
Martinez. Mol. Phys. 104, 1039, 2006. 3. Li,
Smith, Markevitch, Romanov, Levis, Schlegel.
Phys. Chem. Chem. Phys. 7, 233, 2005. 4.
Isborn, Li, Tully. J. Chem. Phys. 126, 134307,
2007.
We would like to thank Berny Schlegel for the
use of the CI Mathematica notebook. The insights
of Oleg Prezhdo, Ernest Davidson and Andri
Arnaldsson are greatly appreciated.
An w at the resonant frequency causes the
population to oscillate, with the final
population being determined by the point in the
population oscillation at which the field is
turned off.