Areas of Parallelograms and Triangles

1
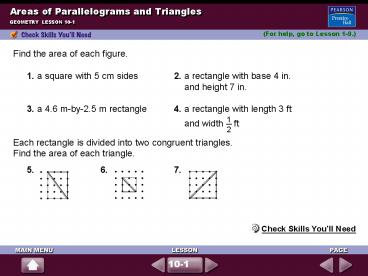
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
(For help, go to Lesson 1-9.)
Find the area of each figure.
Each rectangle is divided into two congruent
triangles. Find the area of each triangle.
Check Skills Youll Need
10-1
2
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
Solutions
10-1
3
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
You are given two sides with lengths 12 m and
10.5 m and an altitude that measures 8 m to the
side that measures 12 m. Choose the side with a
corresponding height to use as a base.
A bh Area of a parallelogram
A 12(8) Substitute 12 for b and 8 for h.
A 96 Simplify.
The area of the parallelogram is 96 m2.
Quick Check
10-1
4
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
A parallelogram has 9-in. and 18-in. sides. The
height corresponding to the 9-in. base is 15 in.
Find the height corresponding to the 18-in. base.
Find the area of the parallelogram using the
9-in. base and its corresponding 15-in. height.
A bh Area of a parallelogram A
9(15) Substitute 9 for b and 15 for h. A
135 Simplify.
The area of the parallelogram is 135 in.2
10-1
5
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
(continued)
Use the area 135 in.2 to find the height to the
18-in. base.
The height corresponding to the 18-in. base is
7.5 in.
Quick Check
10-1
6
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
A 195 Simplify.
Quick Check
10-1
7
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
The front of a garage is a square 15 ft on each
side with a triangular roof above the square. The
height of the triangular roof is 10.6 ft. To the
nearest hundred, how much force is exerted by an
80 mi/h wind blowing directly against the front
of the garage? Use the formula F 0.004Av2.
The total area of the front of the garage is 225
79.5 304.5 ft2.
10-1
8
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
(continued)
Find the force of the wind against the front of
the garage. F 0.004Av2 Use the formula for
force.
F 0.004(304.5)(80)2 Substitute 304.5 for A and
80 for v.
An 80 mi/h wind exerts a force of about 7800 lb
against the front of the garage.
Quick Check
10-1
9
Areas of Parallelograms and Triangles
GEOMETRY LESSON 10-1
150 ft2
15 m2
24 square units
187 in.2
6 cm
10-1
10
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
(For help, go to Lesson 10-1.)
Write the formula for the area of each type of
figure.
Find the area of each trapezoid by using the
formulas for area of a rectangle and area of a
triangle.
Check Skills Youll Need
10-2
11
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
Solutions
10-2
12
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
Solutions (continued)
4. Draw two segments, one from M perpendicular to
CB and the other from K perpendicular to CB.
This forms two triangles and a rectangle between
them. The area A of the triangle on the left
is bh (1)(2) 1. The area A of the
triangle on the right is bh (2)(2) 2.
The area A of the rectangle is bh (2)(2)
4. By Theorem 110, the area of a region is the
sum of the area of the nonoverlapping parts.
So, add the three areas 1 2 4 7
units2. 5. Draw two segments, one from A
perpendicular to CD and the other from B
perpendicular to CD. This forms two triangles and
a rectangle between them. The area A of the
triangle on the left is bh (2)(3) 3. The
area A of the triangle on the right is bh
(3)(3) 4.5. The area A of the rectangle is
bh (2)(3) 6. By Theorem 110, the area
of a region is the sum of the area of the
nonoverlapping parts. So, add the three areas 3
4.5 6 13.5 units2.
1 2
1 2
1 2
1 2
1 2
1 2
1 2
1 2
10-2
13
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
A 504 Simplify.
The area of the car window is 504 in.2
Quick Check
10-2
14
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
Because opposite sides of rectangle ABXD are
congruent, DX 11 ft and XC 16 ft 11 ft 5
ft.
10-2
15
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
A 162 Simplify.
The area of trapezoid ABCD is 162 ft2.
Quick Check
10-2
16
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
Find the area of kite XYZW.
Find the lengths of the diagonals of kite
XYZW. XZ d1 3 3 6 and YW d2 1 4 5
A 15 Simplify.
Quick Check
The area of kite XYZW is 15 cm2.
10-2
17
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
Find the area of rhombus RSTU.
10-2
18
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
The diagonals of a rhombus bisect each other, so
TX 12 ft.
You can use the Pythagorean triple 5, 12, 13 or
the Pythagorean Theorem to conclude that SX 5
ft.
SU 10 ft because the diagonals of a rhombus
bisect each other.
A 120 Simplify.
Quick Check
The area of rhombus RSTU is 120 ft2.
10-2
19
Areas of Trapezoids, Rhombuses, and Kites
GEOMETRY LESSON 10-2
1. Find the area of a trapezoid with bases 3 cm
and 19 cm and height 9 cm. 2. Find the area
of a trapezoid in a coordinate plane with
vertices at (1, 1), (1, 6), (5, 9), and (5, 1).
Find the area of each figure in Exercises 35.
Leave your answers in simplest radical form. 3.
trapezoid ABCD 4. kite with diagonals 20 m
and 10 2 m long 5. rhombus MNOP
99 cm2
26 square units
840 mm2
10-2
20
Areas of Regular Polygons
GEOMETRY LESSON 10-3
(For help, go to Lesson 8-2.)
1. 2. 3. 4. a hexagon with sides
of 4 in. 5. an octagon with sides of 2 3 cm
Find the area of each regular polygon. If your
answer involves a radical, leave it in simplest
radical form.
Find the perimeter of the regular polygon.
Check Skills Youll Need
10-3
21
Areas of Regular Polygons
GEOMETRY LESSON 10-3
10-3
22
Areas of Regular Polygons
GEOMETRY LESSON 10-3
10-3
23
Areas of Regular Polygons
GEOMETRY LESSON 10-3
A portion of a regular hexagon has an apothem
and radii drawn. Find the measure of each
numbered angle.
Quick Check
10-3
24
Areas of Regular Polygons
GEOMETRY LESSON 10-3
p ns Find the
perimeter.
p (20)(12) 240 Substitute 20 for n and 12 for
s.
A 4548 Simplify.
The area of the polygon is 4548 in.2
Quick Check
10-3
25
Areas of Regular Polygons
GEOMETRY LESSON 10-3
10-3
26
Areas of Regular Polygons
GEOMETRY LESSON 10-3
(continued)
Step 2 Find the perimeter p. p ns Find the
perimeter. p (8)(18.0) 144 Substitute 8 for
n and 18.0 for s, and simplify.
10-3
27
Areas of Regular Polygons
GEOMETRY LESSON 10-3
(continued)
To the nearest 10 ft2, the area is 1560 ft2.
Quick Check
10-3
28
Areas of Regular Polygons
GEOMETRY LESSON 10-3
1. Find m 1. 2. Find m 2. 3. Find m
3. 4. Find the area of a regular 9-sided figure
with a 9.6-cm apothem and 7-cm side. For
Exercises 5 and 6, find the area of each regular
polygon. Leave your answer in simplest radical
form. 5. 6.
Use the portion of the regular decagon for
Exercises 13.
36
18
72
302.4 cm2
10-3
29
Perimeters and Areas of Similar Figures
GEOMETRY LESSON 10-4
(For help, go to Lesson 1-9.)
Find the perimeter and area of each figure.
1. 2. 3. Find the perimeter and area of
each rectangle with the given base and
height. 4. b 1 cm, h 3 cm 5. b 2 cm, h
6 cm 6. b 3 cm, h 9 cm
Check Skills Youll Need
10-4
30
Perimeters and Areas of Similar Figures
GEOMETRY LESSON 10-4
10-4
31
Perimeters and Areas of Similar Figures
GEOMETRY LESSON 10-4
4. The perimeter is the sum of the sides 1 3
1 3 8 cm the area is the product of the
base b and the height h A bh (1)(3) 3
cm2 5. The perimeter is the sum of the sides 2
6 2 6 16 cm the area is the product of
the base b and height h A bh (2)(6) 12
cm2 6. The perimeter is the sum of the sides 3
9 3 9 24 cm the area is the product of
the base b and height h A bh (3)(9) 27 cm2
Solutions (continued)
10-4
32
Perimeters and Areas of Similar Figures
GEOMETRY LESSON 10-4
The triangles below are similar. Find the ratio
(larger to smaller) of their perimeters and of
their areas.
The shortest side of the triangle to the left has
length 4, and the shortest side of the triangle
to the right has length 5.
Quick Check
10-4
33
Perimeters and Areas of Similar Figures
GEOMETRY LESSON 10-4
The ratio of the lengths of the corresponding
sides of two regular octagons is . The area of
the larger octagon is 320 ft2. Find the area of
the smaller octagon.
8 3
All regular octagons are similar.
The area of the smaller octagon is 45 ft2.
Quick Check
10-4
34
Perimeters and Areas of Similar Figures
GEOMETRY LESSON 10-4
The similarity ratio of the fields is 3.5 1, so
the ratio of the areas of the fields is (3.5)2
(1)2, or 12.25 1.
Because seeding the smaller field costs 8,
seeding 12.25 times as much land costs 12.25(8).
Seeding the larger field costs 98.
Quick Check
10-4
35
Perimeters and Areas of Similar Figures
GEOMETRY LESSON 10-4
The areas of two similar pentagons are 32 in.2
and 72 in.2 What is their similarity ratio? What
is the ratio of their perimeters?
The similarity ratio is 2 3. By the Perimeters
and Areas of Similar Figures Theorem, the ratio
of the perimeters is also 2 3.
Quick Check
10-4
36
Perimeters and Areas of Similar Figures
GEOMETRY LESSON 10-4
1. For the similar rectangles, give the ratios
(smaller to larger) of the perimeters and of the
areas. 2. The triangles are similar. The area
of the largertriangle is 48 ft2. Find the area
of the smaller triangle. 3. The similarity ratio
of two regular octagons is 5 9. The area of the
smaller octagon is 100 in.2 Find the area of the
larger octagon. 4. The areas of two equilateral
triangles are 27 yd2 and 75 yd2. Find their
similarity ratio and the ratio of their
perimeters. 5. Mulch to cover an 8-ft by 16-ft
rectangular garden costs 48. At the same rate,
what would be the cost of mulch to cover a 12-ft
by 24-ft rectangular garden?
27 ft2
324 in.2
3 5 3 5
108
10-4
37
Trigonometry and Area
GEOMETRY LESSON 10-5
(For help, go to Lesson 10-3.)
Find the area of each regular polygon.
1.
3.
2.
Check Skills Youll Need
10-5
38
Trigonometry and Area
GEOMETRY LESSON 10-5
Solutions
10-5
39
Trigonometry and Area
GEOMETRY LESSON 10-5
Find the area of a regular polygon with 10 sides
and side length 12 cm.
Because the polygon has 10 sides and each side is
12 cm long, p 10 12 120 cm.
Use trigonometry to find a.
10-5
40
Trigonometry and Area
GEOMETRY LESSON 10-5
(continued)
Now substitute into the area formula.
The area is about 1108 cm2.
Quick Check
10-5
41
Trigonometry and Area
GEOMETRY LESSON 10-5
The radius of a garden in the shape of a regular
pentagon is 18 feet. Find the area of the garden.
10-5
42
Trigonometry and Area
GEOMETRY LESSON 10-5
(continued)
So p 5 (2 AM) 10 AM 10 18(sin 36)
180(sin 36).
10-5
43
Trigonometry and Area
GEOMETRY LESSON 10-5
(continued)
1 2
Finally, substitute into the area formula A
ap.
The area of the garden is about 770 ft2.
Quick Check
10-5
44
Trigonometry and Area
GEOMETRY LESSON 10-5
Use Theorem 9-1 The area of a triangle is one
half the product of the lengths of two sides and
the sine of the included angle.
Quick Check
The area of the park is approximately 27,200 ft2.
10-5
45
Trigonometry and Area
GEOMETRY LESSON 10-5
Find the area of each figure. Give answers to the
nearest unit.
1. regular hexagon with perimeter 90
ft 2. regular pentagon with radius 12
m 3. regular polygon with 12 sides of length 1
in.
585 ft2
342 m2
11 in2
4.
5.
490 mm2
70 yd2
10-5
46
Circles and Arcs
GEOMETRY LESSON 10-6
(For help, go to Lesson 1-9 and Skills Handbook,
page 761.)
1. r 7 cm, d 2. r 1.6 m, d 3. d
10 ft, r 4. d 5 in., r 5. 9 of
360 6. 38 of 360 7. 50 of 360 8. 21 of 360
Find the diameter or radius of each circle.
Round to the nearest whole number.
Check Skills Youll Need
10-6
47
Circles and Arcs
GEOMETRY LESSON 10-6
Solutions
1. The diameter is twice the radius (7)(2) 14
cm 2. The diameter is twice the radius (1.6)(2)
3.2 m 3. The radius is half the diameter 10
2 5 ft 4. The radius is half the diameter 5
2 2.5 in. 5. 9 0.09. Read of as times, so 9
of 360 is 0.09 times 360. (0.09)(360)
32.4. The nearest whole number to 32.4 is 32. 6.
38 0.38. Read of as times, so 38 of 360 is
0.38 times 360. (0.38)(360) 136.8. The
nearest whole number to 136.8 is 137. 7. 50
0.50. Read of as times, so 50 of 360 is 0.50
times 360. (0.50)(360) 180. 8. 21 0.21.
Read of as times, so 21 of 360 is 0.21 times
360. (0.21)(360) 75.6. The nearest whole
number to 75.6 is 76.
10-6
48
Circles and Arcs
GEOMETRY LESSON 10-6
Because there are 360 in a circle, multiply
each percent by 360 to find the measure of each
central angle.
65 25 of 360 0.25 360 90
4564 40 of 260 0.4 360 144
2544 27 of 360 0.27 360 97.2
Under 25 8 of 360 0.08 360 28.8
Quick Check
10-6
49
Circles and Arcs
GEOMETRY LESSON 10-6
Quick Check
10-6
50
Circles and Arcs
GEOMETRY LESSON 10-6
Quick Check
10-6
51
Circles and Arcs
GEOMETRY LESSON 10-6
The pool and the fence are concentric circles.
The diameter of the pool is 16 ft, so the
diameter of the fence is 16 4 4 24 ft.
Use the formula for the circumference of a
circle to find the length of fencing material
needed.
Quick Check
About 76 ft of fencing material is needed.
10-6
52
Circles and Arcs
GEOMETRY LESSON 10-6
Quick Check
10-6
53
Circles and Arcs
GEOMETRY LESSON 10-6
100.8
A major arc is greater than a semicircle. A minor
arc is smaller than a semicircle.
30
270
10-6
54
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
(For help, go to Lesson 10-6.)
1. What is the radius of a circle with diameter 9
cm? 2. What is the diameter of a circle with
radius 8 ft? 3. Find the circumference of a
circle with diameter 12 in. 4. Find the
circumference of a circle with radius 3 m.
Check Skills Youll Need
10-7
55
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
Solutions
1. The radius is half the diameter 9 2 4.5
cm 2. The diameter is twice the radius (8)(2)
16 ft 3. C d (12) 12 , or about
37.7 in. 4. C 2 r 2 (3) 6 , or
about 18.8 m
10-7
56
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
Find the areas of the archery target and the
bulls-eye.
10-7
57
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
The area of the yellow region is about 424 in.2
Quick Check
10-7
58
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
.
.
Quick Check
10-7
59
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
Step 1 Find the area of sector AOB.
10-7
60
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
10-7
61
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
To the nearest tenth, the area of the shaded
segment is 353.8 ft2.
Quick Check
10-7
62
Areas of Circles and Sectors
GEOMETRY LESSON 10-7
1571 m2
15 cm2
138 in.2
10-7
63
Geometric Probability
GEOMETRY LESSON 10-8
(For help, go to the Skills Handbook, pages 756
and 762.)
Check Skills Youll Need
10-8
64
Geometric Probability
GEOMETRY LESSON 10-8
Solutions
1. BD 5 2 3 AE 9 0 10
2. CE 9 4 5 AF 10 0 10
3. AB 2 0 2 BC 4 2 2
1 4. The area of the smaller circle is
r2 (1)2 m The area of the larger
circle is r2 (2)2 4 m smaller
larger 4 1 4, or . 5. 4 is
one of six numbers on the number cube. So, the
probability is 1 out of 6 chances or .
BD AE
3 9
1 3
CE AF
5 10
1 2
AB BC
2 2
1 4
1 6
10-8
65
Geometric Probability
GEOMETRY LESSON 10-8
Solutions (continued)
6. The numbers on a number cube are 1, 2, 3, 4,
5, and 6. Three numbers, 1, 3, and 5, are
odd. 3 out of 6 numbers are odd, so the
probability is 3 out of 6 or . 7.
There are 6 numbers on a number cube. The numbers
2 and 5 are two of them. So, 2 out of 6
numbers are desired. The probability is 2 out of
6 or . 8. The numbers on a number cube
are 1, 2, 3, 4, 5, and 6. The numbers 2, 3,
and 5 are prime. So 3 out of 6 numbers are prime.
The probability is 3 out of 6 or .
3 6
1 2
2 6
1 3
3 6
1 2
10-8
66
Geometric Probability
GEOMETRY LESSON 10-8
The length of the segment between 2 and 10 is 10
2 8.
The length of the ruler is 12.
Quick Check
10-8
67
Geometric Probability
GEOMETRY LESSON 10-8
Quick Check
Because the favorable time is given in minutes,
write 1 hour as 60 minutes. Benny may have to
wait anywhere between 0 minutes and 60 minutes.
Starting at 60 minutes, go back 15 minutes. The
segment of length 45 represents Bennys waiting
more than 15 minutes.
10-8
68
Geometric Probability
GEOMETRY LESSON 10-8
Find the area of the square. A s2 202 400
cm2
10-8
69
Geometric Probability
GEOMETRY LESSON 10-8
The probability that a dart landing randomly in
the square does not land within the circle is
about 21.5.
Quick Check
10-8
70
Geometric Probability
GEOMETRY LESSON 10-8
To win a prize, you must toss a quarter so that
it lands entirely within the outer region of the
circle below. Find the probability that this
happens with a quarter of radius in. Assume
that the quarter is equally likely to land
anywhere completely inside the large circle.
15 32
10-8
71
Geometric Probability
GEOMETRY LESSON 10-8
(continued)
15 32
Find the area of the circle with a radius of 9
in. A r2 (9 )2 281.66648 in.2
15 32
10-8
72
Geometric Probability
GEOMETRY LESSON 10-8
The probability that the quarter lands entirely
within the outer region of the circle is about
0.326, or 32.6.
Quick Check
10-8
73
Geometric Probability
GEOMETRY LESSON 10-8
1. A point on AF is chosen at random. What is the
probability that it is a point on BE?
2. Express elevators to the top of a tall
building leave the ground floor every 40
seconds. What is the probability that a
person would have to wait more than 30 seconds
for an express elevator? A dart you throw is
equally likely to land at any point on each
board shown. For Exercises 35, find the
probability of its landing in the shaded
area. 3. regular octagon 4. square 5. circle
3 5
1 4
10-8