Composites

1
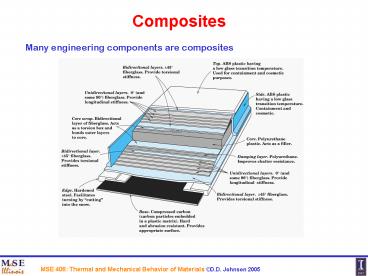
Composites
Many engineering components are composites
2
Composites
ISSUES TO ADDRESS...
What are the classes and types of composites?
Why are composites used instead of metals,
ceramics, or polymers?
How do we estimate composite stiffness
strength?
What are some typical applications?
3
Classification of Composites
Composites - Multiphase material
w/significant proportions of ea. phase.
Matrix - The continuous phase -
Purpose is to transfer stress to other
phases protect phases from environment
- Classification MMC, CMC, PMC
metal
ceramic
polymer
Dispersed phase -Purpose enhance matrix
properties. MMC increase sy, TS, creep
resist. CMC increase Kc PMC
increase E, sy, TS, creep resist.
-Classification Particle, fiber, structural
From D. Hull and T.W. Clyne, An Intro to
Composite Materials, 2nd ed., Cambridge
University Press, New York, 1996, Fig. 3.6, p. 47.
4
COMPOSITE SURVEY Particle-I
Particle-reinforced
Examples
Adapted from Fig. 10.10, Callister 6e.
Adapted from Fig. 16.4, Callister 6e.
Adapted from Fig. 16.5, Callister 6e.
5
COMPOSITE SURVEY Particle-II
Particle-reinforced
Elastic modulus, Ec, of composites -- two
approaches.
From Fig. 16.3, Callister 6e.
Application to other properties --
Electrical conductivity, se Replace E by se.
-- Thermal conductivity, k Replace E by k.
6
COMPOSITE SURVEY Fiber-I
Fiber-reinforced
Aligned Continuous fibers
Ex
--Metal g'(Ni3Al)-a(Mo) by eutectic
solidification.
--Glass w/SiC fibers formed by glass slurry
Eglass 76GPa ESiC 400GPa.
(a)
From F.L. Matthews and R.L. Rawlings, Composite
Materials Engineering and Science, Reprint ed.,
CRC Press, Boca Raton, FL, 2000. (a) Fig. 4.22,
p. 145 (photo by J. Davies) (b) Fig. 11.20, p.
349 (micrograph by H.S. Kim, P.S. Rodgers, and
R.D. Rawlings).
(b)
From W. Funk and E. Blank, Creep deformation of
Ni3Al-Mo in-situ composites", Metall. Trans. A
Vol. 19(4), pp. 987-998, 1988.
7
COMPOSITE SURVEY Fiber-II
Fiber-reinforced
Discontinuous, random 2D fibers
Example Carbon-Carbon --process
fiber/pitch, then burn out at up to
2500C. --uses disk brakes, gas
turbine exhaust flaps, nose cones.
(b)
(a)
Other variations --Discontinuous, random
3D --Discontinuous, 1D
8
Chapter 6 Elasticity of Composites

• Stress-strain response depends on properties of
• reinforcing and matrix materials (carbon,
  polymer, metal, ceramic)
• volume fractions of reinforcing and matrix
  materials
• orientation of fibre reinforcement (golf club,
  kevlar jacket)
• size and dispersion of particle reinforcement
  (concrete)
• absolute length of fibres, etc.

9
Families of Composites particle, fibre,
structural reinforcements
Twisting, Bending
ceramics
Orientation dependence
10
Two simplest cases Iso-load and Iso-strain
Isostrain Load Reinforcements
Aligned Isoload Load Reinforcements
Perpendicular (Isostress below)
Strain or elongation of matrix and fibres are the
same!
Volume fraction
F
Load (Stress) across matrix and fibres is the
same!
F
11
Iso-strain Case in Ideal Composites
Isostrain Case
strain
forces
Load is distributed over matrix and fibers, so
?cAc ?mAm ?fAf.
if the fibers are continuous, then volume
fraction is easy.
For Elastic case
Composite Property
like law of mixtures
Properties include elastic moduli, density, heat
capacity, thermal expansion, specific
heat, ...
12
Consider Density, Heat Capacity, and Thermal
Expansion
For Elastic case
Composite Property
like law of mixtures
density, heat capacity, thermal
expansion,
How?
Need to assess the proper dependence of the
properity to get Rule-of-Mixture correct.
13
Iso-Load Case for Ideal Composites
Isoload Case
strain
forces
Without de-bonding, loads are equal, therefore,
strains must add, so
if the fibers are continuous or planar, then
area of applied stress is the same.
elastic case
Composite Property
like resistors in parallel.
Properties include elastic moduli, density, heat
capacity, thermal expansion, specific
heat, ...
14
ISOSTRAIN Example Suppose a polymer matrix (E
2.5 GPa) has 33 fibre reinforcements of glass (E
76 GPa). What is Elastic Modulus?
26.7 GPA
25 GPA
Stiffness of composite under isostrain is
dominated by fibres.
15
ISOLOAD Example Suppose a polymer matrix (E 2.5
GPa) has 33 fibre reinforcements of glass (E
76 GPa). What is Elastic Modulus?
Rearrange
3.8 GPA
Elastic modulus of composite under isoload
condition Strongly depends on stiffness of
matrix, unlike isostrain case where stiffness
dominates from fibres.
16
Modulus of Elasticity in Tungsten Particle
Reinforced Copper
isostrain
isoload

• Particle reinforcements usually fall in between
  two extremes.

17
Simplified Examples of Composites
Are these isostrain or isoload? What are some
real life examples?
18
COMPOSITE SURVEY Fiber-III
Fiber-reinforced
Critical fiber length for effective stiffening
strengthening
fiber strength in tension
fiber diameter
shear strength of fiber-matrix interface
Ex For fiberglass, fiber length gt 15mm needed
Why? Longer fibers carry stress more
efficiently!
Shorter, thicker fiber
Longer, thinner fiber
Adapted from Fig. 16.7, Callister 6e.
Better fiber efficiency
Poorer fiber efficiency
19
COMPOSITE SURVEY Fiber-IV
Fiber-reinforced
Estimate of Ec and TS --valid when
-- Elastic modulus in fiber direction
--TS in fiber direction
efficiency factor --aligned 1D K 1
(anisotropic) --random 2D K 3/8 (2D
isotropy) --random 3D K 1/5 (3D isotropy)
Values from Table 16.3, Callister 6e.
(aligned 1D)
20
COMPOSITE SURVEY Structural
Structural
Stacked and bonded fiber-reinforced sheets
-- stacking sequence e.g., 0/90 -- benefit
balanced, in-plane stiffness
Adapted from Fig. 16.16, Callister 6e.
Sandwich panels -- low density, honeycomb
core -- benefit small weight, large bending
stiffness
Adapted from Fig. 16.17, Callister 6e.
21
Composite Benefits
CMCs Increased toughness
PMCs Increased E/r
MMCs Increased creep
resistance
Adapted from T.G. Nieh, "Creep rupture of a
silicon-carbide reinforced aluminum composite",
Metall. Trans. A Vol. 15(1), pp. 139-146, 1984.
22
Laminate Composite (Ideal) Example
Gluing together these composite layers composed
of epoxy matrix (Em 5 GPa) with graphite fibres
(Ef 490 GPa and Vf 0.3). Central layer is
oriented 900 from other two layers.

• Case I - Load is applied parallel to fibres in
  outer two sheets.
• Case II - Load is applied parallel to fibres of
  central sheet.
• What are effective elastic moduli in the two
  case?
• First need to know how individual sheets
  respond, then average.

For isoload case.
For isotrain case.
Case I Elam(2/3)(150.5 GPa) (1/3)(7.1 GPa)
102.7 GPa
Case II Elam(1/3)(150.5 GPa) (2/3)(7.1 GPa)
54.9 GPa
23
Mechanical Response of Laminate is Complex and
NOT Ideal

• 3 Conditions required consider top and bottom
  before laminated
• strain compatibility- top and bottom must have
  same strain when glued.
• stress-strain relations - need Hookes Law and
  Poisson effect.
• equilibrium - forces and torques, or twisting
  and bending.

Isostrain for load along x-dir
Poisson Effect and Displacements in D

• When glued together displacements have to be
  same!
• Unequal displacements not allowed!
• So, top gets wider (sytop gt 0) and bottom gets
  narrower (sybott lt 0).
• Equilibrium Fy 0 (sybot tbot sytop
  ttop)L. (t thickness)

24
COMPATIBILITY When glued, displacements have to
be same!
As stress is applied, compatibility can be
maintained, depending on the laminate, only if
materials twists.
25
Symmetry of laminate composite dictates properties
Elastic constants are different for different
symmetry laminates.
26
Orientation of layers dictates response to
stresses
Want compressive stresses at end of laminate so
there are no tensile stresses to cause
delamination - failure!
27
NO delamination - failure!
Apply in-pane Tensile Stress A B 90 45 45 45
45 90 45 90 45 45 90 45 Tensile -gt
delaminate Compressive
28
Why Laminate Composite is NOT Ideal
Depending on placement of load and the
orientation of fibres internal to sheet and the
orientation of sheets relative to one another,
the response is then very different. Examples of
orientations of laminated sheets that provided
compressive stresses at edges of composite and
also tensile stresses there. gtgtgtgt Tensile
stresses lead to delamination! The stacking of
composite sheets and their angular
orientation can be used to prevent twisting
moments but allow bending moments. This is
very useful for airplane wings, golf club
shafts (to prevent slices or hooks), tennis
rackets, etc., where power or lift comes or is
not reduced from bending.
29
Thermal Stresses in Composites

• Not just due to fabrication, rather also due to
  thermal expansion differences between matrix and
  reinforcements ?Tm and ?Tr.
• Thermal coatings, e.g.
• Material with most contraction (least) has
  positive (negative) residual stress. (For
  non-ceramics, you should consider plastic strain
  too.)
• Ceramic-oxide thermal layers, e.g. on gas
  turbine engines
• ceramic coating ZrO2-based (lower ?Tr)
• metal blade (NixCo1-x)CrAlY (higher ?Tm)
• Failure by delamination without a good design of
  composite, i.e. compatibility maintained.

If compatible, composite will bend and rotate
At T1
At T2
30
Summary
Composites are classified according to --
the matrix material (CMC, MMC, PMC) -- the
reinforcement geometry (particles, fibers,
layers). Composites enhance matrix
properties -- MMC enhance sy, TS, creep
performance -- CMC enhance Kc -- PMC
enhance E, sy, TS, creep performance
Particulate-reinforced -- Elastic modulus
can be estimated. -- Properties are
isotropic. Fiber-reinforced -- Elastic
modulus and TS can be estimated along fiber dir.
-- Properties can be isotropic or
anisotropic. Structural -- Based on
build-up of sandwiches in layered form.