THEOREM - PowerPoint PPT Presentation
1 / 16
Title:
THEOREM
Description:
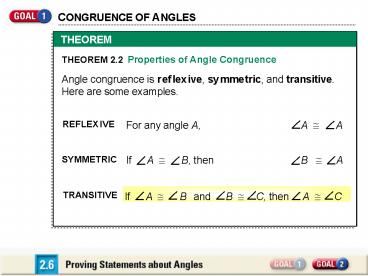
Angle congruence is r ef lex ive, sy mme tric, and transitive. Here ... If A B and B C, then A C. SYMMETRIC. If A B, then B A. REFLEX IVE. For any angle A, A A ... – PowerPoint PPT presentation
Number of Views:102
Avg rating:3.0/5.0
Title: THEOREM
1
THEOREM
THEOREM 2.2 Properties of Angle Congruence
Angle congruence is r ef lex ive, sy mme tric,
and transitive. Here are some examples.
2
Prove the Transitive Property of Congruence for
angles.
SOLUTION
To prove the Transitive Property of Congruence
for angles, begin by drawing three congruent
angles. Label the vertices as A, B, and C.
3
(No Transcript)
4
This two-column proof uses the Transitive
Property.
5
THEOREM
THEOREM 2.3 Right Angle Congruence Theorem
All right angles are congruent.
You can prove Theorem 2.3 as shown.
6
(No Transcript)
7
PROPERTIES OF SPECIAL PAIRS OF ANGLES
THEOREMS
THEOREM 2.4 Congruent Supplements Theorem
If two angles are supplementary to the same angle
(or to congruent angles) then they are congruent.
2
1
3
8
PROPERTIES OF SPECIAL PAIRS OF ANGLES
THEOREMS
THEOREM 2.4 Congruent Supplements Theorem
If two angles are supplementary to the same angle
(or to congruent angles) then they are congruent.
then
9
PROPERTIES OF SPECIAL PAIRS OF ANGLES
THEOREMS
THEOREM 2.5 Congruent Complements Theorem
If two angles are complementary to the same angle
(or to congruent angles) then the two angles are
congruent.
5
6
4
10
PROPERTIES OF SPECIAL PAIRS OF ANGLES
THEOREMS
THEOREM 2.5 Congruent Complements Theorem
If two angles are complementary to the same angle
(or to congruent angles) then the two angles are
congruent.
then
11
1 and 2 are supplements
GIVEN
3 and 4 are supplements
1 4
2 3
PROVE
12
1 and 2 are supplements
GIVEN
3 and 4 are supplements
1 4
2 3
PROVE
Statements
Reasons
13
1 and 2 are supplements
GIVEN
3 and 4 are supplements
1 4
2 3
PROVE
Statements
Reasons
14
PROPERTIES OF SPECIAL PAIRS OF ANGLES
POSTULATE
POSTULATE 12 Linear Pair Postulate
If two angles for m a linear pair, then they are
supplementary.
15
THEOREM
THEOREM 2.6 Vertical Angles Theorem
Vertical angles are congruent
1 3, 2 4
16