solve exponential

1
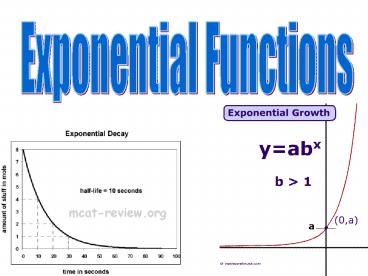
Exponential Functions
2

• Exponential Functions
• An initial amount is repeatedly multiplied by the
  same
• positive number

• Exponential equation A function of the form
  y abx
• a is the initial amount, a 0, the y
  intercept
• where b gt 0 and b 1 , is the amount we
  multiply repeatedly
• exponential growth b gt 1
• exponential decay 0 lt b lt 1
• The independent variable will be in the
  exponent

3
Exponential Growth
you can plot the points on a graph.
Draw in the curve that fits the plotted points.
x y 3x y
-2 3-2 1/9
-1 3-1 1/3
0 30 1
1 31 3
2 32 9
4
With exponential growth, the dependent variable
starts off growing slowly, but, the bigger it
gets.the faster it grows. Eventually an
exponential function will always increase more
than another type of function.
5
Eventually an exponential function will always
increase more than another type of function.
6
Exponential Decay
you can plot the points on a graph.
Draw in the curve that fits the plotted points.
x y(1/3)x y
-2 (1/3)-2 9
-1 (1/3)-1 3
0 (1/3)0 1
1 (1/3)1 1/3
2 (1/3)2 1/9
7
With exponential decay, the dependent variable
starts off decaying (getting smaller)
quickly, but, the smaller it gets. the less it
decays
8
Any quantity that grows or decays by a fixed
percent at regular intervals is said to possess
exponential growth or exponential decay.
9
Where Are They Used?

• Mortgage loans (home economics)
• Student loans (home economics)
• Population growth (government)
• Bacteria or virus growth (science)
• Depreciation on a vehicle (home economics)
• Investment accounts (home economics)
• Carbon dating (science)

10
Exponential Growth Functions
If a quantity increases by the same proportion r
in each unit of time, then the quantity displays
exponential growth and can be modeled by the
equation

• Where
• C initial amount
• r growth rate (percent written as a decimal)
• t of time intervals
• (1r) growth factor

11
Example Interest

• You deposit 1500 in an account that pays 2.3
  interest yearly,
• What was the initial principal (P) invested?
• What is the growth rate (r)? Take the of
  yearly change and divide it by 100 to change it
  to a decimal?
• Using the equation A C(1r)t, how much money
  would you have after 4 years if you didnt
  deposit any more money?

1. The initial amount (C) is 1500.

2) The growth rate (r) is 0.023.
3) The growth factor is 1.023

• t is 4 years, the number of times
• the interest is applied

12
Exponential Decay Functions
If a quantity decreases by the same proportion r
in each unit of time, then the quantity displays
exponential decay and can be modeled by the
equation

• Where
• C initial amount
• r growth rate (percent written as a decimal)
• t of time intervals
• (1 - r) decay factor

13
Example Exponential Decay

• You buy a new car for 22,500. The car
  depreciates at the rate of 7 per year,
• What was the initial amount invested?
• What is the decay rate? The decay factor?
• What will the car be worth after the first year?
  The 10th year?

1. The initial amount (C) is 22,500.

2) The decay rate (r) is 0.07.
3) The decay factor is .93

• t is 10 years, the number of times
• the value is reduced

14
All of the transformations that you learned apply
to functions work on exponential functions , so
what would the graph of
look like?
up 3
down 1
right 2
Reflected over x axis
up 1
15
Transformations Involving Exponential Functions
16
The Natural Base e
An irrational number, symbolized by the letter e,
appears as the base in many applied exponential
functions. This irrational number is
approximately equal to 2.72. The number e is
called the natural base. The function f (x) ex
is called the natural exponential function.
-1
17
The Number e
e 2.718 281 828 459
p
e
4-2-40
18
Exponential Growth and Decay
Description Equation Graph
Uses
Short-term population growth (people, bacteria,
etc.) growth of money at continuous compound
interest Radioactive decay light
absorption in water, glass, etc. atmospheric
pressure electric circuits
y cektc, k gt 0 y cektc, k gt 0
Unlimited growth Exponential decay
4-2-42-1
19
Exponential Growth and Decay
Description Equation Graph
Uses
y c(1 ekt )c, k gt 0
Learning skills sales fads company growth
electric circuits Long-term population
growth epidemics sales of new products
company growth
Limited growth Logistic growth
4-2-42-2
20
Formulas for Compound Interest

• After t years, the balance, A, in an account
  with principal P and annual interest rate r (in
  decimal form) is given by the following formulas
• For n compoundings per year
• For continuous compounding A Pert.

21
Example
You want to invest 8000 for 6 years your bank
pays 7 per year, compounded monthly.
Solution We use the compound interest model with
P 8000, r 7 0.07, n 12 (monthly
compounding, means 12 compoundings per year), and
t 6.
The balance in this account after 6 years is
12,160.84.
22
Example

• Use A Pert to solve the following problem
• Find the accumulated value of an investment of
  2000 for 8 years at an interest rate of 7 if
  the money is compounded continuously
• Solution
• A PertA 2000e(.07)(8)A 2000 e(.56)A
  2000 1.75A 3500