Particle Physics and Cosmology

1
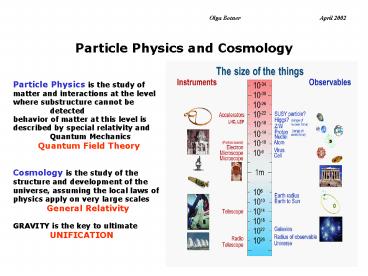
Particle Physics and Cosmology
Olga Botner April 2002

• Particle Physics is the study of
• matter and interactions at the level
• where substructure cannot be
• detected
• behavior of matter at this level is described by
  special relativity and
• Quantum Mechanics
• Quantum Field Theory
• Cosmology is the study of the structure and
  development of the universe, assuming the local
  laws of
• physics apply on very large scales
• General Relativity
• GRAVITY is the key to ultimate
• UNIFICATION

2
Olga Botner April 2002
Particle Physics and Cosmology
Today we understand the physics back to the time
about 1011 s after Big Bang hot soup of
quarks and gluons at a temperature about 1015
K beyond that speculation
3
(No Transcript)
4
(No Transcript)
5
The Standard Model
Olga Botner April 2002

• Matter constituents Quarks and Leptons
• spin ½ (fermions)
• 3 families (generations)

• Force carriers
• spin 1 (bosons)

For each generation
3 stands for number of colors
Today quarks appear only in bound systems -
property of QCD confinement Only color neutral
systems hadrons are directly
observable Quarks observed by shaking hadrons
behave as free if observed during short
time intervals asymptotic freedom
For the entire matter sector necessary for
meaningful electroweak unification
6
Fundamental charges and symmetries
Olga Botner April 2002
electric charge / weak flavour / color charge
weak isospin / weak hypercharge
Applies for each generation and for each quark
color
The conservation of the electric charge follows
from the invariance of the Lagrangian under a
certain type of transformation gauge
transformation. The phase of the electron wave
fct cannot be measured reflects global
gauge symmetry
The SM also assumes conservation of
7
(No Transcript)
8
History of the Universe
Olga Botner April 2002
Phase transition at 1012 K color is hidden
(hadron formation)
mesons
baryons
Some hadrons p, n constitute ordinary
matter Others p, K abundant in cosmic rays
Bound quark systems can be excited !
Ex. a process important for understanding of the
propagation of cosmic rays in the universe
For processes on CMBR get limit on the range of
protons of 100 Mpc (2 of the distance to the
edge of the universe)
9
Olga Botner April 2002
Language of particle physics Quantum Field
Theory - with the interaction Lagrangian as
fundamental ingredient
matter field
Ex. electron
electromagnetic field
Passage of a charged particle nearby produces
disturbances in the e.m. field
Feynman B continually emits carriers of the
electromagnetic force photons A absorbs the
photons and recoils repulsive force In QFT both
signs of impulse are possible
In the SM interaction via particle exchange 12
kinds of force carriers (gauge particles)
10
QED is the archetypical QFT
Olga Botner April 2002
A gauge theory has a hidden
degree-offreedom reflected in the Lagrangian
and the equations of the motion Viz. freedom to
locally define the phase of the
wavefunction Local gauge invariance
Global phase invariance - obvious Local phase
invariance - at the cost of a new long-range
field It can be shown that this new field must
represent a massless particle- the photon see
ex. Martin, Shaw App. C
11
Non-relativistic free electron
Olga Botner April 2002
Schrödinger
Dt
Dx
Covariant derivatives
12
Relativistic free electron
Olga Botner April 2002
Dirac
Dt
Dx
Covariant derivatives
13
QCD
Olga Botner April 2002
Color charges act as sources of strong
interaction Hidden degree of freedom symmetry
under SU(3) The three colors are equivalent.
Demand invariance under local gauge
transformations
Where the F1 F8 are 8 independent color
charge operators (may be represented by 3x3
matrices)
Need 8 long range fields to ensure local gauge
invariance g1 g8
14
Weak interaction
Olga Botner April 2002
Possible to formulate a theory based on
equivalence of the weak flavours like e and
ne Weak isospin symmetry under SU(2)
Demand invariance under local gauge
transformations
Where the I1 and I2 are operators
interchanging e and ne while I3 projects out the
weak isospin quantum numbers
Need 3 long range fields to ensure local gauge
invariance W , W , W0
15
Problems
Olga Botner April 2002

• gauge particles expected massless
• wrong ratio of neutral currents to charged
  currents
• infinite predictions for ordinary processes
  (like ee- -gt m m-)

Remedy
Glashow Salam - Weinberg
Unification condition
group
weak hypercharge YW Q IW3 B0
Physical particles
16
The Electroweak Lagrangian
Olga Botner April 2002
fermions
gauge particles
Higgs
etc
17
Higgs mechanism
Olga Botner April 2002
Gauge theories as described require massless
particles! (to ensure renormalizability) but
Force carriers ( gauge particles) mass range
0 100 GeV Matter constituents mass range 0.5
175 GeV
Higgs 1964 , EnglertBrout 1964 , Kibble 1967
The quantum properties of the system are
obviously wrong Must have left out an essential
piece of the Lagrangian Introduce a new
field! Mathematical game
The Lagrangian for a scalar field
where
Forget about physics
18
Spontaneous symmetry breaking
Olga Botner April 2002
The Higgs Kibble mechanism
The system posesses rotational symmetry. The
equilibrium state does not!
determined
but not the phase!
In the SM an isodoublet of scalar fields 4
degrees-of-freedom Higgs predicts 1 observable
scalar particle MH2 2 m2
1971 tHooft prooves renormalizability of the
e.w. QFT when gauge symmetry spontaneously broken
19
The electroweak Lagrangian
Olga Botner April 2002
after spontaneous symmetry breaking
H is the Higgs field
Dirac
Charged currents
W are the massive charged weak boson fields
Electromagnetic
A is the massless photon field
Neutral currents
Z is the massive neutral weak boson field
20
Where is the Higgs particle ?
Olga Botner April 2002

• The Higgs mechanism is just the simplest
  conjecture
• predicts existence of particle but not its
  exact mass
• predicts mass generation by interaction with
• the Higgs field for fermions gHff
  proportional to mf

Data favour an elementary scalar of mass lt few
hundred GeV
From LEP mH gt 114 GeV (direct searches) mH lt
222 GeV at 95 C.L.
Will the Higgs be found at the LHC ? mH gt
order(1 TeV) has implications for the scattering
of longitudinally polarized W and Z
21
Grand Unified Theories
Olga Botner April 2002

• The SM provides an exceedingly accurate
  description of strong,
• electromagnetic and weak interactions up to
  energies 200 GeV
• Examples
• mZ 91.1874 (21) GeV cannot be improved with
  any
• of the envisaged future machines
• number of light neutrinos Nn 2.9841 (83)
• not the final truth
• strong and electroweak interactions not unified
• gravity left out
• about 20 free parameters
• no viable dark matter candidate

Successful e.w. unification encourages attempts
to incorporate SM into a more global symmetry -
GUT
22
Grand Unified Theories (cont)
Olga Botner April 2002

• In QFT couplings are not constant,
• but depend on energy (and particle
• content of the theory)
• running couplings
• GUTs are inspired by the merging of
• the couplings to one value at the GUT scale
• 1015 1016 GeV
• (provided desert between 102 and 1015)

• GUTs have quarks and leptons in common multiplets
• predict
• interactions converting quarks into leptons and
  vice versa
• new gauge particles (12 in SU(5))
• non conservation of baryon number (proton decay)
• proton charge electron charge

SU(5) GUT fails
23
(No Transcript)
24
Supersymmetry
Olga Botner April 2002
Gauge hierarchy problem!! Simple theory leads to
instabilities in the gauge particle masses the
characteristic energy of the SM (MEW 100 GeV) is
much smaller than MGUT 1016 GeV Expect quantum
mechanical corrections to move MEW close to MGUT
Supersymmetry (SUSY) in the TeV range solves the
hierarchy problem! Remove instabilities by
introducing new particles Each SM particle gets a
partner with spin differing by ½ unit
With SUSY infinities cancel
25
Supersymmetry (cont)
Olga Botner April 2002
In many SUSY models a conserved quantum number
R-parity
Minimal supersymmetry

• If R-parity conserved
• s-particles are produced in pairs
• eventually decay to the lightest
• SUSY particle (LSP)
• the LSP is stable and weakly
• interacting (if neutral)
• a perfect dark matter candidate

The cosmologically interesting models usually
have the LSP as the neutralino higgsino for high
masses, gaugino for lower masses. Accumulation
in heavenly bodies.
There are hints for SUSY but no evidence
yet! Presumably s-particles are very heavy and
SUSY is a broken symmetry
26
Superstrings
Olga Botner April 2002

• Unification of gravity with the other
  interactions
• The holy grail of theorists
• Progress during past 2 decades
• replace particles with strings to avoid
  gravitational interactions at a point
• natural string length scale LPl 1033 cm
• theory of relativistic strings has to be
  formulated in 10 dimensions
• (or more) to be renormalizable
• the 6 extra space dimensions must curl up within
  
• a tiny geometrical space (LPl)

1st superstring revolution 1984 1985 five
different superstring theories 2nd
superstring revolution 1994 - ??? the five
SSTs are in fact five different
perturbation expansions of a single
underlying theory
M theory magic , mystery , meta , membrane ,
mother
27
Superstrings (cont)
Olga Botner April 2002
Local form of supersymmetry forces the
introduction of a spin 2 field with a spin 3/2
superpartner
28
Outside space-time
Olga Botner April 2002
A theory of quantum gravity is needed to reach
higher densities, shorter scales or earlier
instants of cosmic time
The cosmic clock started at the Planck time
10 43 s when the extra dimensions compactified
Prior to that ????
Understanding of black holes is a key issue .
Reconnecting structure of strings and membranes
Hawking, Penrose, Rees, tHooft, Silk, Seiberg .
What did God do before He created the Universe ?
He was preparing Hell for people who might ask
such questions! (attributed to St. Augustine)