Quadratic Equations

1
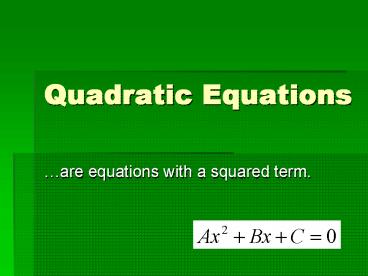
Quadratic Equations

• are equations with a squared term.

2
Quadratic Equations
Quadratic Term
Linear Term
Constant
3
Quadratic Equations

• 6 Methods to solve

• Graphing
• Factoring
• Square Root
• Completing the Square
• Quadratic Formula
• Calculator Program

4
Quadratic Equations

• Graphing Method

The graph is called a PARABOLA.
How do these two equations differ?
5
Quadratic Equations
The graph is called a PARABOLA.
At what value(s) of x is the y value 0 ?
6
Example 2-1a
From the table and the graph, we can see that
the zeroes of the function are 1 and 4.
Answer The solutions of the equation are 1 and
4.
7
Example 2-1b
Answer
3 and 1
8
Example 2-2a
Notice that the graph has only one x-intercept,
2.
Answer The equations only solution is 2.
9
Example 2-2b
Answer 3
10
Example 2-3a
11
Example 2-3a
Notice that the graph has no x-intercepts. This
means that the original equation has no real
solution.
Answer It is not possible for two numbers to
have a sum of 4 and a product of 5.
Examine Try finding the product of several
numbers whose sum is 4.
12
Example 2-4a
13
Example 2-4a
The x-intercepts of the graph are between 0 and 1
and between 5 and 6.
Answer One solution is between 0 and 1 and the
other is between 5 and 6.
14
Example 2-4b
Answer between 0 and 1 and between 3 and 4
15
Example 2-5a
16
Example 2-5a
17
Example 2-5a
Answer The positive zero of the function is
approximately 8. It should take about 8 seconds
for the marble to reach the surface of the water.
18
Example 2-5b
19
Example 2-5b
Answer about 7 seconds
20
Solving Quadratic Equations by Factoring

• Get ZERO on one side by itself.
• Factor. Consider Common Factors FIRST!
• Set each factor 0.
• Solve each part.

21
Example 3-1a
Answer The solution set is 0, 4.
22
Example 3-1a
23
Example 3-1b
Answer 0, 3
24
Example 3-2a
Answer The solution set is 3.
25
Example 3-2a