Lecture 4 MR: 2D Projection Reconstruction, 2D FT

1
Lecture 4 MR 2D Projection Reconstruction, 2D FT
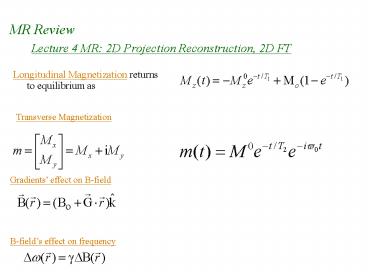
MR Review

• Longitudinal Magnetization returns to equilibrium
  as

Transverse Magnetization
Gradients effect on B-field
B-fields effect on frequency
2
MR Review (2)

• Signal Equation

Signal equation related to k-space
The MR signal is always telling us a point of the
frequency domain expression for M, the Fourier
Transform of m(x,y), the proton density of the
image. The mapping between s(t) and the points
in k-space is determined by the gradient
waveforms.
3
Timing Diagrams
RF
time over which data acquisition occurs
constant gradient
t
Gx
t0
t3
t1
t2
Object is a square box of water

• Gx Readout gradient
• Gy Phase-encoding gradient
• Gz Slice-encoding
• (or slice-selective) gradient

S(t)
Receiver signal
4
Timing Diagrams Time related to position in
k-space
RF
Gx
t
Object is a square box of water
Signal from t1 to t3 is the F.T. of the
projection at angle 0, formed by the line
integrals along y
Signal
5
Timing Diagrams Time related to position in
k-space
RF
Gx
t
Object is a square box of water
Signal from t1 to t3 is the F.T. of the
projection at angle 0, formed by the line
integrals along y
Signal
(Slide repeated without animation)
6
What gradient(s) are playing?
Can you determine the Gx(t) and Gy(t) waveforms?
9PHFRENC.AVI
7
Timing Diagrams Time related to position in
k-space (2)
90
RF
t
Gy
t1
Gx

• At t1, , we are at this
  point in k-space.

ky
kx
8
2D Projection Reconstruction (2D PR) Single-sided
90
t
RF
where
G cos(?)
t
Gx
G sin(?)
t
Gy
t
DAQ
Data Acquisition
? is considered in radians/G here. 4257 Hz/G
often used also
ky

• Repeat at various ?

?
kx
Called single side measurement.
9
2D Projection Reconstruction (2D PR)Double-sided
Called double sided measurement.
ky
t
RF
kx
t
Gx
t
Gy
Called double-sided measurement.
t
DAQ

• Reconstruction convolution back projection or
  filtered back projection

10
Object Domain

• Central Section Theorem

In MR, S(t) gives a radial line in k-space.
y
y
x
F.T.
x
F.T.
x
Interesting - Time signal gives spatial frequency
information of m(x,y)
11
2D Fourier Transform (4) 2 sided

• By far, the 2 sided 2D FT is the most popular.

Readout or frequency-encode gradient (stays the
same)
t
Gx
Phase-encode gradient (varies)
t
Gy
of steps 128-512
In practice, I(x,y) is complex-valued. Displayed
image is I(x,y), not ReI(x,y) Theoretically
is a real image Practically
has a phase due to imperfect, inhomogenous B0
field
12
2D Fourier Transform

• 2D Fourier Transform (2D FT or Spin Warp)
  1-Sided
• 1)

t
RF
ky
t
Gx
kx
t
Gy
t
DAQ
13
2D Fourier Transform (2)

• 2D Fourier Transform (2D FT or Spin Warp)
• 1)

t
RF
t
Gx
ky
kx
t
Gy
Reconstruction 2D FFT
14
2D Fourier Transform (3)

• Lets revisit the object domain -

gives a projection of
y
x
ky
kx
Modified Central Slice Theorem
15
Review Phase Encoding
Consider the 64 x 8 box to the right. A series
of MR experiments as described above were
performed. To simplify visualization, a 1D FFT
was done on each experiment. The results are
shown on the bottom where each row is a separate
experiment with a different Y direction phase
weighting.
ky
kx
16
2D Fourier Transform (2)

• 2D Fourier Transform (2D FT or Spin Warp)
• 1)

t
RF
6GRADECH.AVI
Gx(t)