RateEquation Approach to FM Mode Locking

1
Rate-Equation Approach to FM Mode Locking
Nicholas G. Usechak and Govind P. Agrawal
The Institute of Optics, University of Rochester,
Rochester, New York 14627
and Laboratory for Laser Energetics, Rochester,
New York 14623
CLEO, May 24, 2005
2
We have developed a quasi-analytic approach for
investigating pulses in mode-locked lasers by
introducing rate equations

• Mode-locked lasers are time consuming to model
  parametric studies are not convenient.
• Rate equations are derived by applying the moment
  method1 to the master equation of mode locking.
• These equations allow us to access the pulse
  parameters (energy, timing, frequency shift,
  chirp, and width).
• Using this approach, accurate parametric studies
  can be performed over large parameter spaces in
  fractions of a second.
• The rate-equation approach represents a general
  treatment of this problem its results collapse
  to those of previous theories.

1S.N.Vlasov, et al., Radiophys.Quantum Electron.
14 1062 (1971).
http//www.optics.rochester.edu/users/noodles
3
Since closed form solutions do not exist for the
equation governing mode-locking, prior theories
had to ignore effects
Previous analytic efforts investigated AM
mode-locked lasers using

• Taylor expansion of the modulation cycle and ?2
  ?3 ? 02
• Autosoliton theory3 (assumes the pulse is fixed
  by the cavity elements)
• Soliton perturbation theory4 (sought to include
  the modulator but ?3 0)

Using the moment method we gain

• The ability to include third-order dispersion and
  the modulation depth
• A better understanding of pulse stability in FM
  mode-locked fiber lasers
• The ability to investigate operation in the
  normal dispersion regime
• Fast results

2D. Kuizenga and A. Siegman, IEEE J. Quantum
Electron. QE-6, 694 (1970).
3H. Haus and Y. Silberberg, IEEE J. Quantum
Electron. QE-22, 325 (1986).
4F. Kärtner, D. Kopf, and U. Keller, J. Opt. Soc.
Am. B 12, 486 (1995).
4
The moment method allows a pulses moments to be
monitored as it propagates under the influence
of a governing equation
We focus on pulse energy, timing, frequency
shift, chirp, and width
Energy
Timing
Frequency shift
Chirp
Width
Autosoliton
Gaussian
5
The moments are differentiated with respect to
the propagation variable T to give the rate
equations for FM mode locking
In these equations, constants Cn 1 and the
functions ?n depend on the pulse shape assumed
when applying the moment method
6
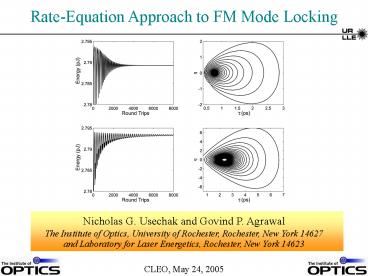
Solving this system of equations using realistic
laser parameters shows how the laser approaches
its steady state
Anomalous dispersion regime
Normal dispersion regime
Pulses are found to converge faster in the
anomalous dispersion regime. In the normal
dispersion regime, the modulators pulse forming
effect must overcome both dispersion and
nonlinearity.
7
Solving this system of equations using realistic
laser parameters shows how the laser approaches
its steady state
Anomalous dispersion regime
Normal dispersion regime
Pulses are found to converge faster in the
anomalous dispersion regime. In the normal
dispersion regime, the modulators pulse forming
effect must overcome both dispersion and
nonlinearity.
8
The real power of this method, however, is its
ability to accurately predict the steady-state
pulse parameters
By comparing this approach with full simulations,
good agreement is found in both dispersion
regimes. The results from prior theories are
shown for comparison.
Anomalous dispersion regime
Normal dispersion regime
Our work reproduces the other results shown above
in the appropriate limits.
9
Parametric studies may be performed very quickly
(ltlt 1 s)while yielding accurate values for the
steady-state pulses
For example, the effect of varying the residual
cavity dispersion on the pulse width, chirp,
timing, and frequency shift is easily
investigated.
Anomalous dispersion regime
Normal dispersion regime
10
Parametric studies may be performed with respect
to any laser parameter
This figure shows that the pulse chirp in an FM
mode-locked laser is independent of nonlinearity
Anomalous dispersion regime
Normal dispersion regime
11
Parametric studies over multiple parameters are
not feasible using the full simulations here,
they are easily carried out
The black spot marks the parameters used to
perform all of the prior (non-parametric) results
in this presentation.
Anomalous dispersion regime
12
By focusing on the pulse timing and
frequency-shift equations, we are able to map out
the stable/unstable operating locations
FM mode-locked lasers are prone to a switching
instability where the pulses may align themselves
with either modulator extrema.
Anomalous dispersion regime
13
We have developed a quasi-analytic approach for
investigating pulses in mode-locked lasers by
introducing rate equations

• Mode-locked lasers are time consuming to model
  parametric studies are not convenient.
• Rate equations are derived by applying the moment
  method1 to the master equation of mode locking.
• These equations allow us to access the pulse
  parameters (energy, timing, frequency shift,
  chirp, and width).
• Using this approach, accurate parametric studies
  can be performed over large parameter spaces in
  fractions of a second.
• The rate-equation approach represents a general
  treatment of this problem its results collapse
  to those of previous theories.

http//www.optics.rochester.edu/users/noodles
14
All numerical simulations and analytic
investigations in this work were based on the
master equation of mode-locking
2nd order dispersion and gain filtering
3rd order dispersion
SPM
propagation
Extra Slide
Extra Slide
Loss and saturated gain
FM Mode-Locker
Where
and
The Master equation of mode-locking is written in
terms of two different time scales one with
respect to the local pulse time t and another
with respect to the round trip time scale T. The
parameters are also averaged over the cavity.