Confidence limits of normal distribution

1
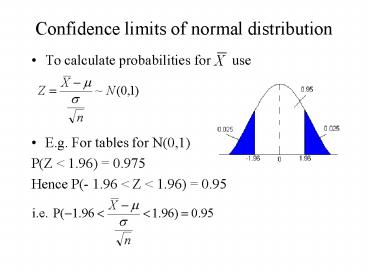
Confidence limits of normal distribution

• To calculate probabilities for use
• E.g. For tables for N(0,1)
• P(Z lt 1.96) 0.975
• Hence P(- 1.96 lt Z lt 1.96) 0.95

2
95 Confidence interval (C.I) for ?

• 95 C.I. Interval containing ?, from data
• Rearrange this to obtain 95 C.I. for µ.

3
Obtaining the C.I.
Rearrange with ? in middle. Doesnt change
probability.
4
Interpretation of C.I.

• The probability that µ lies in the random
  interval
• µ does not belong to
• µ is not a r.v.
• In repeated sampling, 95 of constructed
  intervals

5
(No Transcript)
6
Health survey example

• Sample of n 100 women aged 25-29 years sample
  mean 165 cms, sample s.d. s 5 cms
• Pretend that ? s 5 cms
• 95 confidence limits for population mean, µ are
• 165 165.98 ?166
• 165 - 164.02 ?164
• i.e. 95 C.I. for µ is (164,166) cms

7
?? unknown
If ?? unknown, then we replace ?? by s

• Z follows the Students- T distribution with
  (n-1) d.f.
• In n is small, then using
• insufficient coverage.

8
(No Transcript)
9
C.I. with ?? unknown

• Proper formula for 95 C.I. .

• Other C.I. can be similarly constructed

10
Inference for binary data

• Population of individuals classified into one of
  two categories
• 1. manufactured items defective, OK
• 2. experimental animals dead, alive
• 3. exam results pass, fail etc.
• In general, we call these "success" and
  "failure".
• We want to arrive at conclusions about p, the
  proportion of successes in the population, using
  information from a sample.
• DataR. S. of n individuals.
• X successes in sample

11
Model for binary data

• X Bin(n,p) , E(X) np and var(X) npq

12
Binomial and Normal Compared

• For large n (say n gt 20) and p not too near 0 or
  1 (say 0.05 lt p lt 0.95) the Bin(n,p)
  distribution approximately follows the Normal
  distribution with mean ? and variance ?
  
• This can be used to find binomial probabilities.

13
Sampling distribution of

• X Bin(n,p) can be approximated by the Normal
• X is approx. N(np,npq), provided ngt20 and npgt5
• Hence the sampling distribution of is

• This gives us a basis for making inferences about
  p e.g. constructing confidence intervals.

14
Confidence Intervals for Proportions

• To find a 95 CI for p, we first need to find c
  such that Pr (-c lt Z lt c) 0.95. We know c1.96

• Rearranging terms, we get the 95 C.I. as

15
Example of C.I. for proportions

• Opinion poll to predict outcome of referendum
• r.s. of 552 people asked "will you vote for ...?
• Answers recorded as either "yes" or "other
• 239 people say "yes. Find a 95 C.I. for the
  true population proportion of "yes" votes

16
C.I. as a function of sample size

• E.g. womens heights n 100,
• 95 C.I.

165 1.96

• if n 40, 95 C.I. 165 1.96

• (other things remaining the same)
• e.g. width of 95 confidence interval U - L

• n can be chosen to give a C.I. of desired
  accuracy

17
Sampling distribution of s2

• What is its sampling distribution ?

• Sums of squares of i.i.d normals are chi-squared
  with as many d.f. as there are terms.

18
(No Transcript)
19
C.I. forVariance

• Sampling distribution