Increasing Decreasing Functions

1
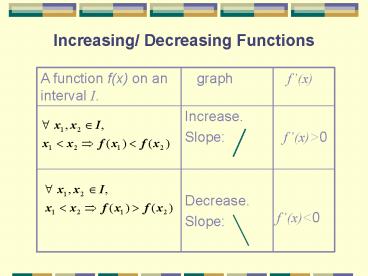
Increasing/ Decreasing Functions
2
First Derivative Test

• Let f continuous on a,b and differentiable
  on(a,b)
• Example

f(x)
R
- - - - - -

0
2
4
f(x)
increase decrease
3
Maxima and Minima

• If f is continuous on a closed interval I, then
  f have maximum and minimum values in I.
• maximum - global
• Extreme - local
• minimum - global
• -
  local
• Let domain( f ) D, and c ?D
• - f(c) is a global (absolute) minimum if
• - f(c) is a global max. if

4
Candidates for global extrema point

• 1. Critical Points
• Stationary points points where f(x)0.
• Singular points points where f(x) does not
  exists (sharp curve, jumps, infinite grad.)
• 2. Interval boundary.
• Steps finding extrema points
• Determine the candidates
• Evaluate f at those points
• Select the largest(max.) and the smallest(min.)
  value.

5
Example

• Find the max and min values of
• Solution Either max occurs at an end point x 0
  or x 2 or at a point in (0,2) which is a
  critical point.

6
Higher Derivatives

• The second derivative of yf(x) with respect to x
  
• We say y is twice differentiable at x, and
  written

7
Second Derivative Test for a Local Max/Min at xc

• If f(c ) 0 and f(c ) lt 0, then local max at
  x c.
• If f(c ) 0 and f(c ) gt 0, then local min at
  x c.
• If f(c ) 0 f(c ) the 2nd derivative test
  fails anything can happen.

8
Examples

• Find all local max and min of

9
(No Transcript)
10
Graph sketching

• Definition If or as
  or
• . The line xa is called a vertical
  asymptote.
• Definition If as or as
• then the line yl is a
  horizontal asymptote.

11

• If on an interval I, the slope
  increases and yf(x) called concave up on I.
• If on an interval I, the slope
  decreases and yf(x) called concave down on I.
• If on I then f(x) increases on I.
• If on I then f(x) decreases on I.

12
When sketching graphs of yf(x)

• Find points where f(x) is not defined
• Find where x0, y0, where ygt0 ylt0.
• Find asymptotes
• Local max and local min, where f(x) increases and
  decreases.

13
Example

• Sketch
• - Undefined at x ?1

14

• By 1st derivative test, local max at x 0 and
  f(0) -1.
• By 2nd derivative test