Direct Variation

1
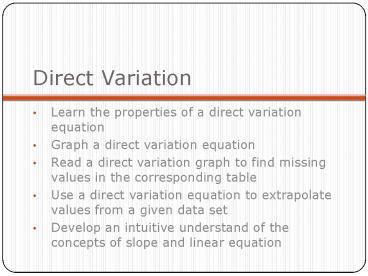
Direct Variation

• Learn the properties of a direct variation
  equation
• Graph a direct variation equation
• Read a direct variation graph to find missing
  values in the corresponding table
• Use a direct variation equation to extrapolate
  values from a given data set
• Develop an intuitive understand of the concepts
  of slope and linear equation

2
Direct Variation

• Page 114
• Materials Needed
• Graph Paper
• Graphing Calculators

3
Ship Canals

• In this investigation you will look at data about
  canals to draw a graph and write an equation that
  states the relationship between miles and
  kilometers. Youll see several ways of finding
  the information that is missing from the table.
• Complete steps 1 2 of the investigation

4
(No Transcript)
5

• Complete step 3 by entering the data in your
  graphing calculator.
• Turn to Calculator Note 1F if you need help
  entering the data in the graphing calculator.
• Complete steps 4 to find the ratio L2L1. See
  Calculator Note 1K to create this list. Write
  your answer to this step on your Communicator.
• Complete step 5 of the investigation. Show how
  your determined your answer on the Communicator.

6

• How can you change x miles to y kilometers?
  Using variables, write an equation to show how
  miles and kilometers are related.
• Use the equation you wrote in the last step to
  find the length in kilometers of the Suez Canal
  and the length in miles of the Trollhatte Canal.
  How is using this equation like using a rate?
• Graph the equation on your calculator. Compare
  this graph to your hand-drawn graph. Why does
  the graph go through the origin?
• Trace the graph of your equation. Approximate
  the length in kilometers of the Suez Canal by
  finding when x is approximately 101 miles. Trace
  the graph to approximate the length in miles of
  the Trollhatte Canal. How do these answers
  compare to the one you got from your hand-drawn
  graph?

7

• Use the calculators table to find the missing
  lengths for the Suez Canal and the Trollhatte
  Canal.
• In this investigation you used several ways to
  find missing information Approximating with a
  graph, calculating with a rate, solving an
  equation, and searching a table. Write several
  sentences explaining which of these methods you
  prefer and why.
• Since the ratio was the same for every pair of
  points, we say that kilometers and miles are
  directly proportional.
• The relationship between kilometers and miles is
  called a direct variation.
• It follows the form y kx where k is a constant
  of variation.

8

• Study the example on page 116
• Use the graphing calculator for parts c and d
• Then complete problem 5 on page 118.