Review: Cauchy Sequences

1
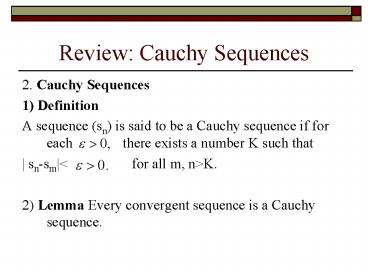
Review Cauchy Sequences

• 2. Cauchy Sequences
• 1) Definition
• A sequence (sn) is said to be a Cauchy sequence
  if for each there exists a number K
  such that
• sn-smlt for all m, ngtK.
• 2) Lemma Every convergent sequence is a Cauchy
  sequence.

2
More Lemma

• Lemma Every Cauchy sequence is bounded.

3
Cauchy Convergent Criterion

• Theorem A sequence is convergent iff it is a
  Cauchy sequence.

4
Homework

• 18.3 a), c), d), 4, 5 a), 6 a), 7, 10 a), 13
• 3 c) and 13 are due Wednesday (11/16)
• Exam 2 Monday (11/21)
• Coverage 3.13, 3.14, 4.16, 4.17, 4.18, 4.19

5
4.19 Subsequences

• Subsequences
• 1) Definition
• Let (sn) be a sequence and let (nk) be any
  sequence of positive integers such that
• n1ltn2ltn3lt .
• The sequence (snk) is called a subsequence of
  (sn)

6

• 2) Examples
• (sn)(n2)(1, 4, 9, .)
• (s2k-1)((2k-1)2)(1, 9, 25, .)
• (s2k)((2k)2)( 4, 16, 36, .)
• (s2k-1) and (s2k) are subsequences.

7

• 3). Theorems
• If a sequence (sn) converges to a real number s,
  then every subsequence of (sn) also converges to
  s.
• Proof Let (snk) be any subsequence of (sn).
• Given any
• Since lim sns, there exists a number L such that
  
• sn-smlt for all ngtL.
• When kgtL, nkgtL. So snk -slt for
  all kgtL.
• This proves that (snk) converges to s.

8

• b) Every bounded sequence has a convergent
  subsequence.
• Proof Let (sn) be a bounded sequence. Then its
  range Ssn n is a natural number is bounded.
• Case 1. If S is finite, then there is some number
  x in S that is equal to sn for infinitely many
  values of n. That is, there exist indices
• n1ltn2ltn3lt .
• such that snkx for all k. So (snk) converges
  to x.

9

• Case 2. If S is infinite, then it follows from
  Bolzano-Weierstrass Theorem that S has an
  accumulation point y. We prove there is a
  subsequence that converges to y.
• For each positive integer k, let
• Nk(y-1/k, y1/k).
• Since y is an accumulation point of S, for each
  k, there are infinitely many sn in Nk.So we can
  pick sn1in N1, sn2in N2, , snkin Nk, ..
• Such that n1ltn2ltn3lt ..

10

• Now we have a subsequence (snk) for which
• snk-ylt1/k.
• It follows that snk converges to y.

11

• c). Every unbounded sequence contains a monotone
  subsequence that diverges to either positive
  infinity or negative infinity.
• Proof Suppose that (sn) is unbounded. Then it
  either unbounded above or below.
• Assume it is unbounded below. We shall construct
  an unbounded decreasing subsequence of (sn) .
• Let M1-1. Then there exists sn1 such that
  sn1lt-1
• Let M2min-2, sn1 . Then there exists sn2 such

12

• that sn2ltM2. Then sn2lt sn1 and, sn2lt -2.
• Continue this process, inductively, we can
  construct a subsequence (snk) such that
• . ltsn2 ltsn1
• and snklt -k.
• So (snk) is unbounded decreasing therefore
  diverges to negative infinity.

13
Limit Superior and Limit Inferior

• 2. Limit Superior and Limit Inferior
• Definition
• Let (sn) be a bounded sequence. A subsequential
  limit of (sn) is any real number that is the
  limit of some subsequence of (sn) . If S is the
  set of all subsequential limits of (sn), then we
  define the limit superior (upper limit) of (sn)
  to be supremum of S, and denote as

14

• lim sup snsup S.
• Similarly, we define the limit inferior (or lower
  limit) to be the infimum of S, which is denoted
  as
• lim inf sninf S.
• Question. Given a sequence (sn), do limit
  superior and limit inferior exist?

15

• 2) Example
• sn(-1)n
• Lim sup sn1, lim inf sn -1.

16

• 3) Theorem
• Let (sn) be a bounded sequence and let
• mlim sup sn. Then the following properties
  hold.
• a) For every there exists a number L
  such that
• for all ngtL.
• b) For every and every positive
  integer i there exists a positive integer kgti
  such that
• Furthermore, if m is a real number satisfying
  properties a) and b), then mlim sup sn.

17
Corollary

• 4) Corollary Let (sn) be a bounded sequence and
  let mlim sup sn. Then m is S, where S is the set
  of all subsequential limits of (sn). That is,
  there exists a subsequence of (sn) that converges
  to m.

18
Homework

• 19.1, 19.2 a, b, c, d, 19.3 a, b, , 19.4 a, b
• 19.5, 19.7 a, b