The Duration Model

1
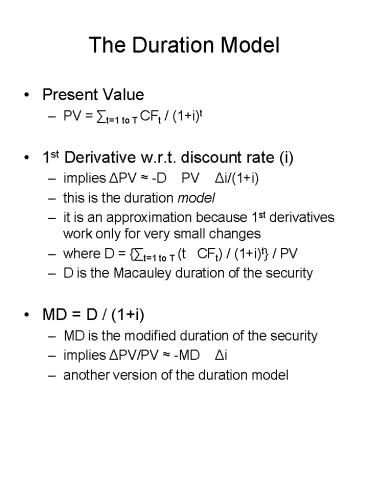
The Duration Model

• Present Value
• PV ?t1 to T CFt / (1i)t
• 1st Derivative w.r.t. discount rate (i)
• implies ?PV -D PV ?i/(1i)
• this is the duration model
• it is an approximation because 1st derivatives
  work only for very small changes
• where D ?t1 to T (t CFt) / (1i)t / PV
• D is the Macauley duration of the security
• MD D / (1i)
• MD is the modified duration of the security
• implies ?PV/PV -MD ?i
• another version of the duration model

2
Meaning(s) of Duration

• Weighted-Average Maturity
• Weights are the relative contribution to the
  total value of the security or PVcashflow/PVsecuri
  ty
• Better way of measuring (and matching) maturity
  than date of receipt of last cash flow
• Measure of Interest Rate Risk
• MD is an elasticity w.r.t. changes in the
  discount rate.
• ?PV/PV -MD ?i

3
Calculating Duration
4
Duration Model vs. Reality

• Duration is a linear approximation of a
  non-linear world.
• The approximation gets worse as the interest rate
  change grows larger
• The difference between the actual change in value
  and the duration prediction is due to convexity

5
Duration of a Portfolio

• The duration of a portfolio is the
  weighted-average duration of the individual
  securities.
• MDportfolio ?j1 to J wj MDj
• The weights equal the contribution of the
  security to the value of the portfolio
• wj PVj/PVportfolio
• As required, the weights sum to one
• ?j1 to J wj 1
• Example Portfolio with 3 securities
• 10K of Bond A (MD 6.3 years)
• 55K of Bond B (MD 2.7 years)
• 35K of Bond C (MD 0.4 years)
• weight of Bond A 10/(105535) 0.10
• MDportfolio (0.10 6.3) (0.55 2.7) (0.35
  0.4) 2.26 years

6
Convexity

• The duration model (?PV/PV -MD ?i) is only an
  approximation.
• As ?i gets larger, the error grows.
• Can we improve on this? Yes.
• ?PV/PV -MD ?i ½C ?i²
• C is the convexity of the security
• Calculated in a manner similar to MD (more
  complex formula)
• based on 2nd derivative w.r.t. discount rate

7
Convexity

• Positive Convexity
• for the typical bond, C gt 0
• gains are bigger for decrease in rates than loss
  for same increase in rates
• when Cgt0 the duration model is conservative
• actual gains and losses are less than predicted
  by duration model
• Negative Convexity
• for some securities, C lt 0
• we call this negative convexity
• BAD gains are smaller for decrease in rates than
  loss for same increase in rates
• when Clt0 the duration model underestimates losses
  and overestimates gains
• only happens when CFs change with changes in
  interest rates
• mortgage portfolios act this way

8
Convexity

• Why isnt convexity used in practice?
• Impractical
• Cannot calculate the convexity of a portfolio
  from the convexity of its individual securities.

9
Duration of Equity

• According to the balance sheet identity
• Assets Liabilities Equity
• Equity Assets Liabilities.
• Using this identity to reflect PV rather than
  Book Value
• PVequity PVassets PVliabilities
• a.k.a. the Economic Value of Equity or EVE
• Not the same as market value
• Equity is a portfolio containing positive assets
  and negative liabilities.
• MDequity (PVassets/PVequity)MDassets
  (PVliabilities/PVequity)MDliabilities

10
Duration of Equity Examples

• Example 1
• PV Assets of 500 million (MD 3.5 years)
• PV Liabilities of 450 million (MD 2.5 years)
• EVE 50 million
• MDequity (500/50)3.5 (450/50)2.5 12.50
  years.
• What happened?

11
Duration of Equity Examples

• Example 2
• PV Assets of 500 million (MD 2.5 years)
• PV Liabilities of 450 million (MD 3.5 years)
• EVE 50 million
• MDequity (500/50)2.5 (450/50)3.5 -6.50
  years.
• Negative Duration?

12
Advantages of Duration Model

• Single Number easy to interpret
• bigger riskier
• positive implies hurt by rising interest rates
• Easy to Explain
• Senior management
• Easy to Calculate
• Spreadsheet technology
• Unambiguous
• Set targets or limits
• Rough but consistent
• For small interest rate changes

13
Disadvantages of Duration Model

• Assumes Parallel Interest Rate Shocks
• All rates move by same amount
• Instantaneously and permanently
• What is short and long rates move differently?
• Linear Model
• Non-linear world cant translate convexity to
  portfolio level
• Embedded Options
• Model only considers promised cash flows (can be
  adjusted to expected cash flows)
• Does not capture changing cash flows
• Off-Balance Sheet Activities
• Fee income sources may be sensitive to changing
  market rates

14
Improvements on Duration Model

• Quasi-Assets
• solution for ignoring OBS activities
• estimate future cash flows and appropriate
  discount rate and calculate value/duration
• treat fee-based activity as an asset with
  predictable, periodic cash flows.

15
Improvements on Duration Model

• Effective duration
• used when cash flows are known to change when
  interest rates change (e.g. embedded options are
  present)
• manipulate ?PV/PV -MD ?i
• to get EMD -(?PV/PV) / ?i
• EMD is the effective (implied) modified duration
• Employ complex ( accurate) valuation model
• measure value under current interest rates
• measure value for fixed increase in interest
  rates use that ?PV to measure EMD()
• measure value for (same) fixed decrease in
  interest rates use that ?PV to get EMD(-)
• EMD average of EMD() and EMD(-)
• not precise but better than strict duration model
  which assumes cash flows dont change

16
Improvements on Duration Model

• Effective duration example
• portfolio of asset-backed securities
• value under current conditions is 5.00 million
• value if interest rates increase by 0.25 is
  estimated at 4.40 million
• EMD() -(-0.60/5.00)/0.0025 48.0
• value if interest rates decrease by 0.25 is
  estimated at 5.35 million
• EMD(-) -(0.35/5.00)/-0.0025 28.0
• EMD (48.0 28.0) / 2 38.0

17
Improvements on Duration Model

• Key Rate Duration
• solution to problem of assuming parallel interest
  rate shocks (all rates change permanently by same
  amount at same time)
• measure the sensitivity of each asset to
• short-term riskless yield
• a set of differences between the short-term
  riskless yield and riskless yields associated
  with different maturities
• An Example measure independent sensitivities to
• 3-month CMT
• 1-year CMT - 3-month CMT
• 5-year CMT - 1-year CMT
• 20-year CMT - 5-year CMT
• these should be independent of one-another
• CMT constant maturity Treasury

18
Scenario Analysis

• Requires accurate valuation model
• (1) Identify key inputs to valuation model
  economic factors
• various interest rates
• volatility of rates
• default expectations/credit spreads
• (2) Select a set of predetermined alternative
  future conditions
• example of one scenario rates rise, interest
  volatility increases, rising default/credit
  spreads
• pick other representative sets of input values
• focus on interest rates
• parallel shocks
• tilts
• twists

19
Scenario Analysis

• (3) Measure the value of the portfolio or
  institution for each scenario using the
  valuation model
• (4) Look for unacceptable losses
• identify cause(s)
• fix problems
• Advantage compared to duration
• allows more complex valuation
• considers additional sources/causes of risk
• Disadvantages compared to duration
• less clear
• cant tell if an important scenario is missing

20
Simulation Models/Analysis

• Requires accurate valuation model
• (1) Identify key inputs to valuation model
• various interest rates
• volatility of rates
• default expectations/credit spreads
• (2) Identify the probability distribution of each
  key input, including parameters
• example joint normal distribution assumption
  requires estimates of mean, standard deviation,
  and correlations for each variable
• other distributions can be more complex with more
  parameters
• (3) Randomly select a set of values for the key
  inputs to the valuation model
• based on distributional assumptions in (2)

21
Simulation Models/Analysis

• (4) Using the key input values
• for each security (face value, coupon rate,
  maturity, contractual features known), determine
  a value
• (5) Repeat (3) and (4) a lot!
• 1,000 times
• (6) Study the results of this simulation
• dont worry about gains or small losses
• big losses? ask why? how many? too many?