Four major statistical categories

1
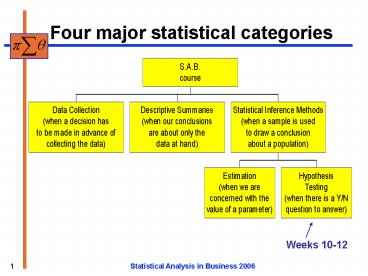
Four major statistical categories
Weeks 10-12
2
When to use a hypothesis test?

• Whenever
• a sample is used to represent a population,
• and
• the question to be investigated is about a
  parameter (or parameters), and has a yes/no
  answer.

3
Week 11 objectives

• 1. Chi-squared tests for categorical data
  general form
• 2. Tests of several proportions against a fixed
  pattern goodness-of-fit tests
• 3. The chi-squared distribution
• 4. Tests in two way contingency tables
• 5. Assumptions and Conditions
• 6. Standardised residuals

4
When is a goodness-of-fit test needed?

• In a national survey, consumers were asked the
  question, In general, how would you rate the
  level of service that businesses provide?
• The response categories were excellent, pretty
  good, only fair and poor.
• A store manager wants to find out whether the
  results of this national survey apply to
  supermarket customers in her city.
• She interviews randomly selected consumers as
  they leave supermarkets in various parts of the
  city.
• Are observed responses consistent with those
  expected on the basis of the national survey?

5
When is a chi-squared test in a contingency table
needed?

• Do men and women prefer the same colours of cars?
• Suppose a study is undertaken to address this
  question.
• A random sample of men and women are asked which
  of five colours (silver, white, black, red, blue)
  they prefer in a car.
• The results are summarised in a contingency
  table.
• Is colour preference for cars independent of
  gender?

6
Multi-parameter tests

• When a test involves several parameters, the
  confidence interval method cannot be used
• The test statistic must be some composite
  quantity
• In testing proportions, what is the test
  statistic when several proportions are being
  tested?

7
1. Goodness-of-fit tests for categorical data
The general form of Pearson's chi-squared goodness
-of-fit test statistic is where O is an
observed count and E is the corresponding
expected count, in various categories S
stands for summation over all the categories, and
Q denotes quadratic.
,
8
2. Example a test of several proportions against
a fixed pattern

• According to standard genetic theory,
• if the sex of new born babies is determined at
  random with probabilities 1/2, 1/2 for males and
  females,
• then among families having two children, the
  proportions of occurrences of 0, 1 or 2 females
  should be 1/4, 1/2, 1/4 respectively.
• Among a sample of 28 families, the counts in
  these categories were 8, 11, 9 respectively.
• The null hypothesis is the statement that the
  population proportions should be 1/4, 1/2, 1/4
  for the three categories respectively.

9
Goodness-of-fit test (continued)
10
Goodness-of-fit test (continued)

• The value of the goodness-of-fit test statistic
  is 1.357
• What is the meaning of this?
• Is the fit to the theoretical proportions
  1/41/21/4 a good fit or a poor fit?
• What is the null distribution of the test
  statistic?
• The null distribution is chi-squared

11
3. What are chi-squared distributions?

• Like t-distributions, every chi-squared
  distribution depends on a degrees of freedom
  parameter
• The mean value of a chi-squared variable is equal
  to its degrees of freedom
• Variables having chi-squared distributions are
  always non-negative, and are usually quadratic,
  ie sum of squares expressions, like sample
  variances or goodness-of-fit statistics

12
(No Transcript)
13
Finding degrees of freedom and P-value

• The degrees of freedom is k?1, where k is the
  number of proportions
• If Ho is not true, the (O-E)-squared terms
  inflate the goodness-of-fit criterion
• So the P-value has the form
• Prchi-squared variable gt observed value
• Minitab will evaluate the P-value

14
Using Minitab to find the P-value
We have k 3 categories (ie 0, 1 or 2
females),so there are k?1 2 degrees of
freedom.
Therefore the P-value will not be small. In
Minitab use Calc gt Probability distributions gt
Chi-square, then select degrees of freedom 2,
Cumulative probability option, and input
constant 1.357. Chi-Square with 2 DF
x P( X lt x) 1.3570 0.4926 The
P-value is 1 0.49 0.51.
15
Solution using the six steps
16
The six steps (contd)

• (iv) The P-value is 0.51
• Decision rule Reject the null hypothesis if
  P-value lt 0.05, but if P -value gt 0.05, then the
  null hypothesis cannot be rejected.
• In this case P-value 0.51, which is gt 0.05, so
  the null hypothesis cannot be rejected.
• (vi) Conclusion there is no evidence to suggest
  that the underlying population proportions of
  numbers of male and female children differ from
  the pattern 1/41/21/4.

17
Further comments

• The case k 2 this can be tested with
• a 2 sample proportion test, using Minitab or
• the confidence interval method, or
• by a chi-squared goodness-of-fit test.
• The three methods give identical results
• Using Minitab to carry out goodness-of-fit tests
  of proportions against a fixed pattern see
  textbook for details

18
Lecture exercise 1

• A large company engages management consultants
  who claim that for employees within the company,
  the probabilities are
• 1/3 for exceeding a set performance target,
• 1/3 for meeting the target exactly, and
• 1/3 for being below target.
• Among a sales team of 24 staff, the actual
  numbers in the three categories turn out to be 6,
  14 and 4.
• Is there evidence that the basis of the
  consultants predictions were wrong? Do steps 1
  2.

19
Solution using the six steps
(i)
(ii)
20
Calculation details
21
The six steps (cont.)

• (iii) Assumptions and conditions see later
• The P-value is about 0.030, that is found from
  the Minitab output below.
• Chi-Square with 2 DF
• P( X lt x) x 0.9000
  4.6052
• 0.9500 5.9915
• 0.9750 7.3778
• 0.9900 9.2103

22
The six steps (cont.)

• Decision rule reject the null hypothesis if
  P-value lt 0.05, but if P-value gt 0.05, then the
  null hypothesis cannot be rejected.
• In this case P-value 0.03 lt 0.05, so the null
  hypothesis is rejected.
• (vi) Conclusion there is evidence to conclude
  that the basis of the consultants predictions
  were wrong.

23
4. Chi-squared tests in two way contingency tables
A survey of clients' satisfaction levels with the
facilities and management of three sporting
facilities is based on random samples of 20
clients in each facility. The results are
summarized in the following contingency table
Is there evidence of different satisfaction
levels in the three facilities?
24
Chi-squared test of independence

• A more common chi-squared test is when patterns
  of row counts are compared for different columns
  (or column counts in different rows).
• In the example are the columns different?
• That is, we test for whether the row-variable
  influenced the column-variable, or vice versa
• This is called a test of independence of row and
  column classifications

25
Contingency table tests (cont.)
26
Using Minitab for contingency table tests
27
Minitab output
28
Solution using the six steps
29
The six steps (cont.)

• (iii) Assumptions and conditions discussed
  shortly
• (iv) The P-value is 0.33
• Decision rule reject the null hypothesis if
  P-value lt 0.05, but if P-value gt 0.05, then the
  null hypothesis cannot be rejected. Here, since
  P-value 0.33 gt 0.05, we cannot reject the null
  hypothesis
• (vi) Conclusion there is not enough statistical
  evidence to suggest any differences in
  satisfaction levels between the three sporting
  facilities

30
5. Assumptions and conditions for all chi-squared
tests

• Well-defined categorical variables
• Representative sample
• Stable population proportions
• Independence
• Large number condition all expected values
  should be gt 5

31
Assumptions and conditions in the sporting
facility example

• Well-defined categorical variables?
• Yes, either satisfaction or sporting
  facility
• Representative sample?
• OK from random sampling
• Stable population proportions?
• It would be OK if the survey is done quickly
• Independence?
• It would be OK if views are gathered in private
• Large number condition from Minitab output all E
  values are either 14.67 or 5.33, both gt 5

32
What if the large number condition fails?

• Isolated E values which are lt 5 may be forgiven,
  especially if only just lt 5
• But a large group of E values which are lt 5 may
  indicate that the large number condition fails
• Remedial action may be possible
• pool together some rows, or some columns. The E
  values will increase.
• Alternatively, simply delete some rows or some
  columns.
• Either way there will be a loss of degrees of
  freedom.
• Try to amalgamate similar rows/columns, so that
  any new combined category has some meaning

33
6. Standardised residuals
34
More on standardised residuals
35
Another example of standardized residuals
36
Lecture exercise 2

• Following large payouts to directors, a company
  surveys randomly selected stockholders about
  their opinions of the companys public relations
  image, and their size of shareholding.
• Opinions were noted favourable, neutral or
  unfavourable,
• Size was categorized as small, medium or
  large.
• Does the size of shareholding influence the
  opinion of the public relations image?

37
Data for lecture exercise 2
Does there seem to be an association between the
variables?
38
Which cells are influential in the final result?
Using Minitab output, complete the solution using
the six steps, including checking Conditions.
Answer
39
Steps (i), (ii)
40
Steps (iii) to (vi)