ThreePhase Circuit Classifications 4

1
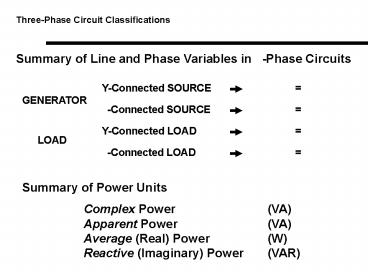
Three-Phase Circuit Classifications (4)
Summary of Line and Phase Variables in 3-Phase
Circuits
Y-Connected SOURCE

GENERATOR
-Connected SOURCE

Y-Connected LOAD

LOAD
-Connected LOAD

Summary of Power Units
Complex Power (VA) Apparent Power (VA) Average
(Real) Power (W) Reactive (Imaginary)
Power (VAR)
2
Power in a Balanced System (1)
Consider a 3-phase generator ( Y- or -
source) connected to a Y- connected load, find
the TOTAL instantaneous power at the load
What is this?
assuming a-c-b sequence
3
Power in a Balanced System (2)
Time-domain treatment
Identity
4
Power in a Balanced System (3)
Constant with time!
For Y-connected load
In terms of LINE voltage and LINE current
The same analysis can be applied to the -
connected load and this yields a similar result!
5
Power in a Balanced System (4)
Consider a 3-phase generator ( Y- or -
source) connected to a Y- connected load, find
the TOTAL COMPLEX power at the load
assuming a-b-c sequence
6
Power in a Balanced System (5)
Consider a 3-phase generator ( Y- or -
source) connected to a - connected load, find
the TOTAL COMPLEX power at the load
assuming a-b-c sequence
7
Power in a Balanced System (6)
Complex-domain treatment
Y- connected load
- connected load
Load-to-Line Conversions
Load-to-Line Conversions
8
Power in a Balanced System (7)
General results! applied to any types of
connections
Prior knowledge of the types of generator and
load is NO LONGER required!!!
9
Numerical Problem
(a)
(b)
Two balanced load are connected to a 3-phase
generator as shown creating a line voltage of
240kVrms at 60Hz. Load (1) draws 30kW at a PF of
0.6 lagging while load (2) draws 45kVAR at a PF
of 0.8 lagging. Assuming a-b-c sequence,
determine (a) complex, real and reactive powers
absorbed by the combined load (b) line
currents (c) the kVAR rating of the three
-connected capacitors C in parallel with the load
that will raise the PF to 0.9 lagging and their
capacitance
10
Chapter 2 Operational Amplifier
Operational Amplifier or OPAMP in short is a
versatile electronic unit that behaves like a
VCVS! with a very HIGH gain (gt106 typically)
Typical symbol

• 3-terminal device
• non-inverting input ()
• inverting input (-)
• output

But dont overlook supply terminals VCC and
-VCC!!
The word OPERATIONAL stems from the fact that
such device can be used to implement many kinds
of mathematical operations, such as summation,
integration, differentiation etc.
11
OPAMP Low Frequency Characteristics (1)
OPAMP is constructed from a complex arrangement
of transistors, capacitors using INTEGRATED
CIRCUIT (IC) technology!!!
Simplified Schematic of 741 OPAMP (the first
successful commercial OPAMP on the planet!
introduced in 197 xx by (Signetic)!! Corp., USA)
But we will only focus on the characteristic at
its terminals (seeing it as a block and ignore
what is going on inside!!)
12
OPAMP Low Frequency Characteristics (2)
OPAMP equivalent model at LOW FREQUENCIES
A VCVS with

• differential input resistance Ri
• output resistance Ro
• OPEN-LOOP voltage gain AOL

Typical parameter ranges
voAOLvid AOL(v-v-)
WHY requires such a high AOL! !
13
OPAMP Low Frequency Characteristics (3)
The model is valid only for the output terminal
voltages less than VCC! beyond which will cause
the device to enter saturation mode!!!
KCL i i- IVcc i-Vcc io
1. Positive saturation, vo VCC 2. Linear range,
vo lt VCC or -VCC lt AOLvid lt VCC 3. Positive
saturation, vo -VCC
We only have HIGH OPEN-LOOP gain in the linear
range!!!
14
OPAMP Low Frequency Characteristics (4)
We will only focus the use of OPAMP in the LINEAR
operation!!! This is often satisfied with the
use of NEGATIVE FEEDBACK
NEGATIVE FEEDBACK
OPAMPs output is fed back to its inverting
terminal
NEGATIVE FEEDBACK principle was invented in 1927
by H.S. Black while he was commuting to work at
Bell Laboratories on Hudson River ferry boat
The principle has been a cornerstone to the
design of amplifiers until NOW!
NEGATIVE FEEDBACK stabilises the so called
closed-loop gain AC of the amplifier
regardless of variations in the open-loop gain,
provided that AOL is sufficiently HIGH!!
15
OPAMP Low Frequency Characteristics (5)
Example
Consider an OPAMP circuit in the figure. Assuming
that the OPAMP exhibits Ri2 MOhm, RO50 Ohm.
Find the closed-loop voltage gain AC Vo/Vin
when (a) AOL 104 V/V (b) AOL 106 V/V
inverting amplifier circuit
Vo is fed back to the inverting terminal of the
OPAMP (Negative feedback)
STEP 1 Put the OPAMP model into
the circuit
16
OPAMP Low Frequency Characteristics (6)
STEP 2 Use conventional circuit analysis
technique to solve for the gain
NODE analysis
NODE A
NODE O
Solve for VO/Vin
NEGATIVE SIGN?
Thus, putting Ri2 MOhm, RO50 Ohm we have VO/Vin
-1.99994988 V/V for AOL 104 V/V VO/Vin
-1.99996972 V/V for AOL 106 V/V
LOOK how close the results!
17
OPAMP Low Frequency Characteristics (7)
WHAT actually happens? Two results are so close
due to the relatively high gain of the OPAMP
(compared to the closed loop gain) !
Lets solve the circuit again but but for the
input voltages v, v- and input current to i
and i- for the same parameters but only when
AOL104 V/V
OBSERVATIONS

• Input voltage difference Vid is SO SMALL!
• Input currents to the OPAMP is SO SMALL!

18
OPAMP Low Frequency Characteristics (8)
Vid approaches zero
HIGH open-loop gain AOL
i and i- approach zero
HIGH input resistance Ri
AOL V/V Ri Ohm RO 0 Ohm
Thus, for ideal OPAMP
Vid 0
i and i- 0
TWO golden rules for solving OPAMP circuits
(assuming operation in the linear region,
normally satisfied using NEGATIVE FEEDBACK)
1. V-V- 0
The inverting and non-inverting
terminal voltages of OPAMP are equal
2. i i- 0
There is NO current entering the inverting and
non-inverting terminals of OPAMP
19
OPAMP Low Frequency Characteristics (9)
Consider again the inverting amplifier. Well now
solve for AC using the two golden rules!
(WARNING MUST USE with CAUTION!!!)
From Rule No. 2, we know that IRFIR (with the
indicated direction)
From Rule No. 1, we know that v- v 0
vo vRF -IRFRF -IRRF
But v- 0 and hence IR vin/R
Overall!
vo/vin AC -RF/R
20
OPAMP Low Frequency Characteristics (10)
Consider the non-inverting amplifier. Lets apply
again the two golden rules to find AC vo/vin
From Rule No. 2,
From Rule No. 1,
But v- vo RF
(RF R)
Overall!
vo/vin AC
21
OPAMP Low Frequency Characteristics (11)
Exercises
From the OPAMP circuits below, solve for
(a)
vo/vin
Voltage buffer
(b)
vo/iin
Transimpedance amplifier
io/iin
(c)
Current amplifier
22
OPAMP Low Frequency Characteristics (12)
Exercises
From the OPAMP circuits below, solve for
vo/vin
(a)
Integrator
vo/vin
(b)
Differentiator
Using (a) time-domain analysis and (b) phasor
analysis
For the circuit (a), find vo(t) when vin(t) is a
pulse function as shown (assuming VC(0)0 V)
23
Cascaded OPAMP circuits
Cascaded OPAMP circuits is a HEAD-to-TAIL
arrangement of two or more OPAMP sub-circuits
such that the output of one circuit is the input
of the next
Due to the assumption that OPAMP model has ZERO
output resistance, the OPAMP sub-circuits can be
cascaded with no LOADING effect, meaning that the
cascade connection do not change the input-output
relation of the sub-blocks
Overall input-output relation is just the product
of each individual sub-block!
24
Transistors Milestones
1948
Invention of transistor (Point-contact) (The
three inventors at Bell Lab)
1953
Concept of integrated circuits was
introduced (Harwick Johnson, RCA)
1959
Planar IC technology was introduced (Robert Noyce)
1967
Intel Corporation was founded