Linear Difference Equations

1
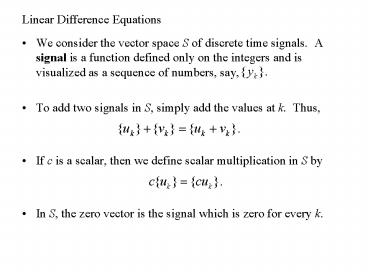
Linear Difference Equations

• We consider the vector space S of discrete time
  signals. A signal is a function defined only on
  the integers and is visualized as a sequence of
  numbers, say,
• To add two signals in S, simply add the values at
  k. Thus,
• If c is a scalar, then we define scalar
  multiplication in S by
• In S, the zero vector is the signal which is zero
  for every k.

2
Linear difference equations defined for third
order or less

• First order, where
• Second order, where
• Third order, where
• Here, is unknown and is a known
  signal. If is the zero sequence, the
  equation is homogeneous otherwise, it is
  nonhomogeneous. Once, we have obtained ,
  it is called a solution of the difference
  equation.

3
Linear Independence in S

• To simplify notation, we consider only three
  signals, but the concepts are easily generalized
  to n signals.
• Three signals,
  are linearly independent when
• Since k is arbitrary, it can be replaced by k1
  and k 2, so that
• The coefficient matrix is called the Casorati
  matrix.

4
Example for linear independence

• Example. Verify that
  are linearly independent signals. We put k
  equal 0 in the Casorati matrix
• Since the Casorati matrix is invertible for k
  0, it follows that
• In general, if the Casorati matrix is not
  invertible, the associated signals may or may not
  be independent. However, if the Casorati matrix
  is not invertible, and the associated signals are
  solutions of the same homogeneous difference
  equation, then the solutions are linearly
  dependent (see Study Guide).

5
S is infinite dimensional

• Consider the following signals
• It is readily verified that any finite set of
  these signals is linearly independent.
  Therefore, S is infinite dimensional.

k0
6
A linear mapping on the space of signals

• Let a and b be real numbers. Define the mapping
  TS?S by
• It is easily shown (Theoretical Exercise) that T
  is a linear map.
• The solutions of the homogeneous equation
• form the kernel of T. Therefore, the
  solutions form a subspace H of S.
• Theorem. The dimension of H is 2.
• Proof See next slide.

7
The dimension of H from previous slide is two

• For the second order difference equation, we may
  specify two initial values
  These initial values may be arbitrarily chosen
  from Once these values are fixed, there
  is a unique solution of the difference
  equation determined (Theorem 16 from the text,
  and also see next slide)
• Define the mapping by
  That is, map a solution
  to its values at k 0 and k 1. It is easily
  shown that the mapping F is linear. By Theorem
  16 from the text, it follows that F is
  one-to-one. In other words, F is an isomorphism.
  
• Since the space of initial values, is
  2-dimensional, it follows that H is also
  2-dimensional.

8
Existence and Uniqueness for Difference Equations

• Example. Given the Fibonacci problem
  
  generate the values of the solution for k
  2, 3,
• Simply rewrite the difference equation
  as Now, we plug in the
  initial conditions to obtain Next,
  use the values for to obtain
  and so on.
• Likewise, values of with k negative could
  also be generated.

9
Solving a homogenous equationan example

• Example. Consider the second order equation
• Assume a solution of the form Upon
  substituting this signal into the equation, we
  have
• The equation is called the
  auxiliary equation. Its roots are r 1 and r
  1. Therefore, two solutions are It is easy
  to verify that these two solutions are linearly
  independent in S. Since the solution space has
  dimension 2, these solutions span the space, and
  all solutions are linear combinations of these
  two solutions.

10
Solving a nonhomogenous equationan example

• Example. Consider the second order equation
• We guess that might work. Indeed, we
  have so that
  is a particular solution of the
  equation.
• As in Section 1.5 of the text, the general
  solution of the equation is the sum of the
  particular solution plus the general solution of
  the homogeneous equation
  If two values of are
  known, then can be determined.
  For example, if
  then

11
The Fibonacci sequence

• Consider the difference equation
• The general solution to this homogeneous equation
  is
• Upon applying the initial conditions, we obtain