The t Test for Two Independent Samples

1
The t Test for Two Independent Samples

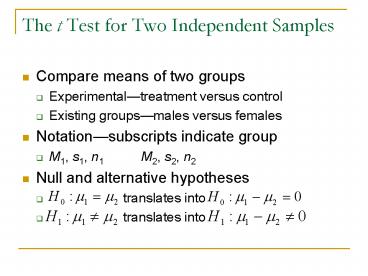
• Compare means of two groups
• Experimentaltreatment versus control
• Existing groupsmales versus females
• Notationsubscripts indicate group
• M1, s1, n1 M2, s2, n2
• Null and alternative hypotheses
• translates into
• translates into

2

• Criteria for use
• Dependent variable is quantitative,
  interval/ratio
• Independent variable between-subjects
• Independent variable has two levels
• t-test
• Basic form
• One sample

3
Two sample

• Difference between sample means M1 - M2
• Population parameter
• Sampling distribution of the difference
• Difference between M1 and M2 drawn from population

4
Standard error of the difference

• Population variance known
• Sum of
• Estimate from samples
• Differences more variable than scores

5
Variability of mean differences

• Randomly generated set of 1000 means
• ? 50, sM 10
• Take difference between pairs

6
S2pooled Pooled Variance

• Homogeneity of variance
• Assume two samples come from populations with
  equal s2s
• Two estimates of s2 and
• Weighted average

7
t-test

• df df1 df2 (n1-1) (n2-1) n1 n2 - 2

8
Hypothesis testing

• Two-tailed
• H0 µ1 µ2, µ1 - µ2 0
• H1 µ1 ? µ2, µ1 - µ2 ? 0
• One-tailed
• H0 µ1 µ2, µ1 - µ2 0
• H1 µ1 lt µ2, µ1 - µ2 lt 0
• Determine a
• Critical value of t
• df n1 n2 - 2

9
Assumptions

• Random and independent samples
• Normality
• Homogeneity of variance
• SPSStest for equality of variances, unequal
  variances t test
• t-test is robust

10
Example 1

• H0 µ1 µ2, µ1 - µ2 0
• H1 µ1 ? µ2, µ1 - µ2 ? 0
• df n1 n2 - 2 10 7 2 15
• ?.05
• t(15) 2.131

11

• t(15) 2.325, p lt .05 (precise p 0.0345)

12
Example 2

• df n1 n2 - 2 15 15 2 28
• ?.05, t(28) 2.049

13

• t(28) .947, p gt .05

14
Confidence Interval for the Difference

• Example 1
• -3.257 - (2.1311.401) lt µ1 - µ2 lt -3.257
  (2.1311.401) -6.243 lt µ1 - µ2 lt -0.272
• Example 2
• -0.867 - (1.7015.221) lt µ1 - µ2 lt -0.867
  (1.7015.221) -9.748 lt µ1 - µ2 lt 8.014
• Includes 0 retain H0

15
SPSS

• Analyze
• Compare Means
• Independent-Samples T Test
• Dependent variable(s)Test Variable(s)
• Independent variableGrouping Variable
• Define Groups
• Cut point value
• Output
• Levenes Test for Equality of Variances
• t Tests
• Equal variances assumed
• Equal variances not assumed

16
Output Example 1
17
Effect size

• Cohens d
• Example 1 Cohens d
• Example 2 Cohens d
• r2 or ?2
• G grand mean

18
(No Transcript)
19
Factors Influencing ttest and Effect Size

• Mean difference M1 M2
• Larger difference, larger t
• Larger difference, larger r2 and Cohens d

20

• Example 1, subtract 1 from first group, add 2 to
  second group
• M1 M2 increases from 3.257 to 6.257
• unaffected t increases from 2.325 to
  4.466
• r2 increases from

21

• Magnitude of sample variances
• As sample variances increase
• t decreases
• Cohens d and r2 decreases
• SSExplained unchanged, SSError and SSTotal
  increases, S2pooled increases

22

• Sample size
• Larger sample smaller t affects
• No effect on Cohens d, minimal effect on r2
• First example increase n1 from 10 to 30 and n2
  from 7 to 21