Matrix Algebra - Tutorial 6

1
Matrix Algebra - Tutorial 6
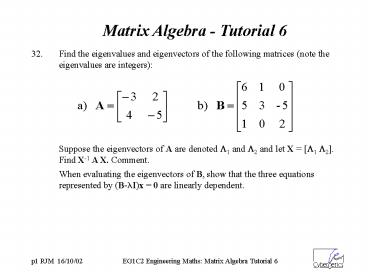
32.
Find the eigenvalues and eigenvectors of the
following matrices (note the eigenvalues are
integers)
Suppose the eigenvectors of A are denoted ?1 and
?2 and let X ?1 ?2. Find X-1 A X.
Comment. When evaluating the eigenvectors of B,
show that the three equations represented by
(B-lI)x 0 are linearly dependent.
2
33.
The Stochastic Matrix equation below shows how
the values of R, C and I change over time. R,C
and I are percentages. Use eigenvalue techniques
to show that their values will stabilise if this
equation is repeatedly applied and hence find
their steady value.
3
34.
In the rotational system above, the angular
position of the mass is q, its angular velocity
is w d?/dt, and it can be shown that -kq -Fw
Jdw/dt Express these equations as two state
equations in q and w if J 2kgm2, F 6Nm per
rad/s and k 4Nm per rad. Find the general
response of q and w and the particular response
if at time 0, w 0 rad/s and q 1 rad.
4
35.
The above circuit can be modelled by equations
Use eigenvalue techniques to find the general
response of v2 and i1 when L 4H, R 8W and C
0.25F, and the particular response if at time t
0, i1 0 and v2 2V.
5
36.
Consider the following, being a permanent magnet
armature controlled d.c. motor in a feedback loop
with controller C. The command input is 0.
Let q be the motor position and w its speed.
Question continued..
6
Examining the block diagram, the equations
describing the system are w dq/dt and Tdw/dt
-Cq - w.
Express the above in terms of the state variables
w and q and find the complex eigenvalues and
eigenvectors to the system if T 0.5s and C 5.
Hence write down the general solution to the
system. Find the particular solution if at time
t 0, ? 0 rad/s and ? 3 rad.
7
Answers
8
(No Transcript)
9
(No Transcript)