Chapter 1 Equations and Inequalities in One Variable

1
Chapter 1Equations and Inequalities in One
Variable
2
1.1 Linear and Quadratic Equations
Objective To solve linear and quadratic
equations and to understand the possible number
of solutions.
1st degree equations
The set of all numbers that make the equation
true.
Solution set -
Equivalent Equations -
Have the same solution set.
Solve 1. 5x 1 7
3. 4 5(x 2) 2x 4
3
A few more linear . . .
4
Quadratic Equations

• y ax2 bx c
• The graph is called a parabola and the solutions
  are where the graph touches the x-axis.
• How many times can a parabola cross the x-axis?
• Solutions zeros roots x-intercepts
• How to solve a quadratic ?
• Quadratic must 0
• Factor and apply the zero product property
• Quadratic Formula if it is not factorable
• Graphing (later)
• Vertex form (later)
• If c 0, factoring is the simplest method!!!!

Degree number of possible solutions.
5
Zero Product Property If (a)(b) 0, then a
0, b 0 or a b 0.
So, what does this mean?????

• x(x 3) 0 2. 5(2x 5)(x 4) 0
• 3. 2x(3x 5)(2x 7)(x 3)(5x 1)(x 9) 0

Do you see a pattern here?
6
Remember Must 0 if degree gt
1 Factor Apply the Zero Product Property
Assign 1.1 24-75 (x3), 77, 89-97 odd
7
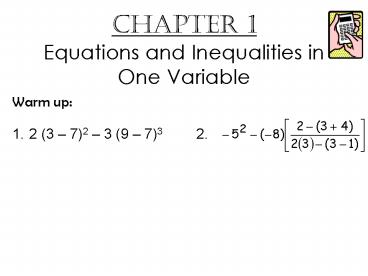
WARM-UP

• Fix at least 3 problems that you missed on the
  Chapter R test.

8
1.1 Even Answers
24. x -6, 1 30. y 2/3, 5 36. x 1, 4 42.
t -7/2, 7/2 48. x 1 54. x -2, -2/3,
2/3 60. x -1/2, 0, 1/2 66. x -2, 1/3, 2 72.
x all real numbers (What does this mean??)
???? Questions ????
9
1.2 Formulas
Objective To solve an equation for a given
variable and to use formulas to solve applications
Formula an equation that contains more than one
variable
2x - 3y 9 ? Solve the equation for y.
Find y when x 3.
1. Solve for w P 2w 2l 2. Solve for x
4x 2y c 0
10
More solving
4. ax y 3x c for x
6. A ½ bh for b
5. ax y 3x c for a
11
This formula pays interest on previously earned
interest.

• A total amount in the account

• P principal investment ( deposited)

• r annual percentage rate as a decimal

• t time in years ( 18 months _______years)

• n number of time compounded in 1 year.

Common values of n annually semi-annually
quarterly monthly
Ex How much money is earned on an investment of
1500 at 2.5 compounded quarterly for 3 years?
12
Vertical Motion
The height of an object at any given time is
given by h vt - 16t2 h height (in
feet) v initial velocity (ft/sec) t
time in seconds
Ex Elroy throws a rock into the air with and
initial velocity of 64 ft per second.
1. How high is the rock after 1 sec? 2
sec? 3 sec?
2. When will the rock reach a height of 60 feet?
3. When will the rock reach a height of 28 feet?
4. When will the rock hit the ground?
Assign Section 1 . 2
13
Lets analyze

• One way to analyze information is to examine
  charts or graphs. Lets take a look at the
  information from the vertical motion problems.
  We will summarize the information using a graph.

14
Warm up 1.4

• Percents
• What number is 5 of 28?
• 14 is what percent of 70?
• 20 is 16 of what number?

15
Answers to 1.2
24. 30. 36. 42. 48.
16
Warm-Up
Factor the following completely 1) 2) 3)
17
Honors Algebra II Section 1.4a Linear
Inequalities in One Variable
Objectives To solve and graph linear
inequalities in one variable To use
interval notation to represent the solution set
18
Inequality means not equal Symbols
Solving inequalities Inequalities are solved
with the same set of rules as equations, except
when you multiply or divide both sides by a
negative value. When that happens
you must flip the inequality sign
What does it mean to have an open circle? What
does it mean to have a closed circle? What type
of circle does ? use?
19
b) Give the set notation for the solution.
d) Give the interval notation for the solution.
20
Remember the different way of representing open
and closed circles that we mentioned earlier?
This way uses the bracket and parentheses from
interval notation. Examples
21
Assignment 1.4a - Can you solve and graph
linear inequalities in one variable? Can
you use interval notation to represent the
solution set?
22
WARM UP
23
Answers to 1.4a

• No even problems were assigned ?

Questions????
24
1.4b Solving Compound Inequalities

• Objective To solve compound inequalities and to
  continue to use interval notation

25
Recall ?and ? the intersection of the
graphs/sets overlap of the
solutions ?or ? the union of the graphs/sets
combine all solutions ? Ø empty set
null set use this when there is no
solution.
26
4 lt x lt 10 This notation is an intersection
(and). The solution set is all values between
the endpoints.
Graph and write this in interval notation.
METHOD 1 Work to undo the middle
27
NOTE smaller lt x lt larger ? (smaller, larger)
Ex 5 3x 6 lt 2x 4 or 3x 4 gt 5x
2
Can you solve compound inequalities and do you
understand interval notation?
Assign 1.4b 47-61odd, 85, 86, 87,89-93, 101-109
odd Start studying for your quiz on Wednesday.
28
Answers to 1.4b

• 86. Less than 55 minutes to keep your bill
  under 30
• 90. (x 3)(xy 4)
• 92. 2x(x 5)(x 3)

29
Quiz Summary
See summary sheet handout
30
Warm Up

• Simplify each fraction
• 1. 2. 3.

31
1.5 Absolute Value Equations
Objective To solve absolute value equations and
be able to recognize the number of possible
solutions.
What does absolute value refer to???
32
Can an absolute value equation have one solution?
HOW?
What if an absolute value equation an absolute
value equation??
33
One more!!!
??? Gives 2 solutions ?
??? Gives 1 solutions ? ??? Gives 0
solutions ?
Can you solve absolute value equations? Can you
determine the number of solutions?
Assign 1.5 3-66 (x3), 67, 68, 91, 93
34
1.5 Answers

• ? 48. 1, 8/3
• 12. -17/5, -3/5 54. Impossible or 9/2
• 18. ? 60. All real numbers
• 24. -5/3, 1 66. -7/2 or 19/6
• 30. -4/5, 2 68. 1981 and 1995
• 36. -28, 56
• 42. -5/2, -1/2

35
Warm Up
Correct each problem 1. 2.
36
1.6 Absolute Value InequalitiesObjective To
solve and graph absolute value inequalities.
In this section, we will apply the definition of
absolute value to solve absolute value
inequalities.
37
So How do we solve and graph one of these????
x 5 lt 4 becomes x 5 lt 4 and x 5 gt
-4(Note when you use the negativeflip the
inequality.) Now solve and graph.
x 5 gt 4 becomes x 5 gt 4 or x 5 lt
-4(Note when you use the negativeflip the
inequality.) Now solve and graph.
Ask Mrs. W for a real easy way to do these!!!
38
Solve and graph each absolute value
inequality.Write each in interval notation.
Can you solve and graph absolute value
inequalities?Assignment 3-66(x3), 71, 79, 80,
87, 89 This is the last section. Start your
test review NOW!!
39
1.6 Answers

• -2 lt x lt 2 48. x gt 12 or x lt 6
• ? 54. -11/4 lt x lt -9/4
• All real numbers 60. 7/12 lt x lt 11/12
• k lt 1 or k gt 4 66.
• -5/6 lt x lt 7/6 80. 1125x17y14
• (- ?, -3) ? (9, ?)
• 42. (-1, 2)

40
WARM-UP

• Take a look at your Chapter 1 Quiz. Fix at least
  2 problems that you missed on each of the 3
  pages. Remember, you may see these same types of
  questions on tomorrows TEST!!

41
Things to think about
1.1 Can you solve the 2 types of equations that
we have studied this chapter?
Linear v. Quadratic (or higher degree)
1.2 Are you comfortable solving formulas for a
designated variable?
1.4 Can you solve and graph an inequality? Put
it in interval notation?
1.5 How many equations should you set up when
solving absolute value equations? How many
answers should you expect?
1.6 Can you apply what you know about
inequalities and absolute value to solve
ABSOLUTE VALUE INEQUALITIES?