Related Rates PowerPoint PPT Presentation
Title: Related Rates
1
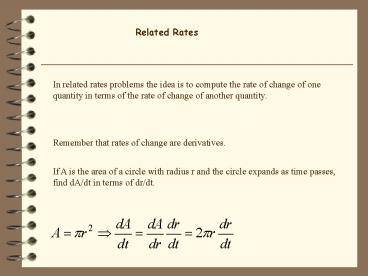
Related Rates
In related rates problems the idea is to compute
the rate of change of one quantity in terms of
the rate of change of another quantity.
Remember that rates of change are derivatives.
If A is the area of a circle with radius r and
the circle expands as time passes, find dA/dt in
terms of dr/dt.
2
Related Rates
Suppose oil spills from a ruptured tanker and
spreads in a circular pattern. If the radius of
the oil spill increases at a constant rate of 1
m/s, how fast is the area of the spill increasing
when the radius is 30m?
3
Related Rates
Strategies
1. Read the problem carefully.
2. Draw a diagram if possible.
3. Introduce notation. Assign symbols to all
quantities that are functions of time.
4. Express the given information and the required
rate in terms of derivatives.
5. Write an equation that relates the various
quantities of the problem.
6. Use the Chain Rule to differentiate both sides
of the equation with respect to t.
7. Substitute the given information into the
resulting equation and solve for the unknown rate.
4
Related Rates
Car A is traveling west at 50 mi/h and car B is
traveling north at 60 mi/h. Both are headed for
the intersection of the two roads. At what rate
are the cars approaching each other when car A is
.3 miles and car B is .4 miles from the
intersection?
A
x
C
y
z
B
5
Related Rates
6
Related Rates
7
Related Rates
Gravel is being dumped from a conveyor belt at a
rate of 30 cubic ft./min and its coarseness is
such that it firms a pile in the shape of a cone
whose base and height are always equal. How fast
is the height of the pile increasing when the
pile is 10 feet high?
8
Related Rates
9
Related Rates
10
Related Rates
PowerShow.com is a leading presentation sharing website. It has millions of presentations already uploaded and available with 1,000s more being uploaded by its users every day. Whatever your area of interest, here you’ll be able to find and view presentations you’ll love and possibly download. And, best of all, it is completely free and easy to use.
You might even have a presentation you’d like to share with others. If so, just upload it to PowerShow.com. We’ll convert it to an HTML5 slideshow that includes all the media types you’ve already added: audio, video, music, pictures, animations and transition effects. Then you can share it with your target audience as well as PowerShow.com’s millions of monthly visitors. And, again, it’s all free.
About the Developers
PowerShow.com is brought to you by CrystalGraphics, the award-winning developer and market-leading publisher of rich-media enhancement products for presentations. Our product offerings include millions of PowerPoint templates, diagrams, animated 3D characters and more.