Absolute Extrema

1
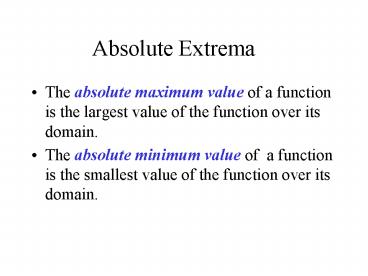
Absolute Extrema

• The absolute maximum value of a function is the
  largest value of the function over its domain.
• The absolute minimum value of a function is the
  smallest value of the function over its domain.

2
Find the absolute extrema
3
Absolute extrema of f(x) on a,b

• Find f(x) and determine all critical values
  contained in a,b.
• Evaluate f(a) and f(b).
• Evaluate f(C)
• The absolute maximum is the largest of the above
  values the absolute minimum is the smallest of
  the above values.

4
Important note

• The absolute extrema of f(x) on a,b are not
• necessarily the turning points!!

5
Find the absolute extrema

• F(x) x3 6x2 22 on -2, 6.
• F(x) x3 6x2 22 on -2, 2.
• F(x) 12 - x 9/x on (0, ?).

6
Example

• Fuel economy (in mpg) of the average
• American compact car is given by
• E(x) -.015x2 1.14x 8.3, where
• 20 lt x lt 60 mph. At what speed is fuel
• economy the greatest? What is the mpg?

7
Example

• The value of a timber forest, in dollars per
• acre, after t years is given by
• V(t) 480?t 40t, where 0 lt t lt50.
• When does forest have its maximum value?
• What is that value?

8
Example

• Daily high temperatures in NYC during
• November are modeled by
• T(x) -.012x3 .62x2 8.85x 85.6, where
• 1 lt x lt 30 and T is degrees Fahrenheit.
• Find and interpret the absolute extrema.
• When is the temperature growing at its fastest
• rate?

9
Strategy for solving optimization problems

• Read the problem carefully. Draw a picture if
  possible.
• Identify the unknown(s) and represent them with
  variables.
• Express the relationship between the unknowns
  what do you know?

10
Strategy, continued

• Determine the quantity which is to be optimized.
  Express it as a function using only one variable.
  Dont forget to state the domain of the
  function.
• Take the derivative and determine the desired
  extreme point(s).
• Verify that you have found what was asked for in
  the problem.

11
Example 1

• How can you divide a 250-foot long board
• into two pieces so that the product of the two
• lengths is a maximum?

12
Example 2

• Suppose that the perimeter of a rectangle is
  fixed at 20 square units. What are some possible
  dimensions for that rectangle?
• How do the different dimensions affect the area
  of the rectangle?

13
Example 3

• A farmer has 1500 feet of fencing with which
• to enclose a rectangular grazing area. What
• dimensions provide maximum grazing area?

14
Example 4

• You have 1500 feet of fencing to enclose a
• rectangular grazing area beside a stream,
• leaving the side next to the stream unfenced.
• Find the dimensions that provide for the
• maximum grazing area.

15
Example 5

• A rectangle has an area of 225 cm2. Find
• the dimensions that allow for the least
• perimeter.

16
Example 6

• A box is to be constructed with a square
• base and open top. The volume is to be
• 108 cubic inches. What dimension allow for
• the minimum amount of material?

17
Example 7

• You are fencing in a rectangular storage area
• next to a building. The area to be enclosed is
• 1280 ft2. The fence along the front costs 8
• per foot the other 2 sides use fencing costing
• 5 per foot. What dimensions minimize the
• cost? What is the minimum cost?