Exponents and Logarithms

1
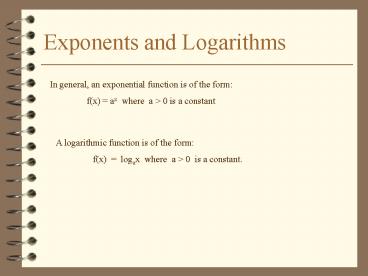
Exponents and Logarithms
In general, an exponential function is of the
form f(x) ax where a gt 0 is a constant
A logarithmic function is of the form f(x)
logax where a gt 0 is a constant.
2
Exponential Functions

• The graph of an exponential function f(x) ax
  where a gt 1 looks like

e.g. This is y 2x
All exponential functions pass through the point
(0,1) and are asymptotic to the x-axis.
3
Graphs of Exponential Functions
4
The yex Function
5
Exponential functions where 0 lt a lt 1

• Recall from earlier discussions how f(-x)
  differs from f(x).
• So, if f(x) 2x, then f(-x) 2-x
  (2-1)x or ( ½ )x whose graph looks like

6
Inverse Functions

• 1-1 Functions f(x) f(y) --gt x y
• horizontal line test

f(x) x3 2 Show this function to be 1-1 and
find its inverse.
The graph shows that the function is 1-1 since it
passes the horizontal line test.
7
Finding the Inverse Function

• 1. Write the function solved for y as a
  function of x.
• 2. Interchange the variables x and y.
• 3. Solve the new equation for y.
• 1. f(x) x3 2 --gt y x3 2
• 2. x y3 2
• 3. x - 2 y3 or y (x - 2)(1/3)

8
y x3 2 blue y (x - 2)(1/3) red
y x
9
Find the inverse for f(x)
y
x
x2 -1 - y y -x2 - 1 x ? 0
10

Find and graph the inverse function using
parametric equations.
Original function x(t) t
Inverse function y(t) t
11
The Graph
Original Function
Inverse Function
12
Logarithmic Functions

• Logarithms are inverse functions for exponential
  functions
• f(x) 2x f-1(x) log2x

13
Definition of a Logarithm

• loga x y iff ay x

Common Logs -- Base 10 Log 7 means log10
7 Natural Logs -- Base e ln 14.3 means
loge 14.3
14
Laws of Logarithms

• loga(x y) logax logay xgt0, ygt0
• log2(4 x 8) log24 log28 2 3 5
• loga(x/y) logax - logay
• log5(25/125) log525 - log5125 2 - 3 -1
• loga(xr) r logax where r is any Real no.
• log1021024 1024 log102 1024 x .3010
• 308.224

15
Examples

• log3 81

4
log 1000
3
6
log7 493
log2 ½
-1
ln e5
5
log5
1/3
log9 27
2/3
-3
log1/2 8
16
Example Given log 2 .3010, log 3
.4771, Find

• log 4
• log 8
• log 6
• log 9
• log 5
• log 12
• log 30
• log 3,000,000

• 0.6020
• 0.9030

17
Change of Base Formula
For example Find log795
18
Sketch the graph of y ln(x - 2) - 1
19
y ln(x - 2)
20
y ln(x - 2) - 1