Numerical integration

1
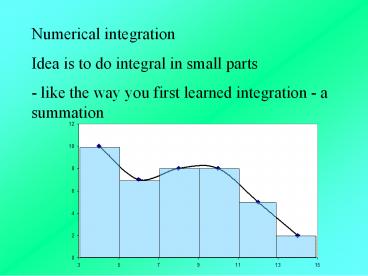
Numerical integration Idea is to do integral in
small parts - like the way you first learned
integration - a summation
2

• Numerical methods just try to make it faster and
  more accurate
• Newton-Cotes
• Simpson's rule
• Romberg integration, Gauss quadrature

3
Numerical integration Newton-Cotes formulas -
based on idea
where
4
can be linear
5
can be quadratic
6
Polynomial can be piecewise over the data
7

• Closed and open forms
• data points at the beginning and end limit of
  integration are known in closed
• open methods have integration limits that extend
  beyond the data
• open methods are akin to extrapolation
• generally not used with Newton-Cotes

8
Trapezoid rule - first of the Newton-Cotes
methods uses first order polynomial - straight
line
9
f(a)
f(b)
a
b
10
Trapezoid rule uses area of trapezoid to
approximate derivative
Error for Trapezoid rule
If function being integrated is linear - exact
(no second derivative)
11
Can apply trapezoid rule to multiple segments
over integration limits
Two segments
Three segments
Four segments
Many segments
12
Use n1 equally spaced points interval is Take
original trapezoidal rule
and break up limits of integration
13
Substituting trapezoid rule result for each
integral
14
Can be thought of as
width
Average height
Error can be estimated by
is the average second derivative
15
Example Numerically integrate
from a0.2 to b0.8
16
Single application of trapezoidal rule
We dont know zeta so approximate with average f
17
(No Transcript)
18
True value of integral is 12.82 Trapezoid rule
is 11.26 - within approx error - Etrue is 12
19
Multiple segment trapezoidal rule Use segments
(0.2,0.4),(0.4,0.6),(0.6,0.8)
20
Etrue is now 2
21
Use segments (0.2,0.3),(0.3,0,4), etc
22
Etrue is now 0.5