Complex numbers and function

1
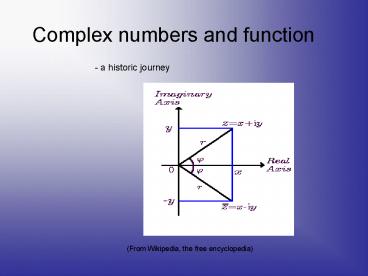
Complex numbers and function
- a historic journey
(From Wikipedia, the free encyclopedia)
2
Contents

• Complex numbers
• Diophantus
• Italian rennaissance mathematicians
• Rene Descartes
• Abraham de Moivre
• Leonhard Euler
• Caspar Wessel
• Jean-Robert Argand
• Carl Friedrich Gauss

3
Contents (cont.)

• Complex functions
• Augustin Louis Cauchy
• Georg F. B. Riemann
• Cauchy Riemann equation
• The use of complex numbers today
• Discussion???

4
Diophantus of Alexandria

• Circa 200/214 - circa 284/298
• An ancient Greek mathematician
• He lived in Alexandria
• Diophantine equations
• Diophantus was probably a Hellenized Babylonian.

5
Area and perimeter problems

• Collection of taxes
• Right angled triangle
• Perimeter 12 units
• Area 7 square units

?
6
Can you find such a triangle?

• The hypotenuse must be (after some calculations)
  29/6 units
• Then the other sides must have sum 43/6, and
  product like 14 square units.
• You cant find such numbers!!!!!

7
Italian rennaissance mathematicians

• They put the quadric equations into three groups
  (they didnt know the number 0)
• ax² b x c
• ax² b x c
• ax² c bx

8
Italian rennaissance mathematicians

• Del Ferro (1465 1526)
• Found sollutions to x³ bx c
• Antonio Fior
• Not that smart but ambitious
• Tartaglia (1499 - 1557)
• Re-discovered the method defeated Fior
• Gerolamo Cardano (1501 1576)
• Managed to solve all kinds of cubic equations
  equations of degree four.
• Ferrari
• Defeated Tartaglia in 1548

9
Cardanos formula

10
Rafael Bombelli
Made translations of Diophantus books
Calculated with negative numbers
Rules for addition, subtraction and
multiplication of complex numbers
11
A classical example using Cardanos formula
Lets try to put in the number 4 for x
64 60 4 0
We see that 4 has to be the root (the positive
root)
12
(Cont.)
Cardanos formula gives
Bombelli found that
WHY????
13
(Cont.)


14
(No Transcript)
15
Rene Descartes (1596 1650)

• Cartesian coordinate system
• a ib
• i is the imaginary unit
• i² -1

16
Abraham de Moivre (1667 - 1754)

• (cosx isinx)n cos(nx) isin(nx)
• zn 1
• Newton knew this formula in 1676
• Poor earned money playing chess

17
Leonhard Euler 1707 - 1783

• Swiss mathematician
• Collected works fills 75 volumes
• Completely blind the last 17 years of his life

18
Euler's formula in complex analysis
19
Caspar Wessel (1745 1818)

• The sixth of fourteen children
• Studied in Copenhagen for a law degree
• Caspar Wessel's elder brother, Johan Herman
  Wessel was a major name in Norwegian and Danish
  literature
• Related to Peter Wessel Tordenskiold

20
Wessels work as a surveyor

• Assistant to his brother Ole Christopher
• Employed by the Royal Danish Academy
• Innovator in finding new methods and techniques
• Continued study for his law degree
• Achieved it 15 years later
• Finished the triangulation of Denmark in 1796

21
Om directionens analytiske betegning

• On the analytic representation of direction
• Published in 1799
• First to be written by a non-member of the RDA
• Geometrical interpretation of complex numbers
• Re discovered by Juel in 1895 !!!!!
• Norwegian mathematicians (UiO) will rename the
  Argand diagram the Wessel diagram

22
Wessel diagram / plane
23
Om directionens analytiske betegning

• Vector addition

24
Om directionens analytiske betegning

• Vector multiplication
• An example

25
(Cont.)
The modulus is
The argument is
Then (by Wessels discovery)
26
Jean-Robert Argand (1768-1822)

• Non proffesional mathematician
• Published the idea of geometrical interpretation
  of complex numbers in 1806
• Complex numbers as a natural extension to
  negative numbers along the real line.

27
Carl Friedrich Gauss (1777-1855)

• Gauss had a profound influence in many fields of
  mathematics and science
• Ranked beside Euler, Newton and Archimedes as one
  of history's greatest mathematicians.

28
The fundamental theorem of algebra (1799)

• Every complex polynomial of degree n has exactly
  n roots (zeros), counted with multiplicity.

If
(where the coefficients a0, ..., an-1 can be real
or complex numbers), then there exist complex
numbers z1, ..., zn such that
29
Complex functions
30

• Gauss began the development of the theory of
  complex functions in the second decade of the
  19th century
• He defined the integral of a complex function
  between two points in the complex plane as an
  infinite sum of the values ø(x) dx, as x moves
  along a curve connecting the two points
• Today this is known as Cauchys integral theorem

31
Augustin Louis Cauchy (1789-1857)

• French mathematician
• an early pioneer of analysis
• gave several important theorems in complex
  analysis

32
Cauchy integral theorem

• Says that if two different paths connect the same
  two points, and a function is holomorphic
  everywhere "in between" the two paths, then the
  two path integrals of the function will be the
  same.
• A complex function is holomorphic if and only if
  it satisfies the Cauchy-Riemann equations.

33
The theorem is usually formulated for closed
paths as follows let U be an open subset of C
which is simply connected, let f U -gt C be a
holomorphic function, and let ? be a path in U
whose start point is equal to its endpoint. Then
34
Georg Friedrich Bernhard Riemann (1826-1866)

• German mathematician who made important
  contributions to analysis and differential
  geometry

35
Cauchy-Riemann equations
Let f(x iy) u iv
Then f is holomorphic if and only if u and v are
differentiable and their partial derivatives
satisfy the Cauchy-Riemann equations
and
36
The use of complex numbers today

• In physics
• Electronic
• Resistance
• Impedance
• Quantum Mechanics
• .

37
u
V