3.1 Lattice Disorder and Association of Defects

1
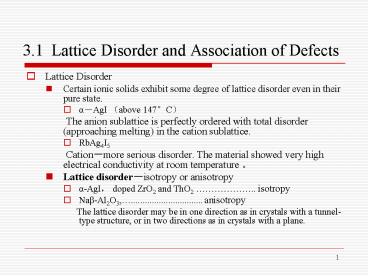
3.1 Lattice Disorder and Association of Defects

• Lattice Disorder
• Certain ionic solids exhibit some degree of
  lattice disorder even in their pure state.
• a-AgI (above 147C)
• The anion sublattice is perfectly ordered
  with total disorder (approaching melting) in the
  cation sublattice.
• RbAg4I5
• Cation-more serious disorder. The
  material showed very high electrical conductivity
  at room temperature ?
• Lattice disorder-isotropy or anisotropy
• a-AgI, doped ZrO2 and ThO2 .. isotropy
• Naß-Al2O3,...............................
  anisotropy
• The lattice disorder may be in one
  direction as in crystals with a tunnel-type
  structure, or in two directions as in crystals
  with a plane.

2
3.1 Lattice Disorder and Association of Defects

• Association of Defects
• Defects which caused by different valency-doped
• randomly
  distributed over the appropriate sublattice sites
  
• often carry a
  net charge
• e.g. ZrO2CaO, CaZr (Ca2 ion occupying a
  Zr4 ion site carries a net effective charge of
  -2 )
• VO(The oxygen ion
  vacancy has a net effective charge of 2)
• ZrO2YO1.5, YZr (A net effective
  charge of -1)
• VO(The oxygen ion
  vacancy has a net effective charge of 2)
• The formation of defect pairs or larger clusters
• Dynamic forceelectrostatic attraction
  between charged defects.
• Electric propertylarger clusters may be
  electrically neutral or carry a net charge.
• The concentration of free and quasifree defects
• Due to such association of defects, the
  concentration of free or quasifree defects does
  not increase linearly with increasing defect
  concentration and may decrease with decreasing
  temperature.

3
3.1 Lattice Disorder and Association of Defects

• The evaluation about the degree of association
  defects
• Lidiard has shown that, if the concentration of
  RX2-type impurity in MX-type compound is x, the
  degree of association ß which is defined in such
  a way that xß represents the mole fraction of
  complexes, is given by
• ß/(1-ß)2Oexp(G/kT)
• O-the number of distinct orientations of
  the complex
• G-the Gibbs free energy of association,
  i.e., the work gained under conditions of
  constant pressure and constant temperature in
  bringing a vacancy from a particular distant
  position to a particular nearest-neighbor
  position of the impurity ion
• T-absolute temperature
• k-Boltzmanns constant
• The ionic conductivities of the impure crystals
• s/s0(AB/A)/(1B)
  
• s,s0-the ionic conductivities of the impure and
  pure crystals
• Ax1/x0x0/x2, x1,x2-mole fractions of the two
  intrinsic defects in the dissociated
  state.x1x2x02
• B-ratio of the mobilities of defects 2 and 1

4
3.1 Lattice Disorder and Association of Defects

• At a given temperature, the conductivity varies
  with the impurity addition at first linearly(up
  to about 1 defect concentration) and then at a
  decreasing rate until it becomes nearly
  asymptotic.
• The impact of anion vacancy concentration on
  ionic conductivity in fluorite solid solution.

The above analysis appears to break down when
the defect concentration is beyond about 3-4,
since the conductivity then decreases with
increasing defect concentration. At these high
defect contents, clustering or ordering of
defects starts.
5
3.1 Lattice Disorder and Association of Defects

• A great deal of research work about the theory of
  association defects have been done
• Bakerthe number of anions taking part in cluster
  formation as a function of anion vacancy
  concentration in fluorite-type phases.
• OKeefehave studied about the nearest-neighbor
  interaction in a simple cubic lattice.
• Dipoles
• Positive and negative charges separated by a
  distance.
• Dipoles generated by charged defectsrespond to
  an alternating electrical field or mechanical
  stress and give rise to dielectric and mechanical
  loss, which is a function of frequency,
  temperature, and concentration.
• The behavior of larger complexes is expected to
  be different from that of dipoles.

Recent calculations based on near-neighbor
interactions show that the effective charge
carrier concentration goes through a maximum at a
certain dopant level in massively defective
solids. When very large defect contents are
involved,new compounds can occur.
6
3.2 Defect Equilibria

• The concentration of a thermally generated point
  defect
• It is a function of the energy of defect
  formation. Using standard statistical
  thermodynamic treatment, it can be shown that, in
  an ionic crystal MX-
• For Schottky defects
• ns/Nexp(-?Gs /2kT)
• For Frenckel defects
• nF/(NNi)1/2exp(-?GF /2kT)
• nS, nF-the numbers of Schottky and Frenckel pairs
• N-the total number of ions in the crystal
• Ni-the number of interstitial positions in the
  crystal
• ?GS-the energy required to form a Schottky pair
• ?GF - the energy required to form a Frenckel pair

7
3.2 Defect Equilibria

• Thermodynamically, each type of point defect is
  considered as an individual chemical species, and
  thus a defect equilibrium is represented by a
  form of chemical equation.
• Application of the law of mass action and the
  concept of equilibrium constant together with the
  electrical neutrality condition enable one to
  calculate the equilibrium concentration of each
  of the defects as functions of the partial
  pressures of the components.
• For simplicity, infinitely dilute solutions of
  defects are considered so that activities can be
  equated to concentrations.

8
3.2 Defect Equilibria

• For example pure ThO2 and Y2O3 doped ThO2
• At high oxygen pressures
• Pure ThO2 exhibits positive hole
  (electronic) conduction due to equilibrium
  between oxygen in the surrounding atmosphere and
  interstitial oxygen ions, Oi, in the lattice,
• ½ O2 Oi 2h
  
• K1 Oi p2 pO2-1/2
  p-the number of positive holes per unit
  volume
• The requirement of electrical neutrality
  in pure ThO2
• Oi ½ p, ? p? pO21/6
• At low oxygen pressures and high temperatures
• Oxygen vacancies are formed, which are
  electrically compensated by electrons dissociated
  from vacant oxygen sites,
• OO ½ O2 (g) VO 2e
• K2VO n2 pO21/2
  n-the number of excess electrons per unit volume
• if VO ½ n, n? pO2-1/6

9
3.2 Defect Equilibria

• At any oxygen pressure
• An equilibrium between interstitial
  oxygen ions and oxygen vacancies OO
  VO Oi
• K3 OiVO

10
3.2 Defect Equilibria

• When aliovalent impurities are added as in
  ThO2-Y2O3, the electrical neutrality requires
  that
• p 2VOn 2Oi YTh
• If the added Y2O3 dopant is more than a trace,
  YTh predominates on the right side of the
  above equation.
• Electrical neutrality is established by
  electronic defects pYTh
• Electrical neutrality is established by
  electronic defects 2VOYTh
• Of these two possible modes of compensation, the
  latter is confirmed by experiment.

11
3.2 Defect Equilibria

• Analysis similar to that used for pure ThO2 is
  applied to the case of Y2O3-doped ThO2
• At high oxygen pressures
• K1Oip2pO2-1/2
• OiVOK3
• For doping ThO2,VOconstant, ? Oiconstant
• ? p? pO21/4
• At extremely high oxygen pressures
• Y2O3 2YTh VO 3OO (2ZrO2)
• ½ O2 VO OO 2h
• Electrical neutrality condition
  YThh

12
3.2 Defect Equilibria

• At low oxygen pressures
• K2 VOn2pO21/2
• n?PO2-1/4
• These two terms cancel each other at intermediate
  oxygen pressures when the materials becomes
  predominantly an ionic conductor.
• The foregoing results can conveniently be
  summarized in a Kröger-Vink-type diagram.

13
3.2 Defect Equilibria
14
3.2 Defect Equilibria

• Point defects and temperature
• Point defects are in thermal equilibrium with the
  crystal, their concentrations are greatly
  influenced by temperature.
• The concentrations of intrinsic Schottky and
  Frenckel pairs
• Similar expressions may be obtained for a highly
  doped material like Y2O3-ThO2.
• (Ionic conduction region which is given
  above)
• The concentration of oxygen vacancies
• VO1/2 YThYT constant, so it is
  independent of temperature.
• However, according toK3 OiVO
• ? OiK3/ VOK30exp (-?G3/kT)/ ½ YTh
  const exp (-?G3/kT)
• ?G3 is the free energy change for the reaction(OO
  VO Oi).

15
3.2 Defect Equilibria

• The concentration of Oi increases with
  temperature until it becomes equal to VO.
• At that temperature the equilibrium condition
  changes and the material shows an intrinsic
  behavior.
• The concentrations in this range are given by
• Oi VO(K30)1/2 exp (-?G3/2kT)

16
3.3 Energy of Formation and Motion of Defects

• Energy of defects formation
• Born modelhas been used for the calculation of
  energies and motion of simple defects in alkali
  halides and the alkaline earth halides.
• Franklin has calculated the energies of formation
  for defects in CaF2.
• anion Frenckel 2.70.4 eV/pair
  cation Frenckel 7.11.0 eV/pair
• Schottky defects 5.80.8 eV/pair
• Obviously anion Frenkel pairs must be the
  dominant defects.
• This is consistent with Ures experimental value
  of 2.8eV for the formation of anion Frenkel pairs
  found from conductivity measurements
• Energy of motion of defects
• Born modelcan be used for calculating the energy
  of the migration of defect.
• The method consists mainly
  of calculating the energy of the defect at a
  saddle point position in an assumed path of the
  defect.
• The path which gives the smallest value of the
  saddle point energy is the most probable path for
  the migration of the defect.
• Chakravorty has found the values of activation
  energies for migration of anion vacancy and
  interstitial in CaF2.
• The values are 2.08 and 1.56eV for migration by
  interstitial and interstitialcy merchanism,
  respectively.
• The experimentally value1.55 eV