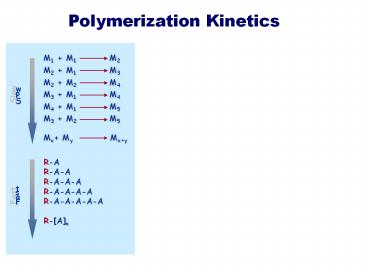
Polymerization Kinetics

1
Polymerization Kinetics
Slow
Mx My Mxy
R-A R-A-A R-A-A-A R-A-A-A-A R-A-A-A-A-A R-An
Fast
2
(No Transcript)
3
(No Transcript)
4
(No Transcript)
5
(No Transcript)
6
(No Transcript)
7
(No Transcript)
8
(No Transcript)
9
(No Transcript)
10
(No Transcript)
11
(No Transcript)
12
(No Transcript)
13
(No Transcript)
14
Chemical Kinetics
First things first. Do you recall anything that
you did in your studies of chemical kinetics? Do
the names vant Hoff and Arrhenius (the guys
shown on the left) mean anything to you? If they
dont, because studying physical chemistry gave
you brain lesions, or if you contracted some
dreaded, horrible disease that erased your memory
banks, then you should read our brief and
thoroughly incomplete review of kinetics. But, if
youre comfortable with the concept of rate
constants, press on!
15
The Reactivity of Chain Molecules
When Carothers was first attempting to synthesize
large molecules using condensation reactions,
focusing initially on polyesters, he ran into a
roadblock (Carothers and the history of nylon is
a great and ultimately tragic story that we cover
in one of our case studies click here if youre
already fed up with kinetics and want to go there
now). At first it seemed that a molecular weight
of about 6000 was the upper limit of what could
be obtained by these
reactions. At that time the consensus of opinion
was that the reactivity of the end group
decreased as the chain length got larger.
16
The Reactivity of Chain Molecules
It turns out that these esterifications were
reversible reactions and the polyesters were
being hydrolyzed back to acids and alcohols. But
before that was fully accepted, a young Paul
Flory made a bold and controversial assumption -
that the intrinsic reactivity of a functional
group is independent of the molecule to which it
is attached. He then proceeded to prove his point
with a detailed study of the kinetics of
polyesterification. So that seems like a good
point to start our studies of polymerization
kinetics.
This group reacts as readily as This group
HOOH
HOOH
17
The Kinetics of Step - Growth
Polymerization
Flory investigated polyesterifications of the
type
If youve already forgot that we use As and Bs
to represent the monomers reactive functional
groups, refresh your memory with the following
example
So here A-A is a diacid and B-B is a dialcohol
(diol). Ones first guess would be that this
bimolecular reaction would be second order with a
rate constant k2
18
The Kinetics of Step - Growth
Polymerization
But it isnt! Experimental measurements show
that the (overall) reaction is actually third
order (rate constant k3) because the reaction
is catalyzed by acids (so one of the reacting
components also acts as a catalyst). Hence
Note two important things. First, the rate of the
reaction is described in terms of the
disappearance of one of the functional groups, in
this case the As or acids. (Because As only
react with Bs and the stoichiometry is 11, we
could just as easily chosen the Bs to follow).
Second, the quantities A and B are the
concentrations of functional groups, not monomers
or molecules. In this reaction there are two
functional groups per monomer, so if some nasty,
sadistic prof. was to set you a homework
question where the concentration of monomers was
given, you would have to multiply these numbers
by two to get the concentration of functional
groups.
19
The Kinetics of Step - Growth
Polymerization
Now lets consider the important case where we
have exactly equal concentrations of functional
groups, so we can put
Instead of
we can write
And if the initial concentration (time, t 0) of
monomer is c0 then we can integrate this equation
to obtain
20
The Extent of Reaction, p.
If this reaction is indeed third order, and if
Florys assumption that the intrinsic reactivity
of a functional group is independent of chain
length is correct, then a plot of 1/c2 vs. t
should be linear. Because it provides a direct
link to the statistics of polymerization,
however, it is useful to first follow Flory and
define a new parameter, p, the extent of reaction
The fraction of unreacted groups must then, by
definition, be 1-p, so that c the concentration
of groups remaining at time t must be this
fraction times the initial concentration, c0.
This give the equation shown in the box, so now a
plot of 1/(1-p)2 vs. t should be linear.
21
The Kinetics of an Uncatalyzed
Polyesterification
And it is! Except right at the begining, in the
initial stages of the polymerization. The
curvature at low concentrations is typical of
simple (i.e. non polymer forming)
esterifications, however and can be attributed to
the large changes in character of the medium in
the initial stages of the reaction. Clearly, over
most of the reaction the plot is linear and the
reactivity of the groups would seem to be
independent of chain length. Note also how long
the reaction takes to reach high degree of
conversions. Well come back to this shortly.
400
20
300
0
100
0
200
100
0
Time (mins)
Redrawn from the data of Flory reported for the
reaction of adipic acid with diethylene glycol at
1660c.P.J.Flory ,J.A.C.S.,61,3334(1939)
22
Catalyzed Polyesterifications
Further evidence supporting Florys assumption
and analysis came from studies of the same
reaction catalyzed by the addition of small
amounts of a strong acid (p-toluene-sulfonic
acid). Because the amount of this acid catalyst
remains constant throughout the reaction its
concentration can be folded into the definition
of a rate constant, k k2Acid, hence
Again assuming equal amounts of reacting
functional groups
Integrating we now get
A plot of 1/(1-p) is linear (again except at low
degrees of conversion). Note that although the
reaction is speeded up considerably by the
catalyst, it still takes a
Redrawn from the data of Flory, P.J.,J.A.C.S.,61,3
334(1939)
long time to get to high degrees of conversion.
23
Conversion and Molecular Weight in Step-Growth
Polymerizations
So, is it important to carry the reaction to high
degree of conversions? For step-growth
polymerizations, absolutely! To understand why
just recall the definition of number average
degree of polymerization. This is simply the
number of monomer molecules you started with
divided by the number of molecules present at a
given moment in the polymerization (which in
principle could be determined by measuring the
number of end groups present)
If this immediately confuse you, just put in some
imaginary numbers lets pretend you started with
100 monomer units. After polymerizing for a time
t you stop the reaction and find you have 5
molecules (i.e. chains) present. Then the average
length of each chain is 100/20 5! Now lets
convert the number of molecules, actually moles,
to concentration by dividing N and N0 by the
volume V. We then get
24
Conversion and Molecular Weight in Step-Growth
Polymerizations
Accordingly, if
you dont get to decent degrees of
polymerization, lets say 200, unless you have
high degrees of conversion, for this example p
0.995, or a conversion of 99.5 ! And you want to
do this on an industrial scale! Organic chemist
friends of ours are happy to get reaction yields
of 80, which for our reaction would give us a
number average degree of polymerization of 5 not
good. The plot on the right demonstrates how you
only get decent degrees of
polymerization at high values of conversion.
Well revisit this when we talk about the
statistics of polymerization.