Optics I

1
Polarization
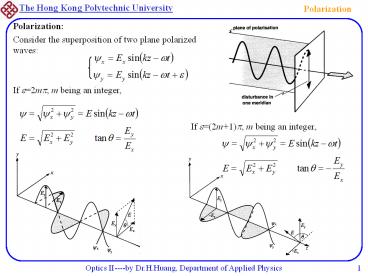
Polarization Consider the superposition of two
plane polarized waves If ?2m?, m being an
integer,
If ?(2m1)?, m being an integer,
2
Polarization
Right Circularly Polarized Light If ?(2m-1/2)?,
m being an integer,
Left Circularly Polarized Light If ?(2m1/2)?,
m being an integer,
Right and left circular light can be written
as, Their superposition becomes A plane
polarized wave can be synthesized from two
oppositely polarized circular waves.
3
Polarization
Elliptically Polarized Light Suppose
and ?(2m1/2)?, m being an integer.
State of Polarization P-state R-state and
L-state E-state Natural Light Natural light is
randomly polarized. We can mathematically
represent natural light in terms of two
arbitrary, incoherent, orthogonal, linearly
polarized waves of equal amplitude (i.e., waves
for which the relative phase difference varies
rapidly and randomly).
4
Polarization
Example A wave ? has the components
?xE1cos(kz-?t) and ?y-E1cos(kz-?t). What is
its state of polarization?
Polarizers a device to generate polarized light
out of natural one. It can also be used as an
analyzer to allow all E-vibrations parallel to
the transmission axis to pass. Malus Law When a
natural light passes though an ideal polarizer,
its intensity is reduced by half.
5
Polarization
Dichorism selective absorption of one of the two
orthogonal P-state in incident natural light.
wire-grid polarizer
dichroic crystal

• Commercial Polaroid H-Sheet
• Its a dichroic sheet polarizer.
• An ideal H-sheet would transmit 50 of the
  incident natural light and is designated HN-50.
• In practice, due to loss, the H-sheet might be
  labeled HN-46, HN-38, HN-32, and HN-22 with the
  number indicating the percentage of natural light
  transmitted through the H-sheet.

6
Polarization
Example Natural light of intensity Ii is
incident on three HN-32 sheets of Polaroid with
their transmission axes parallel. What is the
intensity of the emergent light?
Example Suppose the third Polaroid in the
last question is rotated through 45?. What is
now the intensity transmitted? Example Two
sheets of HN-38 Polaroid are held in contact in
the familiar crossed position. Let I1 be the
intensity emerging from sheet 1 natural light of
intensity Ii is incident upon it. The intensity
emerging from sheet 2 must be I20. Now insert a
third sheet of HN-32 between them with its
transmission axis at 45? to the other sheets
transmission axes. What is I2 now?
7
Polarization
Birefringence A material which displays two
different speeds of propagation in fixed and
orthogonal directions, and therefore displays two
refractive indices, is known as birefringent.
Distinction A dichroic material absorbs one of
the orthogonal P-states is dichroic while in
birefringent material we usually neglect the
absorption.
Rhombohedron of calcite. The optic axis passes
symmetrically through a blunt corner where the
three face angles equal 102?.
Atomic structure of a CaCO3 tetrahedron.
is called birefringence. uniaxial
positive uniaxial negative
8
Polarization
Double Refraction
A narrow beam of natural light incident normally
to a cleavage plane of a calcite crystal emerges
as two parallel beams displaced laterally.
Creation of an elliptical Huygens wavelet by
extraordinary ray. The material is uniaxial
negative. Ray direction S for the extraordinary
ray in birefringence material.
9
Polarization
Example A calcite plate is cut as shown in
figure with the optic axis perpendicular to the
plane of the paper. A ray of natural light,
?589.3 nm, is incident at 30? to the normal.
The plane of the paper is the plane of incidence.
Find the angle between the rays inside the
plate.
10
Polarization
Various Types of Plane Polarizers
Glan-Air prism
Nicol prism
11
Polarization
Example A 50? calcite prism is cut with its
optic axis as shown in the figure. Sodium light
is used in a spectrometer experiment to find no
and ne. Two images of the slit are seen and
minimum deviation is measured for each. Find no
and ne if the angles of minimum deviation are
27.83? and 38.99?. Explain how you would decide
which image was formed by the o-rays and which
was formed by the e-rays.
Since calcite is uniaxial negative,
therefore ne1.4864 and no1.6584
12
Polarization
Example A quartz Wollaston beam-splitting
polarizer is used with a normally incident
parallel beam of sodium light for which ne1.5534
and no1.5443. If the wedge angle is 45?, find
the angular separation of the emergent e- and
o-rays.
13
Polarization
Scattering The displacement of an electron
oscillating harmonically under an external field
is where ?0 is the natural frequency (resonant
frequency) of the oscillation of the bound
electron. At resonant frequency, strong
absorption occurs. At non-resonant frequencies
the absorption of the wave packet and its
subsequent emission is known as scattering.
Rayleigh Scattering Scattering centers have
dimensions smaller than the wavelength. The
radiated power is inversely proportional to the
fourth power of the wavelength of the incident
radiation. Polarization due to scattering
14
Polarization
Polarization by Reflection At Brewsters angle
ip, the reflected
light becomes plane polarized perpendicular to
the plane of incidence.
pile-of-plates polarizer
Brewster window
Degree of polarization
15
Polarization
Example The figure shows a ray of monochromatic
light incident at an angle ip on extra-dense
flint glass for which ng1.653. Show that the
angle between the transmitted and reflected rays
is generally equal to 90?, and find ip and i? in
this particular case.
Example Natural light is incident on a plane
air/glass boundary. Suppose the angle of
incidence is about 55? and the refractive index
of glass is 1.5. Calculate the degree of
polarization.
16
Polarization
Retarders Retarders are devices that cause one
orthogonal P-state component to lag behind the
other on emerging from the retarders. The path
difference is Full wave plate Half-wave
plate Quart-wave plate
The component E// and E? that travels faster
defines the fast axis of the plate.
17
Polarization
Compensator ?A device that allows a continuous
adjustment of the relative phase shift, the
retardance.
Soleil-Babinet compensator. (Left) Zero
retardation. (Right) Maximum retardation.
A circular light can be changed into P-state with
a quarter-wave plate. The handiness of circular
light can be checked by this method.
18
Polarization
Example A beam of left circular light meets a
quarter-wave plate with its fast axis vertical.
What is the state of the emergent light?

?x leads ?y by ?/2. On passing through
the wave plate, the ?y component travels faster
than the ?x one. It emerges with a phase advanced
by ?/4 and the two components are now in phase.
The resultant wave is a P-state, making an
angle ?45? with the x-axis.
19
Polarization
Example A quarter-wave plate made from calcite,
ne1.4864 and no1.6584, is placed in a beam of
normally incident light, ?656 nm, plane
polarized at 45? to the x-axis. Right circular
light emerges. Find the minimum thickness of the
plate and the orientation of the optic axis.
For minimum thickness, m0, therefore,
t953.49 nm. Example Show that the reflectance
R// is equal to the amplitude reflection
coefficient squared, r//2, and also show that
R?r?2. By definition
20
Polarization
Optical Activity The property that causes the
plane of polarization of P-state light to rotate
when it passes through certain material.
Viewing the beam head-on, if the rotation is
clockwise, the material is referred to as
dextrorotatory or d-rotatory. If the rotation is
anticlockwise, the material is referred to as
laevorotatory or l-rotatory.
Phenomenologically, the optical activity can be
explained by viewing that the linearly polarized
light as to be the superposition of equal amounts
of left- and right-circularly polarized
components through an optically active materials
with different velocities, vL and vR,
respectively.
21
Polarization
Some Applications Photoelasticity Kerr effect
Homework 12.1 12.3 12.4 12.6 12.7 12.10