Molecular Forces in Biological Media: - PowerPoint PPT Presentation
Title:
Molecular Forces in Biological Media:
Description:
Molecular Forces in Biological Media: Reference: Nossal, R. & Lecar, H. (1991): Molecular & Cell Biophysics Addison-Sesley Pub., Redwood City CA. – PowerPoint PPT presentation
Number of Views:36
Avg rating:3.0/5.0
Title: Molecular Forces in Biological Media:
1
- Molecular Forces in
Biological Media - Reference Nossal, R. Lecar, H. (1991)
Molecular Cell Biophysics - Addison-Sesley Pub., Redwood
City CA. - I. Forces within Macromolecules
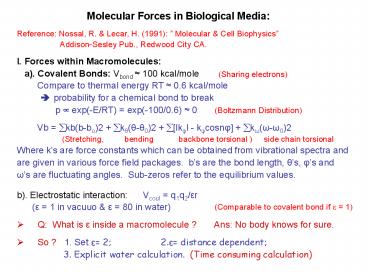
- a). Covalent Bonds Vbond ? 100 kcal/mole
- Compare to thermal energy RT ? 0.6
kcal/mole - ? probability for a chemical bond to
break - p ? exp(-E/RT) exp(-100/0.6) ? 0
- Vb ?kb(b-bo)2 ?k?(?-?0)2 ?lkfl -
kfcosnf ?k?(?-?0)2 - Where ks are force constants which can be
obtained from vibrational spectra and - are given in various force field packages. bs
are the bond length, ?s, fs and - ?s are fluctuating angles. Sub-zeros refer to
the equilibrium values. - b). Electrostatic interaction Vcoul
q1q2/er
(Sharing electrons)
(Boltzmann Distribution)
(Stretching, bending backbone
torsional ) side chain torsional
(Comparable to covalent bond if e 1)
2
c). Hydrogen bond
-
VH 0.5 4 kcal/mole - Comparable to RT (labile protons)
- When several H-bonds are formed the additive
- effect makes the structure quite stable.
- VH (A/raDA - B/r6DA)cosm(?D.H.A)cosn(?H.A.AA
) - The above equation showed that VH depends on
bond length and bond angles - between the donor and acceptor.
- d). Dispersive Forces (London dispersive
forces, van der Waals) - VVDW -a/r6
(Attractive force) - Lennard-Jones Potential V(r)LJ -(a/r6 b/rm)
rDA
A
H
D
?D.H.A
If m 12 VLJ(r) is also called 6-12 potential
Total Internal Energy V(r) Vb Vc Ve
VH VLJ
3
- II. Water as a Solvent
- Bound waters are found to strongly associate
with macromolecules, are - therefore affect the stability and
conformation of macromolecules strongly. - Hydrophobic interaction, the tendency of
nonpolar (aliphatic) groups to - aggregate in solution, is a major factor in
determining the conformation of - macromolecules.
- Charges groups preferred to be located on the
surface, whilst the hydrophobic - groups tends to be buried inside.
- How do solvent affect macromolecular structure
is still not fully understood.
III. Electrostatic Forces in Ionic Solution Q
How to calculate the electrostatic interaction
in an electrolytic solution? Solution The
Debye-Hückel Theory The electrostatic
potential at a point r, ?(r), is given by the
Poissons equation ?2?(r)
-4??(r)/? Where ?(r) is the charge density of
the electrolyte in the solution and ? is the
dielectric constant.
4
The charge distribution of a given mobile ion
is given by ni(r)
ni0exp-Wi(r)/kBT (Boltzmann Distribution) Where
Wi(r) is the potential energy of such an ion
arising from the charge on the stationary surface
acting in conjunction. kB is the Boltzmann
constant (1.38 x 10-23J/K) ni0 is the
concentration of i at Wi(r) 0. Thus, the total
charge density in the solution at r is given by
?(r) ?1(Zie)ni0exp-Wi(r)/kBT
(Poisson-Boltzmnn equation) Where Zi is the
valance of the ith species and e is the
elementary charge (1.6x10-19C). The potential
energy of ith ion can be written as Wi(r)
(Zie)?(r) Thus, ?2? -4??-1?1(Zie)ni0exp-(Zie
)?/kBT For e?/kBT ltlt 1 exp-(Zie)?/ kBT ?
1 - Zie?/kBT Thus, ?2? ?
-4??-1?1(Zie)ni01-Zie?/kBT ? ?2? The first
term vannishes due to charge neutrality and ? is
the inverse Debye length and is given by ?2
8?ne2/? kBT Debye length RDB (8?ne2/?
kBT)-1 where n 1/2?1ni0 Zi2 is the ionic
strength of the solution.
5
- Example 1 Under physiological condition, NaCl
140 mM 0.14M - n n(Na) n(Cl-)
(0.14)x12(0.14)x12/2 0.14 - (Z 1 for both Na and Cl-)
- and ?-1 8 Å (check it out)
- Example 2 Calculate the potential near a planer
surface (one dimensional) such as a linear lipid
bilayer. - d2?/dx2 ?2?, ? ? ?sexp(-?x)
- In 100 mM NaCl ?-1 8 Å. Thus, when x ?-1 8
Å , ? ?s/2.7 - The effect of ions in solution (counterions) is
to decrease the range of - the interaction between surface charge and
any charge in the medium. - Example 3 Potential between two charged sphere
of radius a. - ?(r) Z2e2?(1?a/2)2-1e-(r-a)/r
- Where r is the center-to-center distance between
the two particles. In comparison, ?(r) ? 1/r
between two unshielded charged particles.
6
Homework 1. (a)
Assuming that all other factors are equal, in
which ionic solution is the Debye length greater
0.12M CaCl2 or 0.2 M NaCl ? If the
Debye length in a 0.14 M NaCl is 8.2 Å , what is
the Debye length in a 0.2 M NaCl solution ?
(b) What would happen to the Debye screening if
one adds ethanol to an aqueous solution ? 2. (a)
The attractive can der Waals energy between two
neutral nonpolar small molecules, arising from
coupled charge fluctuations, varies as
-?/R6, where ? is the order of 10-58 erg-cm6 (in
vacuum, where the dielectric constant is
? 1). Estimate the energy of attraction
between two such molecules that are approximately
1 Å apart. At what separation does
energy become comparable with kBT, assuming T
293K ? What would be the effect on these
forces if the particles were immersed in a
medium with higher dielectric constants (e.g.,
that of water ?). (b) To first approximation,
the interaction between two identical large
particles can be calculated by pairwise
addition of the charge fluctuation forces
between individual atoms located in each of the
particles, that is W
? - N2 ?VAdra ?VBdrb ?/r6 , where
r is the separation between a mass point in
particle A and a mass point in particle B, N
represents the number of atoms in each
particle. If the particles have radius ra are
separted by a center-to-center distance
R, the interaction energy can be shown to be
W - (?2 ?N2/6)2a2/(R2
4a2) 2a2/R2 ln(1 4a2/R2) show
that when the spheres are very close so that R ?
2a, W ? (?2 ?N2)(a/R 2a)/12
Similarly, show that if R gtgt 2a , W ? (16?2
?N2)(a/R)6/9 Although the indicated
interaction seems to vary with the square of the
number of atoms when the spheres get
too big the intra atomic oscillation (induced
dipole) no longer in phase and these simple
expressions no longer valid.
7
3 The energy level of a quantized oscillator
are given by En (n ½)h?, where n 1, 2 .
And h is the Plancks constant. In
thermal equilibrium these levels are populated
according to the Boltzmann distribution
pn exp(-En/kBT)/?n
exp(-En/kBT) where kB is the Boltzmanns
constant and T is the absolute temperature. The
sum in the denominator is the partition
function, Z. (a) For an oscillator whose
energy spacing is 0.5 ev, determine which levels
are occupied by more than 10 of the
oscillator when T 300 K. Explain why the
quantity kBT is said to gauge the strength of
interaction. (b) The average internal
energy per molecule, U, is given by the sum U
?pnEn. Show that U is given by
U h?1/2 exp(h?/kBT) 1-1.
What is the average internal energy when h? is
much smaller than kBT ? (Hint
Evaluate U by noticing that ne-nx -d(e-nx)/dx
and use this relation to evaluate U from the
partition function Z, recall that
?n0? xn (1-x)-1).
8
(No Transcript)
9
(No Transcript)
10
(No Transcript)