Outline - PowerPoint PPT Presentation
1 / 15
Title:
Outline
Description:
Outline Correlation and Covariance Bivariate Correlation Coefficient Types of Correlation Correlation Coefficient Formula Correlation Coefficient Computation – PowerPoint PPT presentation
Number of Views:159
Avg rating:3.0/5.0
Title: Outline
1
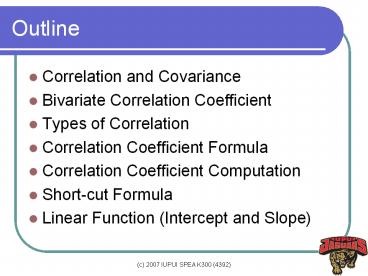
Outline
- Correlation and Covariance
- Bivariate Correlation Coefficient
- Types of Correlation
- Correlation Coefficient Formula
- Correlation Coefficient Computation
- Short-cut Formula
- Linear Function (Intercept and Slope)
2
Correlation and Covariance
- It asks how two variables are related
- When x changes, how does y change?
- Underlying information is covariance
- Cov(x,y)E(x-xbar)(y-ybar)
- Cov(x,y)Cov(y,x)
- Cov(x,x)Var(x), variance is a special type of
covariance (covariance of a variable and itself)
3
Bivariate Correlation Coefficient
- (Karl Pearson product moment) correlation
coefficient - Bivariate correlation coefficient (BCC) for two
interval/ratio variables - Differentiated from Spearmans rank correlation
coefficient (nonparametric) - Differentiated from partial correlation
coefficient that controls the impact of other
variables - No causal relationship imposed. X?Y or Y?X
- BCC is used for prediction
4
Bivariate Correlation Coefficient
- BCC ranges from -1 to 1 (So does Gamma ?)
- Covariance component can be negative
- means positive relationship when x increases 1
unit, y increases r unit - 0 means no relationship.
- - means negative relationship when x increases 1
unit, y decreases r unit. - http//noppa5.pc.helsinki.fi/koe/corr/cor7.html
5
Positive relationship
6
Negative relationship
7
No relationship
8
Correlation Coefficient
- Ratio of the covariance component of x and y to
the square root of variance components of x and y
9
Correlation Coefficient (short-cut)
Textbook suggests a short-cut formula below but
it is not recommended.
10
Illustration example 10-2, p.526
No x y (x-xbar) (y-ybar) (x-xbar)2 (y-ybar)2 (x-xbar)(y-ybar)
1 43 128 -14.5 -8.5 210.25 72.25 123.25
2 48 120 -9.5 -16.5 90.25 272.25 156.75
3 56 135 -1.5 -1.5 2.25 2.25 2.25
4 61 143 3.5 6.5 12.25 42.25 22.75
5 67 141 9.5 4.5 90.25 20.25 42.75
6 70 152 12.5 15.5 156.25 240.25 193.75
Sum 345 819 561.5 649.5 541.5
Mean 57.5 137
SSxx SSyy SPxy
Correlation coefficient Correlation coefficient Correlation coefficient Correlation coefficient 0.8967
11
Hypothesis Test
- How reliable is a correlation coefficient?
- r is a random variable drawn from the sample ?
is its corresponding parameter - H0 ? 0, Ha ? ? 0
- TS follows the t distribution with dfn-2
- If H0 is not rejected, r is not reliable
regardless of its magnitude (? 0)
12
Illustration Example 10-3, p.529
- Step 1. H0 ? 0, Ha ? ? 0
- Step 2. a.05, df4 (6-2), CV2.776
- Step 3. TS4.059, r.897
- Step 4. TSgtCV, reject H0 at the .05 level
- Step 5. ? ? 0
13
Linear function
- A function transforms input into output in its
own way - Ex ysquare_root(x). Whey you put x (input) into
the funciton square_root(), you will get y
(output). - Linear function consists of a intercept and
linear combinations of variables and their slops.
Y a bX cX2 - Slopes are constant
14
Intercept and Slope of a function
- A linear model Y a b X
- Dependent variable Y to be explained
- Independent variable X that explains Y
- Y-Intercept a the coordinate of the point at
which the line intersects Y axis. - Slope b the change of dependent variable Y per
unit change in independent variable X
15
Illustration







![[PDF] DOWNLOAD FREE Clinical Outline of Oral Pathology: Diagnosis and PowerPoint PPT Presentation](https://s3.amazonaws.com/images.powershow.com/10076578.th0.jpg?_=20240711025)


![[READ]⚡PDF✔ Black Letter Outline on Contracts (Black Letter Outlines) 5th Edition PowerPoint PPT Presentation](https://s3.amazonaws.com/images.powershow.com/10044064.th0.jpg?_=20240531080)



















