Rational Functions

1
Rational Functions
3.6 Rational Functions and Their Graphs
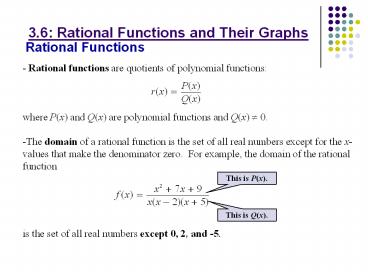
- Rational functions are quotients of polynomial
functions where P(x) and Q(x) are polynomial
functions and Q(x) ? 0. -The domain of a
rational function is the set of all real numbers
except for the x-values that make the denominator
zero. For example, the domain of the rational
function is the set of all real numbers
except 0, 2, and -5.
This is P(x).
This is Q(x).
2
Rational Functions
3.6 Rational Functions and Their Graphs
Graphs of rational functions have breaks in them
and can have distinct branches. We use a special
arrow notation to help describe this situation
symbolically
Arrow Notation Symbol Meaning x ? a x
approaches a from the right. x ? a - x
approaches a from the left. x ? ? x approaches
infinity that is, x increases without bound. x ?
- ? x approaches negative infinity that
is, x decreases without bound.
3
3.6 Rational Functions and Graphs

• Consider the graph of
• What happens as ?
• What happens as
• What happens as
• What happens as

4
Vertical Asymptotes of Rational Functions
3.6 Rational Functions and Their Graphs
Definition of a Vertical Asymptote The line x
a is a vertical asymptote of the graph of a
function f if f (x) increases or decreases
without bound as x approaches a. f (x) ?
? as x ? a f (x) ? ? as x ?
a -
Thus, f (x) ? ? as x approaches a from either
the left or the right.
5
Vertical Asymptotes of Rational Functions
3.6 Rational Functions and Their Graphs
Definition of a Vertical Asymptote The line x
a is a vertical asymptote of the graph of a
function f if f (x) increases or decreases
without bound as x approaches a.
f (x) ? - ? as x ? a f (x) ? -
? as x ? a -
Thus, f(x) ? - ? as x approaches a from either
the left or the right.
6
Vertical Asymptotes of Rational Functions
3.6 Rational Functions and Their Graphs
If the graph of a rational function has vertical
asymptotes, they can be located in the following
way
7
Horizontal Asymptotes of Rational Functions
3.6 Rational Functions and Their Graphs
A rational function may have several vertical
asymptotes, but it can have at most one
horizontal asymptote.
Definition of a Horizontal Asymptote The line y
b is a horizontal asymptote of the graph of a
function f if f (x) approaches b as x
increases or decreases without bound.
f (x) ? b as x ? ? f (x) ? b as
x ? ? f (x) ? b as x ? ?
8
Horizontal Asymptotes of Rational Functions
3.6 Rational Functions and Their Graphs
If the graph of a rational function has a
horizontal asymptote, it can be located in the
following way
9
3.6 Rational Functions and Their Graphs
Strategy for Graphing a Rational Function Suppose
that where P(x) and Q(x) are polynomial
functions with no common factors. 1. Factor
Factor numerator and denominator. 2.
Intercepts Find the y-intercept by evaluating f
(0). Find the x-intercepts by finding the
zeros of the numerator. 3. Asymptotes Find the
horizontal asymptote by using the rule for
determining the horizontal asymptote of a
rational function. Find the vertical
asymptote(s) by finding the zeros of the
denominator. 4. Behavior check the behavior on
each side of vertical asymptote(s).5. Sketch
graph. (Plot at least one point between and
beyond each x-intercept and vertical asymptote.)
10
EXAMPLE Finding the Slant Asymptote of a
Rational Function
3.6 Rational Functions and Their Graphs
11
EXAMPLE Finding the Slant Asymptote of a
Rational Function
3.6 Rational Functions and Their Graphs
12
Horizontal Asymptotes of Rational Functions
3.6 Rational Functions and Their Graphs
If the graph of a rational function has a
horizontal asymptote, it can be located in the
following way
Locating Horizontal Asymptotes Divide the
numerator and denominator by the highest power of
x that appears in denominator and then let