Chapter 4 Differential Relations For Viscous Flow

1
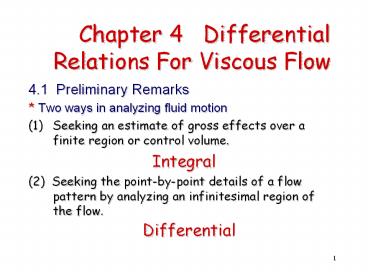
Chapter 4 Differential Relations For Viscous
Flow

• 4.1 Preliminary Remarks
• Two ways in analyzing fluid motion
• Seeking an estimate of gross effects over a
  finite region or control volume.
• Integral
• (2) Seeking the point-by-point details of a flow
  pattern by analyzing an infinitesimal region of
  the flow.
• Differential

2
Viscous flow Viscosity is inherent nature of
real fluid. Strain(??) is very strong in internal
flow.
Two forms of flow Turbulent(?,?) flow,
laminar(?)flow
Turbulent Flow VS. Laminar Flow
Transition
Reynolds tank
Reynolds number
???/???
3
4.2 The Acceleration Field of a Fluid
4
Substantial (Material) derivative ??(????)??
5
Example
Given . Find the
acceleration of a particle.
6
4.3 Differential Equation of Mass Conservation
X inlet (mass flow)
X outlet
X flow out
In the like manner

7
Loss of mass in the CV
8
For steady flow
For incompressible flow
9
Solution
Continuity for incompressible flow
Solution
10

• Assignment
• P264 P4.1(a), P4.2, P4.4 ,P4.9(a)

11
4.4 Differential Equation of Linear Momentum
Newtons second law
What are the surface forces Fs on the elemental
volume?
12
Surface force on an elemental volume
Vector Sum
Net Surface Force
13
It is not these stresses but their gradient,
which cause a net force on the differential
volume.
Momentum equation
In the like manner
14
Tensor ??
15
Constitutive Relation ??
Newtons Law (?????????)
16
Newton fluid, linear fluid (????,????)
Substitute Newtons Constitutive Relation into ME
17
N-S Equation
18
For incompressible flow
For inviscid flow
For 2-D,steady,incompressible flow
19
(No Transcript)
20
4.5 The Differential Equation of Energy
Infinitesimal fluid element
The first thermodynamic law
21
(1) Thermal conductivity (2) others
X Heat flow
According Fouriers Law
22
Body force
23
Surface force
dy
dz
X Net power
dx
Y,Z
24
Net power by Fs
left
25
(No Transcript)
26
???????,?s??h???p????????
27
?? Dû CvDT, Dh CpDT
28
Summary of the Equations
29
(No Transcript)
30
(No Transcript)
31
To Solve A Flow
equation unknown variables
continuity 1 r ,u,v,w
momentum 3 p, r u,v,w
energy 1 p, r u,v,w,T
perfect gas 1 p,r,T
32
4.6 Initial (??)and Boundary(??)Conditions for
the Basic Equations
Initial Conditions
Boundary Conditions
33
Solving the N-S equations numerically
Due to the highly complex of the N-S equations,
only a few particular solutions were found up to
now. For most problems, the equations must be
solved numerically, which is a brand new course
called CFD (Computational Fluid Dynamics) Flow
pass a cylinder An experiment result A
computation result
34
4.7 Exact solutions of N-S Equations
Flow between two parallel walls, Steady,
incompressible, neglect body force, 2-D
Continuity
Momentum
35
Integrate relative to y
Boundary condition
36
Apply the boundary condition
When U0
Poiseuille flow
Simple Couette flow
37
General case
38
Q0
39
The wall shear stresses
40
4.8 Dynamical Similarity Nondimensionalization
Measurement for Wing tip vortex
Flow pass a cylinder D 5cm D 10cm
41
4.8.1 Nondimensionalization of N-S Equation
N-S equation, 2-D, steady, no body force,
incompressible
Use U,L as reference velocity and length
Dimensionless quantities
42
Boundary conditions need to be normalized too
43
For steady, incompressible, no body force flow,
if two geometrically similar flow fields has same
Reynolds number, then they have similar flow
structure when same boundary conditions are
provided.
Why Reynolds number?
44
Inertia force / viscous force
?????????
45
4.8.2 Dynamical Similarity
Flow pass a cylinder D 5cm D 10cm
Flow pass a square Re 50 Re 10000
The flow fields for two objects of the same shape
but different size are said to be geometrically
similar. If,in addition,the Reynolds number are
the same,the two flows are said to be dynamically
similar,since the ratio of relevant forces are
the same in the two cases.
46
Low-Speed Large-Scale Compressor Facility
47
Chapter 5 Boundary Layer(BL)
Fluid-fluid Boundary
Fluid-solid Boundary
Fluid-gas Boundary
48
Chapter 5 Boundary Layer(BL)
The video shows the growth of a laminar boundary
layer over a bullet-shaped object, made visible
by the periodic introduction of lines or bubbles.
49
Chapter 5 Boundary Layer(BL)
The two animations show Lagrangian markers
following the flow over a plate for fluids with
high and low viscosities.
50
Chapter 5 Boundary Layer(BL)
How the viscous boundary layer develops close to
the surface as a result of the no-slip condition
at the wall?
51
Chapter 5 Boundary Layer(BL)
5.1 Three Thicknesses of a Boundary Layer
d
52
Chapter 5 Boundary Layer(BL)

• 5.1 Three Thicknesses of a Boundary Layer
• BL Thickness d (????,??)
• The locus of points where the velocity u
  parallel to the plate reaches 99 percent of the
  external velocity U.

53
(No Transcript)
54
(No Transcript)
55
The thickness of the viscous layer, d,can be
plotted as a function of time for fluids of
different viscosities. The viscous layer grows
with time and at a given time,it is larger for
the higher viscosity fluid.
56
Chapter 5 Boundary Layer(BL)
5.1 Three Thicknesses of a Boundary Layer 2.
Displacement Thickness (????)
Volume flux
Ideal flux
57
Chapter 5 Boundary Layer(BL)
5.1 Three Thicknesses of a Boundary Layer
3. Momentum Thickness
(????,??????)
58
Chapter 5 Boundary Layer(BL)
3. Momentum Thickness
(????,??????)
5.1 Three Thicknesses of a Boundary Layer
3. Momentum Thickness
(????,??????)
59
5.2 Momentum Integral Relation for Flat-plate BL
y
P
Free stream
U
Stream line
d
h
x
x
CV
Uconst Pconst
Steady incompressible
60
5.2 Momentum Integral Relation for Flat-plate BL
Outlet
Inlet
Continuity
61
Meantime
For flat plate boundary layer
62
(No Transcript)
63
(No Transcript)
64
Shape factor
65
Skin-friction coefficient
Drag coefficient
66
5.3 Boundary Layer Equation
Inviscid
67
5.3 Boundary Layer Equation
Outside the boundary layer where the gradients
are small,the viscous stresses are negligible
compared to the inertial forces and we can
approximate the flow as being inviscid. However,wi
thin the boundary layer,velocity gradients normal
to the wall are large,and thus the viscous
stresses are comparable to the inertial terms.
68
5.3 Boundary Layer Equation
69
5.3 Boundary Layer Equation
If we approximate the magnitudes of the
streamwise velocity by U,the vertical velocity by
V,the streamwise length scale by L,and the
vertical length scale by d,we can use these
estimates to simplify the continuity and momentum
equations.
70
5.3 Boundary Layer Equation
71
(No Transcript)
72
5.3 Boundary Layer Equation
The important feature here is the fact that the
pressure field in the boundary layer,and the
velocity at the edge of the boundary layer are
entirely determined by the inviscid solution
obtained for the outer flow.
73
5.3 Boundary Layer Equation
2-D,steady,incompressible,neglect body force

74
For BL,
External flow
(Inviscid Flow)
75
Euler Equation
Laminar flow
Turbulent flow
76
Supplementary Reynolds Equation
We can analyze the turbulent boundary layer by
recognizing that we can think of the velocities
and pressures as being comprises of an average
and fluctuating part. This representation was
first suggested by Reynolds and is called the
Reynolds decomposition.
77
Supplementary Reynolds Equation
78
Supplementary Reynolds Equation
79
Supplementary Reynolds Equation
80
Supplementary Reynolds Equation
81
Supplementary Reynolds Equation
82
Continuity(For incompressible flow)
83
(No Transcript)
84
Reynolds Equation
85
U(x)
U(x)
u
u
1
1
d
d
0
0
1
1
1
1

0
0
86
Blasius 1908
87
(No Transcript)