HEAT FLOW

1
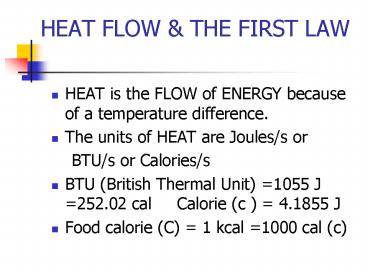
HEAT FLOW THE FIRST LAW

• HEAT is the FLOW of ENERGY because of a
  temperature difference.
• The units of HEAT are Joules/s or
• BTU/s or Calories/s
• BTU (British Thermal Unit) 1055 J
  252.02 cal Calorie (c ) 4.1855 J
• Food calorie (C) 1 kcal 1000 cal (c)

2
HEAT FLOW THE FIRST LAW

• The Calorie is defined as the amount of heat
  added 1 gram of water between 14.5 and 15.5 oC
  to raise it 1oC.
• A food calorie or kilocalorie is the amount of
  heat added to 1 kg of water (a liter) between
  16.5 and 17.5 oC to raise it one degree Celsius.

3
HEAT FLOW THE FIRST LAW

• The BTU is the amount of heat added to 1 lb of
  water (averaged over the temperature range of
  32oF to 212oF) one degree Fahrenheit.
• Both the BTU and calorie are non-metric units.
  The calorie will eventually be phased out in
  preference to the Joule.

4
HEAT FLOW THE FIRST LAW

• The heat flow must be made so that thermal
  equilibrium occurs at each stage of the process
  and an equation of state may be used. These
  changes must be done slowly (compared to
  propagation of molecules, the speed of sound)
• V 330 m/s in air at STP.

5
HEAT FLOW THE FIRST LAW

• The processes of heating must be reversible. (So
  the process can traverse the same path on a P-V
  diagram)
• There are irreversible processes which are not
  controlled and the system is not in thermal
  equilibrium, so these are not considered. These
  are not considered.

6
HEAT FLOW THE FIRST LAW

• In order to measure the heat flow through a
  substance one needs to know the HEAT CAPACITY C,
  so one writes
• dQ C dT
• where dQ is the small heat flow and dT is the
  small amount of temperature change.

7
HEAT FLOW THE FIRST LAW

• For finite changes one writes
• ?Q C ?T
• One can define
• specific heat capacity c ?Q/m?T for a one
  gram mass of the substance.
• Molar heat capacity c ?Q/n?T for one mole of
  the substance.

8
HEAT FLOW THE FIRST LAW

• Where C m c n c
• m mass and n number of moles
• C has units of cal/K
• c has units of cal/(g K)
• c has units of cal/mol K
• Also c Mc where M is molecular wt (g)
• (See Table 18-1 for values.)

9
HEAT FLOW THE FIRST LAW

• The heat capacity is C C(V,T)
• So C depends on how P,V,and T change
• dQ Cv dT (isochoric)
• dQ Cp dT (isobaric)
• (later well see that Cp Cv )
• The measurement of heat capacities is Adiabatic
  Calorimetry.

10
HEAT FLOW THE FIRST LAW

• Heat can also occur during phase changes because
  changes in molecular form either release or
  absorb energy. This occurs during melting or
  freezing or boiling or condensation or during
  sublimation or deposition. During these
  processes, the temperature does not change so
  they are called Latent heats.

11
HEAT FLOW THE FIRST LAW

• The Latent Heats are measured in three different
  units kJ/mol, kJ/kg, cal/g
• Your text has a table of Latent Heats, Melting
  and Boiling Points (18-2)
• Latent heats depend on p and T.
• Latent heat fusion heat vaporization
• (H2O) 79.7 cal/g 540 cal/g (1 atm)
• 333.5 kJ/kg 2257 kJ/kg (1 atm)

12
First Law of Thermodynamics Applied to the Human
Body
Chemical Energy
Work
Food
Thermal Energy
Heat

• Food enters the body containing chemical energy
• Some is converted to stored chemical energy and
  some to thermal energy
• Then chemical energy is converted to work
  (mechanical energy) and some heat is released
• Sometimes the body has to do work to replace heat
  lost (shivering)
• If the internal energy of the body is constant
  then food energy must equal heat loss and work
  done
• What happens if not?

13
Results of DU

• Changes in the internal energy result in changes
  in the measurable macroscopic variables of the
  system
• Pressure
• Temperature
• Volume
• For the human body it is usually temperature or
  volume (isobaric)

14
Metabolic Rates (Cal/m2-hr)
Your surface area can be approximated using
the formula SA .202m.425 x h.725 where m is in
kg and h is in meters. Calculate your surface area

• Sleeping 35
• Lying awake 40
• Sitting 50
• Standing 60
• Walking 140
• Running 600
• Shivering 250

The metabolic rate at rest is the basal metabolic
rate. The surface area of a 70 kg man of height
1.55m is about 1.70 m2. His metabolic rate is
therefore 40 x 1.70 68 Cal/hr while lying
awake.
15
Heat and Life

• We need energy to function (blood circulation,
  cell repair, etc.)
• Even at rest a 70 kg person consumes about 70
  Cal/hr
• The energy needed depends on a persons weight and
  build
• However, it has been found that human energy
  consumption (usage) divided by a persons surface
  area is approximately the same for most people
• It is given a unit of Cal/m2-hr and called
  metabolic rate

16
Measuring Metabolic Rate

• The metabolic rate is related to oxygen
  consumption by
• About 80 W is the basal metabolic rate, just to
  maintain and run different body organs

17
Various Metabolic Rates
18
Aerobic Fitness

• One way to measure a persons physical fitness is
  their maximum capacity to use or consume oxygen

19
Energy Output vs. Food Intake

• Food requirements depend on activity levels
• Consider this schedule
• Activity Energy (Cal/m2) For a
  person of SA 1.7m2 this is
  2320(1.70)
• 8 hr of sleep 280 or
  3940 Cal per day.
• 8 hr of moderate activity 1200 This
  could be met by a
• 4 hr of reading 240 diet of
• 1 hr of heavy exercise 300 400g of carbs
  1600 Cal
• 3 hr of dressing, eating 300 200g of
  Protein 800 Cal
• Total 2320 171g of fat 1540 Cal
• 3940 Cal

20
Weight Gain vs. Weight Loss

• They are stored as tissue (fat or muscle)
• Lack of caloric intake results in the body
  getting energy from stored fat first (9 Cal/g)
  and then proteins (4 Cal/g)
• The average person can go 50 days without food
• Angus Barbieri of Scotland consumed only tea,
  coffee and water from June 1965 to July 1966
  reducing his body weight from 472 lbs to 178 lbs
• Pregnant women need an extra 136 Cal/day which
  can come from an increased appetite or a decrease
  in physical activity
• The body cannot eliminate excess calories

21
Efficiency of the Human Body

• Efficiency is the ratio of the mechanical power
  supplied to the metabolic rate or total power
  input

22
Example Hiking Howards Knob

• Suppose one starts out from King Street
• and climbs Howards Knob. How much energy is
  needed?
• ?h1400 ft427 m Assume m80 kg
• PE mgh 80 kg 9.8 (m/s2) 427 m
• 335 kJ/4.186kj/kcal 80 kcal e.2
• ?PE(ME) e?PEfood ?PEfood 400 kcal

23
HEAT FLOW IN MATERIALS

• Heating occurs by Radiation, Convection and
  Conduction. In materials it is by conduction.
• ?Q/?t ? A ?T/L
• where is ? is the thermal conductivity.
• The unit of conductivity w/(mK) ,see text Table
  18-3 for ? values.

24
HEAT FLOW IN MATERIALS

• Materials which have poor thermal conductivity
  can be thought of as thermal resistors. R L/?
• the metric unit of R is m2 K /W
• in the English System R is ft2 oF hr/BTU
• R(value)1 ft2 oF hr/BTU 0.18 m2 K/W
• R value of 4 inch fiberglass 11

25
HEAT FLOW IN MATERIALS

• R value for 6 inch fiberglass is 19
• R value for 3/8 plasterboard is 0.32
• R value for glass is 0.02
• R value for plywood 0.6
• R value for brick is between 0.6 ? 1.0
• If A 1 ft2 , ?T (68-18) 50 oF, R11
• then, Q/t 4.6 BTU/hr 1.35 W.

26
HEAT FLOW IN MATERIALS

• In analogy with electrical phenomena
• one can apply the mathematical formalism of
  electrical phenomena to similar thermal
  phenomena.
• Electrical Thermal
• R R/A
• R in series R1 /A R2 /A RT /A

27
HEAT FLOW IN MATERIALS

• Electrical Thermal
• R in parallel A1 /R1 A2 /R2 AT /Reff
• Conductivity
• s ?
• ? (resistivity) 1/s 1/?
• ? V ? T
• i ?QCharge /?t ?QHeat/?t
• Flux i ? V/R ?QHeat/?t ?T A/R

28
HEAT FLOW IN MATERIALS

• Let Electrical Conductivity s 1/?
• The ratio of the thermal conductivity and the
  electrical conductivity and the Kelvin
  Temperature is the
• Widermann- Franz LorentzRatio
• s/?T 3 (R/F)2 2.23 x 10-8 (V/K)2
• Approximately constant for metals.

29
Work in a THERMAL SYSTEM

• Consider a piston of area A being pushed outward
  by a gas in a volume V
• The WORK DONE by the gas is
• dW F dx p A dx p dV
• W V1?V2 p dV
• Sign of work determined by V1 gt or lt V2